Marianne Freiberger Rachel Thomas - Numericon: A Journey Through the Hidden Lives of Numbers

New York London

2014 by Marianne Freiberger and Rachel Thomas

All rights reserved. No part of this book may be reproduced in any form or by any electronic or mechanical means, including information storage and retrieval systems, without permission in writing from the publisher, except by reviewers, who may quote brief passages in a review. Scanning, uploading, and electronic distribution of this book or the facilitation of the same without the permission of the publisher is prohibited.

Please purchase only authorized electronic editions, and do not participate in or encourage electronic piracy of copyrighted materials. Your support of the authors rights is appreciated.

Any member of educational institutions wishing to photocopy part or all of the work for classroom use or anthology should send inquiries to .

e-ISBN 978-1-62365-411-5

Distributed in the United States and Canada by
Hachette Book Group
1290 Avenue of the Americas
New York, NY 10104

www.quercus.com

Marianne Freiberger and Rachel Thomas are the Editors of Plus Magazine (www.plus.maths.org), a free online magazine opening a door to the world of maths for the general public.

Before joining Plus in 2005, Marianne did a PhD in pure mathematics, followed by three years as a postdoc at Queen Mary, University of London. She has also been Editor-in-Chief of the mathscareers website.

Rachel worked as a maths consultant for business, government and industry after completing her Masters in pure mathematics at the University of Western Australia. She has edited the Gazette of the Australian Maths Society and designed mathematical walking tours with Marcus du Sautoy for Maths in the City.

Rachel and Marianne were editors of the popular maths book 50: Visions of Mathematics (OUP, 2014).

Humanity has an urge to explore. We cant stop ourselves from wondering what is around the corner, over the next hill and beyond the horizon. This is as true for the great explorers as it is for any of us travelling somewhere for the first time. In the spirit of exploration, we would like to take you on a journey to an exciting land, one that is familiar to some but foreign to many. We will take you to some of our favourite places, show you the dramatic landscapes, beautiful views and precious treasures, and tell you stories of the valiant heroes, the baffling mysteries and the brilliant conquests. Were going on a guided tour through the world of mathematics.

Its a world that can seem hard to penetrate, but the forbidding symbols and equations are just another language: code for beautiful ideas that often find surprising uses in the ordinary world we all live in. We will help translate, taking you to some of mathematics most famous landmarks, as well as some secluded coves and exotic beaches weve discovered on our own travels. Our guides will be those friendly representatives of maths we all meet every day: numbers. Each number is an opportunity to stop off, enjoy the view and explore the local territory along paths weve found fun to travel down ourselves.

People love a place for many reasons the views, the weather, the people, the food, the culture there are also many things that draw people to mathematics. For many, maths is fundamentally beautiful; indeed many mathematicians wont be entirely satisfied with their work until it has an elegance, simplicity and grace. Others are drawn by its unreasonable effectiveness its power to explain the world we live in. Sometimes this happens long after a piece of mathematics was discovered and often its hidden from view. Maths is the language spoken by all the sciences, taking us to the frontiers of knowledge, from the workings of the Universe to the workings of our minds, which enabled us to dream it all up in the first place.

As editors of Plus magazine (plus.maths.org), an online magazine that aims to open the door onto the world of maths, weve had the privilege to explore mathematics widely and to meet some of the amazing (and sometimes eccentric) people that build it. Apart from visiting our favourite mathematical sights, we would also like to tell the stories of the people and cultures that have created them. Funny, bizarre, tragic and dramatic, these stories are worth telling all by themselves. And in the same way that you appreciate a great piece of architecture more when you learn who built it and why, these stories can also give great insight into the lofty mathematical structures well meet along the way.

This book is our opportunity to do what we love doing most: to show off the beauty of maths in all its glory and tell the stories that weave through it. Youll probably have heard of many of our destinations, but some may be new, and we might even reveal some surprises along the way. We hope you enjoy the ride

In the beginning, there was nothing. Well, actually, no. In the beginning there was always something. It might have been beans, successful hunts, or victories in battle, but, for millennia, people were using maths to describe things counting them, measuring them, dividing them up. A mathematical description of nothing, zero, was still a long way off.

Its most likely that early humans counted on their fingers in the same way that we all first learn to count. (Its handy having a set of counting sticks at the end of your arm, or, rather, in the fold of your animal skin.) One of the first pieces of evidence of our use of numbers is what are believed to be tally marks cut into a 20,000-year-old bone, known as the Ishango Bone, found in Zaire, Africa. A tally system is a very sensible way to keep track of accumulating quantities, whether youre keeping track of a score or you are a prisoner marking your days in prison on your cell wall. The way we keep control of a large number of tally marks today is still firmly connected to our early days of counting we group them into fives, like the five fingers on our hands. The first four are marked individually, the fifth as a line crossing the first four, making a complete set. It makes sense that our idea of an easily manageable set is the same as the count of the digits on our hand.

What we called these counting quantities, whether we even had words for them, is another question. There are still cultures today, including the Pirah and Munduruk from the Brazilian Amazon, who have a name for small numbers or quantities, but refer to anything larger simply as many.

But over the centuries almost all cultures developed names and symbolic representations for numbers, and a way to combine these to write any number they could possibly need. Inscriptions found in Egyptian tombs from over 5,000 years ago (3000 BC) show that the Egyptians were using beautiful hieroglyphs to represent numbers, such as coils of rope, lotus flowers and frogs, to represent 100, 1,000 and 100,000. These symbols would then be repeated, to build up the number they required, some numbers requiring a large collection of symbols to be represented.

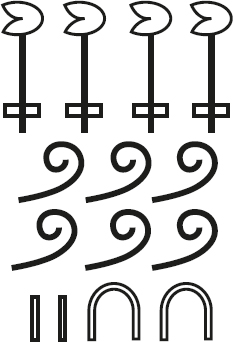
The number 4622 written in Egyptian numerals

The ancient Greeks built up numbers in a similar way, using letters from their alphabet to write numbers for example for 1, for 2, for 3, for 20, for 300. The Romans used combinations of symbols such as I for 1, V for 5, X for 10, L for 50, C for 100, D for 500 and M for 1,000 to write numbers. Generally Roman numbers were built up by adding together the symbols values, for example XII means 12 (though there were conventions for subtraction too, for example IV means 5 1 = 4). Its a system we still use today for names of kings and queens (King George VI) and for dates at the end of movies and TV shows.