APPENDIX 1
Simple Derivation of the Lorentz Transformation [Supplementary to section 11]
For the relative orientation of the co-ordinate systems indicated in , the x-axes of both systems permanently coincide. In the present case we can divide the problem into parts by considering first only events which are localised on the x-axis. Any such event is represented with respect to the co-ordinate system K by the abscissa x and the time t, and with respect to the system K by the abscissa x and the time t. We require to find x and t when x and t are given.
A light-signal, which is proceeding along the positive axis of x, is transmitted according to the equation
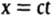
or

Since the same light-signal has to be transmitted relative to K with the velocity c, the propagation relative to the system K will be represented by the analogous formula

Those space-time points (events) which satisfy . Obviously this will be the case when the relation

is fulfilled in general, where indicates a constant; for, according to , the disappearance of (x ct) involves the disappearance of (x ct).
If we apply quite similar considerations to light rays which are being transmitted along the negative x-axis, we obtain the condition

By adding (or subtracting) equations , and introducing for convenience the constants a and b in place of the constants and , where
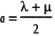
and

we obtain the equations

We should thus have the solution of our problem, if the constants a and b were known. These result from the following discussion.
For the origin of K we have permanently x = 0, and hence according to the first of the equations

If we call v the velocity with which the origin of K is moving relative to K, we then have

The same value v can be obtained from equations , if we calculate the velocity of another point of K relative to K, or the velocity (directed towards the negative x-axis) of a point of K with respect to K. In short, we can designate v as the relative velocity of the two systems.
Furthermore, the principle of relativity teaches us that, as judged from K, the length of a unit measuring-rod which is at rest with reference to K must be exactly the same as the length, as judged from K, of a unit measuring-rod which is at rest relative to K. In order to see how the points of the x-axis appear as
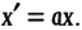
Two points of the x-axis which are separated by the distance x=1 when measured in the K system are thus separated in our instantaneous photograph by the distance

But if the snapshot be taken from K(t=0), and if we eliminate t from the equations , we obtain 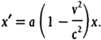 From this we conclude that two points on the x-axis separated by the distance l (relative to K) will be represented on our snapshot by the distance
From this we conclude that two points on the x-axis separated by the distance l (relative to K) will be represented on our snapshot by the distance

But from what has been said, the two snapshots must be identical; hence x in , so that we obtain

The equations , we obtain the first and the fourth of the equations given in section 11.
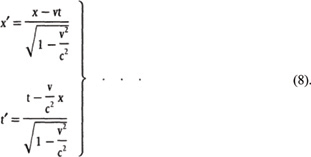
Thus we have obtained the Lorentz transformation for events on the x-axis. It satisfies the condition

The extension of this result, to include events which take place outside the x-axis, is obtained by retaining equations and supplementing them by the relations

In this way we satisfy the postulate of the constancy of the velocity of light in vacuo for rays of light of arbitrary direction, both for the system K and for the system K. This may be shown in the following manner.
We suppose a light-signal sent out from the origin of K at the time t=0. It will be propagated according to the equation
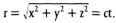
or, if we square this equation, according to the equation

It is required by the law of propagation of light, in conjunction with the postulate of relativity, that the transmission of the signal in question should take placeas judged from Kin accordance with the corresponding formula
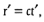
or,

In order that equation , we must have
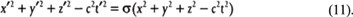
Since equation . We have thus derived the Lorentz transformation.
The Lorentz transformation represented by still requires to be generalised. Obviously it is immaterial whether the axes of K be chosen so that they are spatially parallel to those of K. It is also not essential that the velocity of translation of K with respect to K should be in the direction of the x-axis. A simple consideration shows that we are able to construct the Lorentz transformation in this general sense from two kinds of transformations, viz. from Lorentz transformations in the special sense and from purely spatial transformations, which corresponds to the replacement of the rectangular coordinate system by a new system with its axes pointing in other directions.













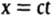




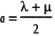




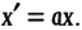

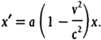 From this we conclude that two points on the x-axis separated by the distance l (relative to K) will be represented on our snapshot by the distance
From this we conclude that two points on the x-axis separated by the distance l (relative to K) will be represented on our snapshot by the distance