Günter Ludyk - Einstein in Matrix Form: Exact Derivation of the Theory of Special and General Relativity without Tensors

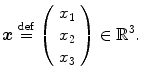
An event is anything that can happen in space and time, e.g. the emission of a flash of light in a room corner. Events happen at a single point. We assign to each event a set of four coordinates t , x 1, x 2 and x 3, or with t and the three-dimensional vector The position vector x and the time t form a reference frame . In this frame, Newtons fundamental law of mechanics has the form or, if the mass m in the momentum is constant, An observer may now execute any motion, for example, he makes an experiment in a moving train. We want to find the equation that takes the place of for the moving observer. 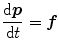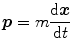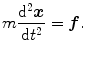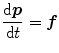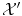A coordinate system is connected firmly with the moving observer; it should be axis-parallel to the original coordinate system 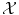. x o is the location of the origin of 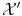measured in 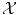(Fig. ). 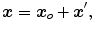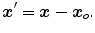Then 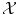or Here x is the position vector of the event measured by an observer at rest in the reference system , and x is what an observer measures in the moving reference system 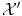. 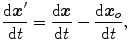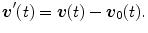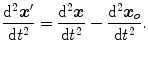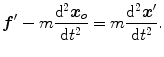Equation () differentiated with respect to time t , 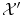results in the speed addition theorem of classical mechanics: For the acceleration one obtains The force f acting on the mass m is independent of the chosen coordinate system, so f = f . This and () result in The fundamental law of mechanics has lost its validity! If the moving observer knows the external force f , he can determine with measurements in his acceleration with respect to the rest system 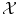. However, if the motion of 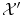with respect to 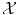is uniform and rectilinear, i.e. x o = v o t with a constant v o , then from ()