Christophe Bourlier - Shadowing Function from Randomly Rough Surfaces: Derivation and applications
Here you can read online Christophe Bourlier - Shadowing Function from Randomly Rough Surfaces: Derivation and applications full text of the book (entire story) in english for free. Download pdf and epub, get meaning, cover and reviews about this ebook. year: 2019, publisher: Scitech Publishing, genre: Home and family. Description of the work, (preface) as well as reviews are available. Best literature library LitArk.com created for fans of good reading and offers a wide selection of genres:
Romance novel
Science fiction
Adventure
Detective
Science
History
Home and family
Prose
Art
Politics
Computer
Non-fiction
Religion
Business
Children
Humor
Choose a favorite category and find really read worthwhile books. Enjoy immersion in the world of imagination, feel the emotions of the characters or learn something new for yourself, make an fascinating discovery.
- Book:Shadowing Function from Randomly Rough Surfaces: Derivation and applications
- Author:
- Publisher:Scitech Publishing
- Genre:
- Year:2019
- Rating:5 / 5
- Favourites:Add to favourites
- Your mark:
Shadowing Function from Randomly Rough Surfaces: Derivation and applications: summary, description and annotation
We offer to read an annotation, description, summary or preface (depends on what the author of the book "Shadowing Function from Randomly Rough Surfaces: Derivation and applications" wrote himself). If you haven't found the necessary information about the book — write in the comments, we will try to find it.
The shadowing function is an important element in the simulation and calculation of how electromagnetic waves scatter at randomly rough surfaces. Its derivation and use is an active, interdisciplinary area of research with practical applications in fields such as radar, optics, acoustics, geoscience, computer graphics and remote sensing.
This book addresses the general problem of the derivation of the shadowing function from randomly rough surfaces. The authors present an overview of theory and advances of this topic by detailing recent progress. Firstly, the simpler problems are investigated (monostatic case - 1D surface - one reflection) progressing in difficulty to more complicated problems (bistatic case - 2D surface - multiple reflections). In addition, the authors focus on the introduction of the simplifying assumptions to derive closed-form expressions of the shadowing function and quantify their impact on the accuracy of the resulting models. Applications of the shadowing function in problems encountered in physics are also addressed.
The problem of the derivation of the shadowing function from a rough surface is at the boundary between several scientific communities, each with its terminology. This book makes the link between these different communities and will help the reader to understand the theoretical aspects of this problem while giving practical applications.
Christophe Bourlier: author's other books
Who wrote Shadowing Function from Randomly Rough Surfaces: Derivation and applications? Find out the surname, the name of the author of the book and a list of all author's works by series.









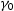 and on the height
and on the height 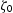 of any arbitrary point on the surface, which are random variables.
of any arbitrary point on the surface, which are random variables.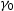 or/and
or/and 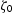 . This requires the knowledge of the joint PDF (probability density function) of
. This requires the knowledge of the joint PDF (probability density function) of 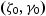 assumed to be uncorrelated.
assumed to be uncorrelated. and
and 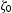 , Section 1.4 deals with the case of a correlated process assumed to be Gaussian. This Gaussian assumption leads to closed-form expressions of the average shadowing functions.
, Section 1.4 deals with the case of a correlated process assumed to be Gaussian. This Gaussian assumption leads to closed-form expressions of the average shadowing functions.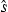 are blocked by the surface itself. This phenomenon is called the shadowing effect.
are blocked by the surface itself. This phenomenon is called the shadowing effect.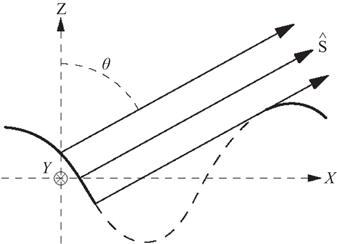
 with the zenith. The
with the zenith. The 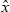 direction corresponds to the horizontal direction of the receiver. The dashed part of the surface lies in the shadow of the receiver, whereas the solid part of the surface is seen by the receiver
direction corresponds to the horizontal direction of the receiver. The dashed part of the surface lies in the shadow of the receiver, whereas the solid part of the surface is seen by the receiver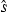 and the shape of the surface related to the roughness (heights, slopes, etc.). For instance, a flat surface is always fully illuminated in all observation directions
and the shape of the surface related to the roughness (heights, slopes, etc.). For instance, a flat surface is always fully illuminated in all observation directions  .
. , which means that the emission ray is propagating vertically upward, no emission ray is blocked (see (a)).
, which means that the emission ray is propagating vertically upward, no emission ray is blocked (see (a)).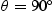 , which means that the emission ray is propagating horizontally towards the receiver located at the level of the horizon, nearly all emission rays are blocked, except for the few ones at the edge of the surface (see (b)).
, which means that the emission ray is propagating horizontally towards the receiver located at the level of the horizon, nearly all emission rays are blocked, except for the few ones at the edge of the surface (see (b)).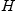 ). Indeed, if a surface point is in the shadow of the receiver, its emission ray along
). Indeed, if a surface point is in the shadow of the receiver, its emission ray along  reaches the surface at some other point.
reaches the surface at some other point. in the
in the 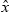 direction (corresponds to the horizontal direction of the receiver) being larger than the slope
direction (corresponds to the horizontal direction of the receiver) being larger than the slope 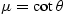 of the emission ray lie in the shadow of the receiver, as the local angle of incidence(d))
of the emission ray lie in the shadow of the receiver, as the local angle of incidence(d)) 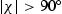 , which is not physical.
, which is not physical.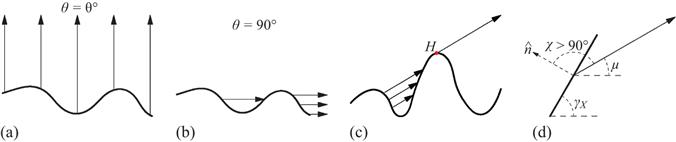
 . (b) Case = 90. (c) Shadowing versus the height of the surface point. (d) Shadowing versus the slope of the surface point
. (b) Case = 90. (c) Shadowing versus the height of the surface point. (d) Shadowing versus the slope of the surface point , shadowing is too significant to be neglected.
, shadowing is too significant to be neglected.