Contents
Guide
Page List


Copyright 2018 by McGraw-Hill Education. All rights reserved. Except as permitted under the United States Copyright Act of 1976, no part of this publication may be reproduced or distributed in any form or by any means, or stored in a data base or retrieval system, without the prior written permission of the publisher. ISBN: 978-1-26-002593-4
MHID: 1-26-002593-4 The material in this eBook also appears in the print version of this title: ISBN: 978-1-26-002592-7, MHID: 1-26-002592-6. eBook conversion by codeMantra
Version 1.0 All trademarks are trademarks of their respective owners. Rather than put a trademark symbol after every occurrence of a trademarked name, we use names in an editorial fashion only, and to the benefit of the trademark owner, with no intention of infringement of the trademark.
Where such designations appear in this book, they have been printed with initial caps. McGraw-Hill Education eBooks are available at special quantity discounts to use as premiums and sales promotions or for use in corporate training programs. To contact a representative, please visit the Contact Us page at www.mhprofessional.com. TERMS OF USE This is a copyrighted work and McGraw-Hill Education and its licensors reserve all rights in and to the work. Use of this work is subject to these terms. Except as permitted under the Copyright Act of 1976 and the right to store and retrieve one copy of the work, you may not decompile, disassemble, reverse engineer, reproduce, modify, create derivative works based upon, transmit, distribute, disseminate, sell, publish or sublicense the work or any part of it without McGraw-Hill Educations prior consent.
You may use the work for your own noncommercial and personal use; any other use of the work is strictly prohibited. Your right to use the work may be terminated if you fail to comply with these terms. THE WORK IS PROVIDED AS IS. McGRAW-HILL EDUCATION AND ITS LICENSORS MAKE NO GUARANTEES OR WARRANTIES AS TO THE ACCURACY, ADEQUACY OR COMPLETENESS OF OR RESULTS TO BE OBTAINED FROM USING THE WORK, INCLUDING ANY INFORMATION THAT CAN BE ACCESSED THROUGH THE WORK VIA HYPERLINK OR OTHERWISE, AND EXPRESSLY DISCLAIM ANY WARRANTY, EXPRESS OR IMPLIED, INCLUDING BUT NOT LIMITED TO IMPLIED WARRANTIES OF MERCHANTABILITY OR FITNESS FOR A PARTICULAR PURPOSE. McGraw-Hill Education and its licensors do not warrant or guarantee that the functions contained in the work will meet your requirements or that its operation will be uninterrupted or error free. Neither McGraw-Hill Education nor its licensors shall be liable to you or anyone else for any inaccuracy, error or omission, regardless of cause, in the work or for any damages resulting therefrom.
McGraw-Hill Education has no responsibility for the content of any information accessed through the work. Under no circumstances shall McGraw-Hill Education and/or its licensors be liable for any indirect, incidental, special, punitive, consequential or similar damages that result from the use of or inability to use the work, even if any of them has been advised of the possibility of such damages. This limitation of liability shall apply to any claim or cause whatsoever whether such claim or cause arises in contract, tort or otherwise.
Contents
Preface
Easy Algebra Step-by-Step is an interactive approach to learning basic algebra. It contains completely worked-out sample solutions that are explained in detailed, step-by-step instructions. Moreover, it features guiding principles, cautions against common errors, and offers other helpful advice as pop-ups in the margins.
The book takes you from number concepts to skills in algebraic manipulation and ends with word problems. Concepts are broken into basic components to provide ample practice of fundamental skills. The anxiety you may feel while trying to succeed in algebra is a real-life phenomenon. Many people experience such a high level of tension when faced with an algebra problem that they simply cannot perform to the best of their abilities. It is possible to overcome this difficulty by building your confidence in your ability to do algebra and by minimizing your fear of making mistakes. No matter how much it might seem to you that algebra is too hard to master, success will come.
Learning algebra requires lots of practice. Most important, it requires a true confidence in yourself and in the fact that, with practice and persistence, you will be able to say, I can do this! In addition to the many worked-out, step-by-step sample problems, this book presents a variety of exercises and levels of difficulty to provide reinforcement of algebraic concepts and skills. After working a set of exercises, use the worked-out solutions to check your understanding of the concepts. We sincerely hope Easy Algebra Step-by-Step will help you acquire greater competence and confidence in using algebra in your future endeavors.
The study of algebra requires that you know the specific names of numbers.
Natural Numbers, Whole Numbers, and Integers
The
natural numbers (or
counting numbers) are the numbers in the set
N = {1, 2, 3, 4, 5, 6, 7, 8, } The three dots indicate that the pattern continues without end.
Natural Numbers, Whole Numbers, and Integers
The
natural numbers (or
counting numbers) are the numbers in the set
N = {1, 2, 3, 4, 5, 6, 7, 8, } The three dots indicate that the pattern continues without end.
You can represent the natural numbers as equally spaced points on a number line, increasing endlessly in the direction of the arrow, as shown in . 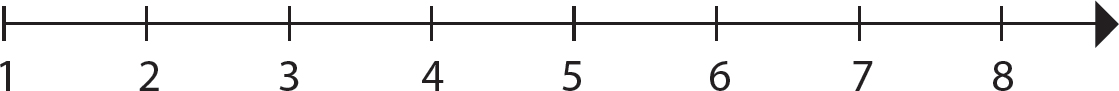 Figure 1.1 Natural numbers The sum of any two natural numbers is also a natural number. For example, 3 + 5 = 8. Similarly, the product of any two natural numbers is also a natural number. For example, 2 5 = 10. However, if you subtract or divide two natural numbers, your result is not always a natural number.
Figure 1.1 Natural numbers The sum of any two natural numbers is also a natural number. For example, 3 + 5 = 8. Similarly, the product of any two natural numbers is also a natural number. For example, 2 5 = 10. However, if you subtract or divide two natural numbers, your result is not always a natural number.
For instance, 8 5 = 3 is a natural number, but 5 8 is not. You do not get a natural number as the answer when you subtract a larger natural number from a smaller natural number. Likewise, 8 4 = 2 is a natural number, but 8 3 is not. You do not get a natural number as the quotient when you divide natural numbers that do not divide evenly. When you include the number 0 with the set of natural numbers, you have the set of whole numbers: W = {0, 1, 2, 3, 4, 5, 6, 7, 8, } The number 0 is a whole number, but not a natural number. If you add or multiply any two whole numbers, your result is always a whole number, but if you subtract or divide two whole numbers, you are not guaranteed to get a whole number as the answer.
Like the natural numbers, you can represent the whole numbers as equally spaced points on a number line, increasing endlessly in the direction of the arrow, as shown in .  Figure 1.2 Whole numbers The graph of a number is the point on the number line that corresponds to the number, and the number is the coordinate of the point. You graph a set of numbers by marking a large dot at each point corresponding to one of the numbers. The graph of the numbers 2, 3, and 7 is shown in .
Figure 1.2 Whole numbers The graph of a number is the point on the number line that corresponds to the number, and the number is the coordinate of the point. You graph a set of numbers by marking a large dot at each point corresponding to one of the numbers. The graph of the numbers 2, 3, and 7 is shown in . 












 Copyright 2018 by McGraw-Hill Education. All rights reserved. Except as permitted under the United States Copyright Act of 1976, no part of this publication may be reproduced or distributed in any form or by any means, or stored in a data base or retrieval system, without the prior written permission of the publisher. ISBN: 978-1-26-002593-4
Copyright 2018 by McGraw-Hill Education. All rights reserved. Except as permitted under the United States Copyright Act of 1976, no part of this publication may be reproduced or distributed in any form or by any means, or stored in a data base or retrieval system, without the prior written permission of the publisher. ISBN: 978-1-26-002593-4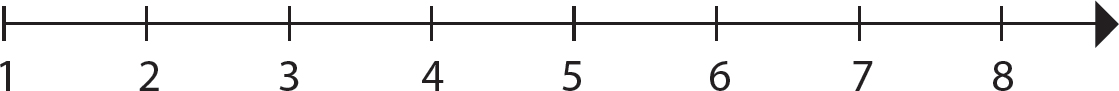 Figure 1.1 Natural numbers The sum of any two natural numbers is also a natural number. For example, 3 + 5 = 8. Similarly, the product of any two natural numbers is also a natural number. For example, 2 5 = 10. However, if you subtract or divide two natural numbers, your result is not always a natural number.
Figure 1.1 Natural numbers The sum of any two natural numbers is also a natural number. For example, 3 + 5 = 8. Similarly, the product of any two natural numbers is also a natural number. For example, 2 5 = 10. However, if you subtract or divide two natural numbers, your result is not always a natural number. Figure 1.2 Whole numbers The graph of a number is the point on the number line that corresponds to the number, and the number is the coordinate of the point. You graph a set of numbers by marking a large dot at each point corresponding to one of the numbers. The graph of the numbers 2, 3, and 7 is shown in .
Figure 1.2 Whole numbers The graph of a number is the point on the number line that corresponds to the number, and the number is the coordinate of the point. You graph a set of numbers by marking a large dot at each point corresponding to one of the numbers. The graph of the numbers 2, 3, and 7 is shown in . 