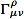Supergravity

Supergravity, together with string theory, is one of the most significant developments in theoretical physics. Although there are many books on string theory, this is the first-ever authoritative and systematic account of supergravity.
Written by two of the most respected workers in the field, it provides a solid introduction to the fundamentals of supergravity. The book starts by reviewing aspects of relativistic field theory in Minkowski spacetime. After introducing the relevant ingredients of differential geometry and gravity, some basic supergravity theories ( D = 4 and D = 11) and the main gauge theory tools are explained. The second half of the book is more advanced: complex geometry and N = 1 and N = 2 supergravity theories are covered. Classical solutions and a chapter on anti-de Sitter/conformal field theory (AdS/CFT) correspondence complete the text.
Numerous exercises and examples make it ideal for Ph.D. students, and with applications to model building, cosmology, and solutions of supergravity theories, this text is an invaluable resource for researchers. A website hosted by the authors, featuring solutions to some exercises and additional reading material, can be found at www.cambridge.org/supergravity.
Daniel Z. Freedman is Professor of Applied Mathematics and Physics at the Massachusetts Institute of Technology. He has made many research contributions to supersymmetry and supergravity: he was a co-discoverer of the first supergravity theory in 1976. This discovery has been recognized by the award of the Dirac Medal and Prize in 1993, and the Dannie Heineman Prize of the American Physical Society in 2006.
AntoineVan Proeyen is Head of the Theoretical Physics Section at the KU Leuven, Belgium. Since 1979, he has been involved in the construction of various supergravity theories, the resulting special geometries, and their applications to phenomenology and cosmology.

The metric is mostly plus, i.e. + +. The curvature is
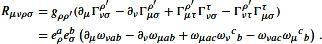
Ricci tensor and energymomentum tensors are defined by
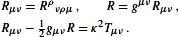
Covariant derivatives involving the spin connection are, for vectors and spinors,

We use (anti)symmetrization of indices with weight 1, i.e.

The Levi-Civita tensor is

The dual, self-dual, and anti-self-dual of antisymmetric tensors are defined by
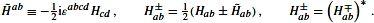
Structure constants are defined by
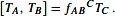
The Clifford algebra is
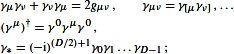
in four dimensions:
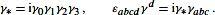
The Majorana and Dirac conjugates are

We mostly use the former. For Majorana fermions the two are equal. The main SUSY commutator is
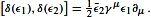
p -form components are defined by
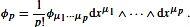
The differential acts from the left:
Supergravity

DANIEL Z. FREEDMAN
Massachusetts Institute of Technology, USA
and
ANTOINEVAN PROEYEN
KU Leuven, Belgium
CAMBRIDGE UNIVERSITY PRESS
Cambridge, New York, Melbourne, Madrid, Cape Town,
Singapore, So Paulo, Delhi, Mexico City Cambridge University Press
The Edinburgh Building, Cambridge CB2 8RU, UK Published in the United States of America by Cambridge University Press, New York www.cambridge.org
Information on this title: www.cambridge.org/9780521194013 D. Z. Freedman and A. Van Proeyen 2012 This publication is in copyright. Subject to statutory exception and to the provisions of relevant collective licensing agreements, no reproduction of any part may take place without the written permission of Cambridge University Press. First published 2012 Printed in the United Kingdom at the University Press, Cambridge A catalog record for this publication is available from the British Library Library of Congress Cataloging in Publication data
Freedman, Daniel Z.
Supergravity / Daniel Z. Freedman and Antoine Van Proeyen.
p. cm.
ISBN 978-0-52119401-3 (hardback)
1. Supergravity. I. Van Proeyen, Antoine. II. Title.
QC174.17.S9F735 2012
530.1423-dc23
2011053360 ISBN 978-0-52119401-3 Hardback Additional resources for this publication at www.cambridge.org/supergravity Cambridge University Press has no responsibility for the persistence or accuracy of URLs for external or third-party internet websites referred to in this publication, and does not guarantee that any content on such websites is, or will remain, accurate or appropriate.

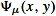
symmetry

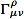

The main purpose of this book is to explore the structure of supergravity theories at the classical level. Where appropriate we take a general D -dimensional viewpoint, usually with special emphasis on D = 4. Readers can consult the Contents for a detailed list of the topics treated, so we limit ourselves here to a few comments to guide readers. We have tried to organize the material so that readers of varying educational backgrounds can begin to read at a point appropriate to their background. in considerable detail. It should be possible to understand these chapters without full study of earlier parts of the book.
Next page















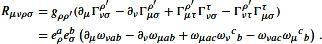
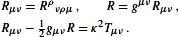



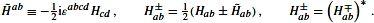
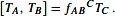
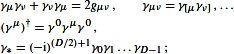
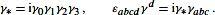

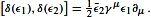
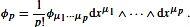
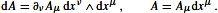


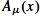
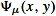 symmetry
symmetry