Fuzzy logic is used in many applications from industrial process control to automotive applications, including consumers trend forecast, aircraft maneuvering control and others. Considering the increased interest in using of multi-rotor aircrafts (usually called drones) for many kinds of applications, it is important to study new methods to improve multi-rotor maneuverability while controlling its stability in a proper way. Controlling the flight of multi-rotors, specially those equipped six rotors, is not a trivial task. When considering the design of such a control systems, traditional approaches such as PD/PID are very difficult to design, in spite of being easily implementable. This work proposes an approach based on multiple interconnected fuzzy controllers, aiming to control the various aspects related to maneuverability of a hexacopter carrying a free payload forming a pendulum. The behavior produced by such a control system has been simulated on a well-known robotics simulation environment and analyzed in terms of flight stability, as well as roll, pitch and yaw movements. The results show the feasibility of the proposed approach in keeping the hexacopter flying in a stable way.
1.1 Introduction
Nowadays, technology advances and cost reductions have popularized the use of small electromechanical aircrafts in many distinct application fields, such as video recording, plantation inspections, search-and-rescue assistance, military and civil surveillance applications, among others. Multi-rotor helicopters (also known as drones) are among the popular small electromechanical aircrafts that are being used in such applications. Moreover, some of these new applications demand multi-rotor helicopters that fly autonomously, as presented in []. Thus, the multi-rotor helicopter must have additional computational systems on top of the more basic movement and stabilization control systems. These computational systems provide higher level capabilities to support the mission accomplishment. Therefore, Unmanned Aerial Vehicles (UAV) are the preferred choice for these applications, due to the cost reductions obtained from eliminating the need of high-skilled and trained pilots.
There are several topologies for multi-rotor helicopter, varying on the number of rotors (i.e. motor and propeller), as well as on the position of these rotors onto the aircraft frame. The most common multi-rotor helicopter has 4 rotors and is called quadcopter. However, recently, other multi-rotor helicopter topologies are becoming popular, such as those with 6 rotors, the so-called hexacopter, as discussed in [].
The UAV stabilization is commonly performed by hybrid control approaches (parallel, cascade) with multiple PID controllers, like works of [].
Adaptive algorithms can be applied to establish multivariable systems (like UAVs) with more efficiency which classical strategies. In [] a fuzzy logic method is used to position control of a hexacopter. However, the main focus of previous works is the UAV stabilization over linear disturbances and is not evaluate the proposed control strategies over nonlinear disturbances, like a variable payload.
This work focuses on the control system for the movement and stabilization of a Hexacopter, whose rotors have been configured as a Hexa
topology. A multi-layer controller has been proposed and integrates multiple fuzzy controllers. The outputs from these fuzzy controllers must be applied on each rotor accordingly, in order to get the correct Hexacopter movements. A closed control loop is obtained by reading of sensors that measure the position and the movements of the Hexacopter, which, on the other hand, are used as feedback information to the proposed multi-layer controller. The main goal is to create a robust and flexible controller that is able to keep the Hexacopter stability when moving or hovering, even when it carries a free or loose payload that changes its center of gravity.
The major challenge tackled in this work is the switching among fuzzy controllers at the right moment. For instance, lets assume that a hexacopter starts on the ground and receives a command to fly to a certain position, e.g. 2 m in latitude, 5 m in longitude and 3 m upward. To achieve the commanded position, every movement must be executed properly, and hence, all controllers must work cooperatively to achieve the goal. In other words, the fuzzy controller that controls the longitudinal position cannot override the other controllers actions. On the other hand, when the fuzzy controllers do not work well together, a controller outputs can take the rotors actuation over the outputs of the other controllers. In this case, there must exist a high-level controller that performs the contention of misbehaved controllers.
The proposed approach has been validated through simulation. For that, a model of a hexacopter has been created in the V-REP simulation environment. A free payload has been attached to the hexacopter forming a pendulum. Thus, the proposed multi-layer controller must control the hexacopter movement when it is commanded to move to another position, while keeping the its body stabilized during the flight. Results indicate that the proposed approach is robust since it allows the hexacopter move from one position to another, even though it must carry a moving payload.
The reminder of this chapter is organized as follows: Sect. draws some conclusions and presents future work directions.
1.2 Description of the Controlled Plant: The Hexacopter
This section describes briefly the system under control, i.e. the hexacopter, as a control plant . Figure
By activating the rotors accordingly, it is possible to control the hexacopter maneuvering through the X, Y and Z axes.
In general, each plant must be analyzed to discover the interaction of each force. In this case, the thrust force produced by speeding up or slowing down some rotors leads the hexacopter toward the desired direction (on each axis), i.e. the vectors of the forces acting on the plant.
Fig. 1.1
The plant to be controlled: (i) X axis angle is the Roll rotation, (ii) the Y axis is the Pitch rotation, and (iii) the Z axis is the Yaw rotation. The arrow direction means positive
To understand the movements performed by the hexacopter it is worth to take a look at the forces acting in frame.
In the Figs. ) if the rear rotor has a value greater than the front rotor. If the front rotor has a value greater than the rear rotor, the hexacopter moves backward or, if it is going forward in this situation, this rotation makes it to slows down.












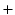 topology. A multi-layer controller has been proposed and integrates multiple fuzzy controllers. The outputs from these fuzzy controllers must be applied on each rotor accordingly, in order to get the correct Hexacopter movements. A closed control loop is obtained by reading of sensors that measure the position and the movements of the Hexacopter, which, on the other hand, are used as feedback information to the proposed multi-layer controller. The main goal is to create a robust and flexible controller that is able to keep the Hexacopter stability when moving or hovering, even when it carries a free or loose payload that changes its center of gravity.
topology. A multi-layer controller has been proposed and integrates multiple fuzzy controllers. The outputs from these fuzzy controllers must be applied on each rotor accordingly, in order to get the correct Hexacopter movements. A closed control loop is obtained by reading of sensors that measure the position and the movements of the Hexacopter, which, on the other hand, are used as feedback information to the proposed multi-layer controller. The main goal is to create a robust and flexible controller that is able to keep the Hexacopter stability when moving or hovering, even when it carries a free or loose payload that changes its center of gravity.