Šiljak - Murder on the Einstein Express and Other Stories

International Burch University Sarajevo, EEE Department, Sarajevo, Bosnia and Herzegovina

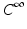At the moment of t =0, we arrived in the space B . Our group (denoted G ) was small, but commutative with respect to addition, composed of different non-negative elements. The trajectory that lead us to this space was class , smooth and continuous. The mission for the group remained unknown, although given implicitly.

It is the time to introduce myself. I am the supremum of the set of our group members, its maximum, actually since the set is closed. My name in the epsilon-delta language was The one equal to his derivative. You may call me e x . Strictly increasing, monotone, exponential. According to the DAlemberts test, I was the best choice for this mission, the only one to fulfill the Lipschitz condition on the hyperrectangle given in the well-protected basis of vector space V . I had the right to select a countable number of elements in my set, which I did, via a bijection with the set of integers to the group. I chose the members wisely, by the Cauchys test its strongest form.

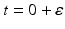While the days uniformly converged to the day of our departure, periodic function of my heart started shrinking its period, excitement grew factorial-wise. But the mission still remained a secret, multiplied with Heaviside function, available to us only at for an arbitrary small 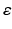, of course.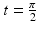

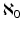I had to split with my family, the e x + C . They kept our famous dignity while saying farewell. Integrable (Riemann sense) even when its hardest, one could say.

While t 0, the Dirichlet kernel started its action on me. I didnt know what transform is it going to perform, I just knew I might easily be not equivalent to what I once was. Shall I ever return to our Euclidean Space? Shall an inverse transform ever make me the same I am today? What awaits in space B ?

I moved just an epsilon from the Origin, making insecure steps through the Space B . I was still the same exponential function, the transform did nothing to me. Thank Supremum for that!

I was afraid this was one of those spaces based on a set with cardinality . At one point in those spaces youre safe, but the next step can lead you to disappearance that is why I preferred the spaces with the power of continuum: better uncountable than incomplete, as my father would say. Luckily, this space, as wild as it looked like, didnt cause problems for my movement: I felt like moving in the [0,1] segment, and not in a strange, distant space. I examined the sinusoids of mountains, diverging in the infinity and thinking about the plane I grew up in. Alas, there wasnt time to think. Our mission has been officially stated: we are looking for an inverse Melin Transform of one of the functions from our Euclidean Space, which disappeared recently. Periodic functions have that habit disappearing and coming back. This function, as the members of her class informed us, isnt periodic at all.

It hasnt been clear how are we going to do our task, especially knowing that there are a lot of members of this B -Space that dont like us being here. Ever since t =0, I noticed that in an arbitrary small neighborhood of every one of us there had been countably infinite number of asymptotic followers: simply put, spies. Who sent them?

I knew that the ruler of this world is the infamous Dirichlet Function ( x ) (usually called just Function). Discontinuous at every point, graphically inexpressible, periodic without a period Fascinating and frightening properties. Not many functions in our Euclidean Space lived their lives without knowing of this Functions existence (and uniqueness), and her everyday wrongdoings in the B -Space. Still, there was nothing we could do, but hope that the vector of life wont bring us to her domain.

As they say, life is a convolution of luck and its inverse and I got majorised by the inverse. Obviously.

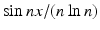In one of the epsilon neighborhoods of the Origin we met a series with a curious general term . He introduced himself as a Fourier series of one function not known well in our Space and he offered services as a guide through trajectories of B -Space. Other members of the group didnt find this series strange, but I definitely didnt like his story. True, it was a trigonometric series, even uniformly convergent (Im sure younger female members of my group liked that a lot, together with that modern stylish graph he had). Nevertheless, using the Parseval theorem (in V space we were reminded about it just before leaving), I have easily proven what I conjectured.

He is not a Fourier series.

Ignoring that for a moment, I let him guide us, with sole desire to see what is the Function up to. She obviously knew we are here.

The way which the alleged Fourier series chose looked more and more like a logarithmic spiral. We were moving, but I didnt see us approaching our goal, the Accumulation Point of B -Space (to be more precise, my goal was the Kernel of B -Space). Flocks of sinusoids flew over our level surface, disturbing the flux of my thoughts. I was thinking about danger that might be ahead in the trajectory given. My knowledge of weapons used here was fairly limited, because there werent many survivors after their use. Weapons I was warned about were the Differentiator and Riemann Integrator. Principle of their action was pretty simple: making derivatives and definite integrals out of the functions aimed at, respectively. Integrator sounded deadly and it was, because turning to a constant means death for functions from most spaces (luckily, there was only one Riemann Integrator in whole B -Space, held deep in its kernel, where only the Function and the most loyal Metric Guards have access to). There were stories of mass integrations and differentiations in integration and differentiation camps, respectively. Although this action has been forbidden by the Abel-Laplace theorems, there is already evidence of such procedures being conducted.

Beside these dangerous operators, functions in this space use multiplicators and additors in everyday life: operators for multiplication and addition with a non-zero real constant, respectively. Such actions are considered harmless. Such behavior was even encouraged by the Function herself!