Geometric Algebra
Emil Artin
Dover Publications, Inc.
Mineola, New York
Bibliographical Note
This Dover edition, first published in 2016, is an unabridged republication of the work originally published as No. 3 in the Interscience Tracts in Pure and Applied Mathematics series by Interscience Publishers, Inc., New York, in 1957.
Library of Congress Cataloging-in-Publication Data
Artin, Emil, 18981962.
Geometric algebra / Emil Artin.Dover edition.
p. cm.
Originally published: New York : Interscience Publishers, Inc., 1957.
Includes bibliographical references and index.
eISBN-13: 978-0-486-80920-5
1. Algebras, Linear. 2. Geometry, Projective. I. Title.
QA251.A7 2016
512.1 dc23
2015027103
Manufactured in the United States by RR Donnelley
80155101 2016
www.doverpublications.com
TO NATASCHA
PREFACE
Many parts of classical geometry have developed into great independent theories. Linear algebra, topology, differential and algebraic geometry are the indispensable tools of the mathematician of our time. It is frequently desirable to devise a course of geometric nature which is distinct from these great lines of thought and which can be presented to beginning graduate students or even to advanced undergraduates. The present book has grown out of lecture notes for a course of this nature given at New York University in 1955. This course centered around the foundations of affine geometry, the geometry of quadratic forms and the structure of the general linear group. I felt it necessary to enlarge the content of these notes by including projective and symplectic geometry and also the structure of the symplectic and orthogonal groups. Lack of space forced me to exclude unitary geometry and the quadratic forms of characteristic 2.
I have to thank in the first place my wife who helped greatly with the preparation of the manuscript and with the proofs. My thanks go also to George Bachman who with the help of Bernard Sohmer wrote the notes for the original course, to Larkin Joyner who drew the figures, and to Susan Hahn for helping with the proofreading.
E. A RTIN
SUGGESTIONS FOR THE USE OF THIS BOOK
The most important point to keep in mind is the fact that should be used mainly as a reference chapter for the proofs of certain isolated algebraic theorems. These proofs have been collected so as not to interrupt the main line of thought in later chapters.
An inexperienced reader should start right away with except for a few harder algebraic theorems which he should skip in a first reading.
This skipping is another important point. It should be done whenever a proof seems too hard or whenever a theorem or a whole paragraph does not appeal to the reader. In most cases he will be able to go on and later on he may return to the parts which were skipped.
The rest of the book definitely presupposes a good knowledge of suggests an exercise on which the reader can test his understanding of the preceding paragraphs. If he can do this exercise then he should be well equipped for the remainder of the book.
gives the theory of quadratic and of skew symmetric bilinear forms in a geometric language. For a first reading the symplectic geometry may be disregarded.
will be needed.
.
Any one of these chapters contains too much material for an advanced undergraduate course or seminar. I could make the following suggestions for the content of such courses.
1) The easier parts of .
2) The linear algebra of the first five paragraphs of , either on orthogonal or on symplectic geometry.
3) The fundamental theorem of projective geometry, followed by some parts of .
4) is the statement:
If W* is the space orthogonal to a subspace W of a non-singular space V then dim W + dim W* = dim V. This statement could be obtained from the naive theory of linear equations and the instructor could supply a proof of it. Our statement implies then W** = W and no further reference to is needed.
It is sufficient to know it for finite dimensional spaces only.
CHAPTER I
Preliminary Notions
1. Notions of set theory
We begin with a list of the customary symbols:
a S | means a is an element of the set S. |
ST | means S is a subset of T. |
ST | means the intersection of the sets S and T; should it be empty we call the sets disjoint. |
ST | stands for the union of S and T. |
iSi and iSi stand for intersection and union of a family of indexed sets. Should Si and Si be disjoint for ij we call iSi a disjoint union of sets. Sets are sometimes defined by a symbol {} where the elements are enumerated between the parenthesis or by a symbol {x|A} where A is a property required of x; this symbol is read: the set of all x with the property A. Thus, for example:
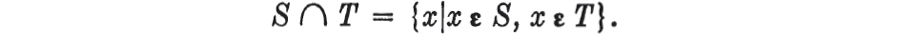
If f is a map of a non-empty set S into a set T, i.e., a function f(s) defined for all elements sS with values in T, then we write either

If 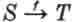 and
and 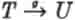 we also write
we also write  . If sS then we can form g(f(s)) U and thus obtain a map from S to U denoted by
. If sS then we can form g(f(s)) U and thus obtain a map from S to U denoted by 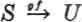 . Notice that the associative law holds trivially for these products of maps. The order of the two factors gf comes from the notation f(s) for the image of the elements. Had we written (s)f instead of f(s), it would have been natural to write fg instead of gf. Although we will stick (with rare exceptions) to the notation f(s) the reader should be able to do everything in the reversed notation. Sometimes it is even convenient to write sf instead of f(s) and we should notice that in this notation (sf)g = sgf.
. Notice that the associative law holds trivially for these products of maps. The order of the two factors gf comes from the notation f(s) for the image of the elements. Had we written (s)f instead of f(s), it would have been natural to write fg instead of gf. Although we will stick (with rare exceptions) to the notation f(s) the reader should be able to do everything in the reversed notation. Sometimes it is even convenient to write sf instead of f(s) and we should notice that in this notation (sf)g = sgf.
If 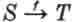 and S0S then the set of all images of elements of S0 is denoted by f(S0); it is called the image of S0. This can be done particularly for S itself. Then f(S) T; should f(S) = T we call the map onto and say that f maps S onto T.
and S0S then the set of all images of elements of S0 is denoted by f(S0); it is called the image of S0. This can be done particularly for S itself. Then f(S) T; should f(S) = T we call the map onto and say that f maps S onto T.
Let T0 be a subset of T. The set of all sS for which f(s) T0 is called the inverse image of T0 and is denoted by f1(T0). Notice that f1(T0) may very well be empty, even if T0 is not empty. Remember also that f1 is not a map. By f1(t) for a certain tT we mean the inverse image of the set {t} with the one element
Next page












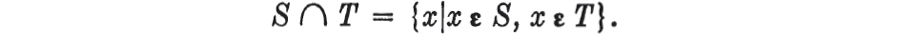

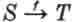 and
and 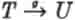 we also write
we also write  . If sS then we can form g(f(s)) U and thus obtain a map from S to U denoted by
. If sS then we can form g(f(s)) U and thus obtain a map from S to U denoted by 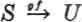 . Notice that the associative law holds trivially for these products of maps. The order of the two factors gf comes from the notation f(s) for the image of the elements. Had we written (s)f instead of f(s), it would have been natural to write fg instead of gf. Although we will stick (with rare exceptions) to the notation f(s) the reader should be able to do everything in the reversed notation. Sometimes it is even convenient to write sf instead of f(s) and we should notice that in this notation (sf)g = sgf.
. Notice that the associative law holds trivially for these products of maps. The order of the two factors gf comes from the notation f(s) for the image of the elements. Had we written (s)f instead of f(s), it would have been natural to write fg instead of gf. Although we will stick (with rare exceptions) to the notation f(s) the reader should be able to do everything in the reversed notation. Sometimes it is even convenient to write sf instead of f(s) and we should notice that in this notation (sf)g = sgf.