Hugh Barker - Million Dollar Maths: The Secret Maths of Becoming Rich (or Poor)

First published in hardback in Great Britain in 2018 by Atlantic Books, an imprint of Atlantic Books Ltd.

Copyright Hugh Barker, 2018
The moral right of Hugh Barker to be identified as the author of this work has been asserted by him in accordance with the Copyright, Designs and Patents Act of 1988.

All rights reserved. No part of this publication may be reproduced, stored in a retrieval system, or transmitted in any form or by any means, electronic, mechanical, photocopying, recording, or otherwise, without the prior permission of both the copyright owner and the above publisher of this book.

1 2 3 4 5 6 7 8 9

A CIP catalogue record for this book is available from the British Library.

Internal illustrations Diane Barker

Hardback ISBN: 978-1-78649-322-4

EBook ISBN: 978-1-78649-323-1

Printed in Great Britain
Atlantic Books
An Imprint of Atlantic Books Ltd
Ormond House
2627 Boswell Street
London
WC1N 3JZ

www.atlantic-books.co.uk

Annual income twenty pounds, annual expenditure nineteen [pounds] nineteen [shillings] and six [pence], result happiness. Annual income twenty pounds, annual expenditure twenty pounds ought and six, result misery.

Charles Dickens, David Copperfield

Like it or not, we live in a material world, in which money can help to create opportunities in life. We all know that money cant buy you love or happiness. But the lack of money can certainly lead to deprivation and frustration. So it is only natural that people with reasonable maths skills may occasionally ponder how that know-how can be used to maximize their wealth. Can they, for instance, manage their financial affairs or business better? Can they come up with a brilliant new mathematical idea or a related piece of technology? Or can they use their maths skills for more nefarious purposes, such as gambling systems or hacking and cracking?

The quote above, from David Copperfield by Charles Dickens, points to the fact that solvency is always preferable to bankruptcy. This is not the most astounding insight, although it is sound advice in its own way. But most of us would prefer to put away a bit more than sixpence a year for a rainy day. If were really honest, most of us would like to become as rich as possible. The self-help industry is hugely profitable largely because it sells the dream of rapid wealth for minimal effort. I wont be making that claim here, just exploring the many large and small ways that you can put maths to work for you.

Ill explore the many connections between maths and finance and the opportunities this creates for significant moneymaking. Ill include stories of famous investors, business people and gamblers who have used mathematical formulae or techniques in their work (Ill mostly avoid getting bogged down in value judgements about the morality of gambling and speculation as opposed to investment, though I will acknowledge where there are potential legal issues or other risks in a financial strategy). Modern technology also relies increasingly on maths, whether it be in the algorithms used by social media companies, the complex maths that underpins Bitcoin, or the ongoing battle between hackers, crackers and internet security experts. Ill also give quick summaries of things to do and things not to do as we go along.

The largest part of the book will focus on personal finance, gambling and investment, all of which can be easily understood by anyone with high school level maths. Some of this maths may already be obvious to you, but it is surprising how many people enjoy an occasional flutter without fully understanding the mathematics of the roulette table, or consult analytical tools such as the price-to-earnings ratio without realizing the intuitive and obvious way that this relates to interest rates. And when it comes to haggling over your salary, you may or may not know how game theory affects your chances of an increase.

Along the way well be meeting a strange assortment of problems that are also interesting from a purely mathematical point of view, from the Keynesian beauty contest to the Byzantine Generals Problem, and from the Kelly criterion to Maverick solitaire.

You dont need to be any kind of maths genius to put mathematical thinking to work in your everyday life. In fact most successful investors and businesspeople do not use complex maths, but instead rely on a clear understanding of how the numbers work, and of the mistakes we tend to make when we analyse data and probability. Avoiding irrational blunders can often be as crucial as making good judgement calls, and being familiar with the common mathematical and statistical errors people make is an enormous help.

Its not all easy maths later in the book Ill discuss the mathematics of the broader financial system and maths prizes and awards, and this cant be done without an attempt to outline the more complex maths involved. It would take a far more advanced mathematician than me to have a detailed knowledge of every single maths theorem I mention. Ill be honest and own up where Im getting out of my depth and will make it clear where the theory is likely to go beyond the reach of an amateur mathematician. But for the most part the maths required in this book is no more complex than you would learn at school.

If a man is proud of his wealth, he should not be praised until it is known how he employs it.

Socrates

If you ask fifty people what money is, youll get fifty different answers: its a peculiarly hard thing to define, so lets start by trying to pin that down. That definition will underpin the most basic ways you can make your money grow and help to explain why exponential growth is the key to successful wealth accumulation.

At its most basic, money is just a mathematical tool for counting and measuring value. In pre-monetary societies goods could be traded by barter in which, for instance, a sack of grain might have been swapped directly for pots or beans or for a days labour in the fields.

Lets imagine a transaction where one dairy cow was swapped for three bushels of wheat. We could use a visual equation to express their comparative value (see ).

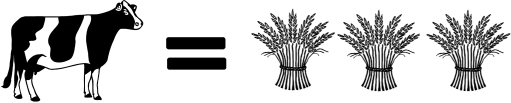
Figure 1. This represents the algebraic equation c = 3b (where c represents one cow and b represents one bushel).

But you can only use pure barter if you have exactly the goods the other party wants and vice versa. Otherwise, you can end up in complicated webs of buyers and sellers where person A gives person B a cow, they give their wheat to person C, person C gives person D some beehives, and they give person A their pots and pans. This would be monstrously tricky to choreograph. So, very quickly systems of money and credit were developed. By using tally sticks or other primitive records of trades, people could sell their goods or services and store a credit to be used for purchases at a later time. If we call the unit of currency