Daniele Faccio Francesco Belgiorno Sergio Cacciatori - Analogue Gravity Phenomenology
Here you can read online Daniele Faccio Francesco Belgiorno Sergio Cacciatori - Analogue Gravity Phenomenology full text of the book (entire story) in english for free. Download pdf and epub, get meaning, cover and reviews about this ebook. City: Cham, publisher: Springer International Publishing, genre: Home and family. Description of the work, (preface) as well as reviews are available. Best literature library LitArk.com created for fans of good reading and offers a wide selection of genres:
Romance novel
Science fiction
Adventure
Detective
Science
History
Home and family
Prose
Art
Politics
Computer
Non-fiction
Religion
Business
Children
Humor
Choose a favorite category and find really read worthwhile books. Enjoy immersion in the world of imagination, feel the emotions of the characters or learn something new for yourself, make an fascinating discovery.
- Book:Analogue Gravity Phenomenology
- Author:
- Publisher:Springer International Publishing
- Genre:
- City:Cham
- Rating:3 / 5
- Favourites:Add to favourites
- Your mark:
Analogue Gravity Phenomenology: summary, description and annotation
We offer to read an annotation, description, summary or preface (depends on what the author of the book "Analogue Gravity Phenomenology" wrote himself). If you haven't found the necessary information about the book — write in the comments, we will try to find it.
Daniele Faccio Francesco Belgiorno Sergio Cacciatori: author's other books
Who wrote Analogue Gravity Phenomenology? Find out the surname, the name of the author of the book and a list of all author's works by series.














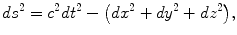
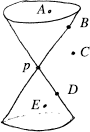

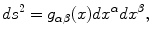
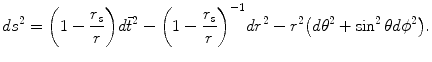
 does not reach any r < r s .)
does not reach any r < r s .)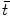 is the Schwarzschild time . It measures proper time at r =, whereas at any other fixed r , , the proper time interval is
is the Schwarzschild time . It measures proper time at r =, whereas at any other fixed r , , the proper time interval is 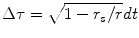 . The coefficients in the line element are independent of
. The coefficients in the line element are independent of 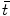 , hence the spacetime has a symmetry under
, hence the spacetime has a symmetry under 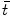 translation. This is ordinary time translation symmetry at r =, but it becomes a lightlike translation at r = r s , and a space translation symmetry for r < r s , since the coefficient of
translation. This is ordinary time translation symmetry at r =, but it becomes a lightlike translation at r = r s , and a space translation symmetry for r < r s , since the coefficient of 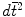 is negative there. The defining property of the Schwarzschild time coordinate, other than that it measures proper time in the rest frame of the black hole at infinity, is that surfaces of constant
is negative there. The defining property of the Schwarzschild time coordinate, other than that it measures proper time in the rest frame of the black hole at infinity, is that surfaces of constant 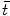 are orthogonal, in the spacetime sense, to the direction of the time-translation symmetry, i.e. to the lines of constant ( r ,,): there are no off-diagonal terms in the line element. But this nice property is also why
are orthogonal, in the spacetime sense, to the direction of the time-translation symmetry, i.e. to the lines of constant ( r ,,): there are no off-diagonal terms in the line element. But this nice property is also why