Greenberg - Foundations of Applied Mathematics

Fourier Transforms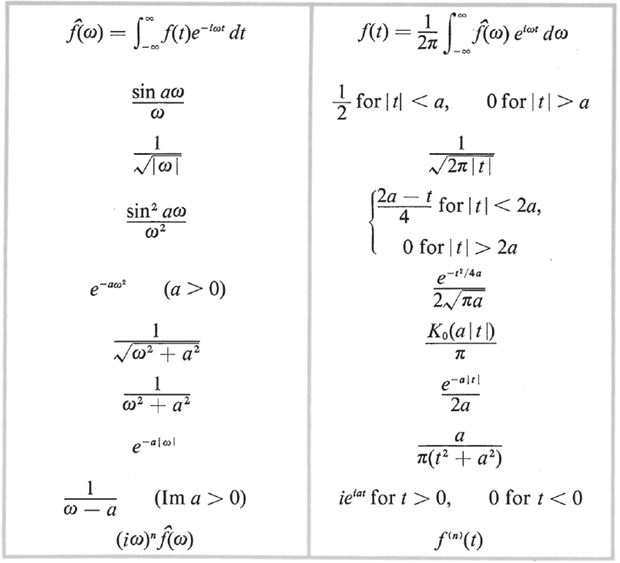Laplace Transforms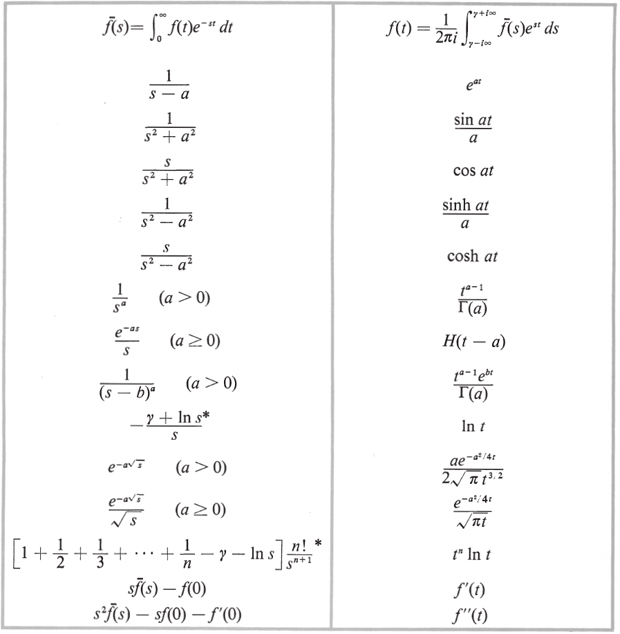

---

* = Eulers constant 0.577215665. FOUNDATIONS OF
APPLIED
MATHEMATICS Michael D. Greenberg Professor Emeritus
Department of Mechanical and Aerospace Engineering
University of Delaware Dover Publications, Inc.
Mineola, New York Copyright Copyright 1978 by Michael D. Greenberg
All rights reserved. Bibliographical Note This Dover edition, first published in 2013, is an unabridged republication of the work originally published by Prentice-Hall, Inc., Englewood Cliffs, N.J., in 1978. Library of Congress Cataloging-in-Publication Data Greenberg, Michael D., 1935author. Library of Congress Cataloging-in-Publication Data Greenberg, Michael D., 1935author.

Foundations of applied mathematics / Michael D. Greenberg, professor emeritus, Department of Mechanical and Aerospace Engineering, University of Delaware. Dover edition. p. cm. Summary: A longtime classic text in applied mathematics, this volume also serves as a reference for undergraduate and graduate students of engineering.

Topics include real variable theory, complex variables, linear analysis, partial and ordinary differential equations, and other subjects Answers to selected exercises are provided, along with Fourier and Laplace transformation tables and useful formulas. 1978 editionProvided by publisher. Includes bibliographical references and index. eISBN-13: 978-0-486-78218-8 1. Engineering mathematics. Title. Title.

TA330.G73 2013
620.001'51dc23 2013015816 Manufactured in the United States by Courier Corporation
49279601 2013
www.doverpublications.com This book is dedicated,
with love, to Mim. Contents by PartPartPrefacePurpose. This book is intended primarily as a text for a single- or multi-semester course in applied mathematics for first-year graduate students or seniors in engineering. It grew out of a set of lecture notes for a two-semester course that I taught both at Cornell University and at the University of Delaware, a course taken primarily by first-year graduate students in applied mechanics, materials science, mechanical, chemical, civil, and aerospace engineering, as well as some undergraduates and a number of people from electrical engineering, oceanography, chemistry, and astronomy. Prerequisites. Prerequisites consist of the usual undergraduate four-semester sequence in calculus and ordinary differential equations, together with the general maturity and background of a senior or beginning graduate student.

Knowledge of computer programming is not required. Content and Organization. In books of this type it is tempting to try to include something for everyone, that is, diverse physical applications from all branches of engineering, applied physics, and so on. Instead we have elected to concentrate on only a few areas of application, including fluid mechanics, heat conduction, and Newtonian mechanics. Discussion of such topics is self-contained; for instance, the governing equations of fluid mechanics and heat conduction are developed in the section on vector field theory before solutions are sought in the later sections on partial differential equations. As a result, these physical concepts weave through the mathematics and act as a unifying element.

I try to emphasize not only how the mathematics permits us to clarify and understand the physics but also how our physical insight can support the mathematics and provide the key to finding the appropriate mathematical line of approach. Practical and numerical aspects are emphasized as well. We begin in . Although the most natural sequence of topics is , which deals with that subject in more detail. Ive also tried to refer the reader back to any specific formulas or results that are used and that appeared in an earlier part of the book. It should be possible to begin with any of (not including the starred material), however, I suggest the following preliminary reading: For Part IV: .

For Part V: . In addition, some complex variable theory is used in . Suggested Courses. TWO OR THREE SEMESTERS: In the two-semester course that I teach we cover about 90 percent of the material. This means that the pace is rather fast and does not permit class time to be used for both lecture and the discussion of assigned homework. Instead, I use class time for lecture and simply make the Solutions Manual available to the students to assist them in working out the suggested Exercises.

The grade is based solely on two or three examinations with at least one class before each exam reserved for questions and review. At a more leisurely pace, the entire book could be covered in three semesters. ONE SEMESTER: A natural one-semester course might cover only, and would amount to a course in Advanced Calculus for Scientists and Engineers. Starred Material. A certain amount of starred material is included. Exercises. Exercises.

The Exercises at the end of each chapter cover the material in that chapter and introduce additional material as well. They are arranged in the same order as the topics within the chapter and are not ordered in terms of their degree of difficulty. Starred problems, however, are generally more difficult and/or are based on material from starred sections within the chapter. References. Besides the references cited in footnotes, a list of Additional References appears at the end of each part. Acknowledgment. Acknowledgment.

I would like to extend special thanks to my department chairperson, Professor Jack R. Vinson, who has encouraged me in this time-consuming endeavor, to my students who are a continuing source of inspiration and friendship, and to Miss Leslie A. Month in particular. Finally, I am also grateful to Professors A. Eshett, Peter J. Gingo, Jerry Kazdan, Ronald J.

Lomax, Ivar Stakgold, Dale W. Thoe, and Erich Zauderer for offering helpful comments on the manuscript. Closure. In spite of all precautions, errors will no doubt be uncovered in using this book, and I would like to encourage you to let me know about them, if you have the time, so that they can be corrected. Beyond that, any comments, suggestions, or ideas that you may wish to share would be most appreciated. MICHAEL D. MICHAEL D.

GREENBERG PART IREAL VARIABLE THEORY. Besides discussing FUNCTIONS OF SEVERAL VARIABLES, VECTORS, SURFACES AND VOLUMES, VECTOR FIELD THEORY, and THE CALCULUS OF VARIATIONS, the final four chapters provide self-contained introductions to the physics and governing differential equations of heat transfer, fluid mechanics, strings and membranes, and the gravitational potential, so that these subject areas will be readily available for many of the applications that form an important part of the rest of the book. Chapter 1The Important Limit Processes In this first chapter we review a number of fundamentals: functions, functionals, limits, continuity, and uniform continuity. Then we look at two important limit processes, differentiation and integration, the latter in some detail. Practical and computational aspects are emphasized as well. 1.1.

FUNCTIONS AND FUNCTIONALS Recall that a functionf is a mapping from one set, called the domain of f, to another, called the range of f. Suppose, for instance, that f is defined by f (

Next page