Relativistic Wave Mechanics
Lectures by
E. CORINALDESI
Edited by
F. STROCCHI
English text prepared with assistance from
R. HILTON
Dover Publications, Inc.
Mineola, New York
Bibliographical Note This Dover edition, first published in 2015, is an unabridged republication of the work originally published by North-Holland Publishing Company, Amsterdam, and John Wiley & Sons, Inc., New York, in 1963. The English text was prepared with assistance from R.
Library of Congress Cataloging-in-Publication Data Corinaldesi, E., author.
Library of Congress Cataloging-in-Publication Data Corinaldesi, E., author.
Relativistic wave mechanics / E. Corinaldesi; edited by F. Strocchi; English text prepared with assistance from R. Hilton. p. cm.
This Dover edition, first published in 2015, is an unabridged republication of the work originally published by North-Holland Publishing Company, Amsterdam, and John Wiley & Sons, Inc., New York, in 1963. The English text was prepared with assistance from R. HiltonTitle page verso. Includes bibliographical references and index. eISBN-13: 978-0-486-80573-3 1. 2. 2.
Relativity (Physics) I. Strocchi, F., editor. II. Title. QC174.2.C645 2015 530.11dc23 2014048064 Manufactured in the United States by Courier Corporation 79377X012015 www.doverpublications.com
PREFACE
During the academic years 195960 and 196061, Prof. A. A.
Radicat was on leave from the University of Pisa and asked me to lecture in his place to fourth-year students on Advanced Quantum Mechanics. This book originates from those lectures. Dr. Franco Strocchi, then a student in my class, volunteered to go through and amplify the notes I handed to him at the end of each lecture and edited them in mimeographed form (in Italian) for the use of his fellow students. In the Spring of 1961, while visiting University College London, I made a rough translation into English of the Introduction and . The manuscript was ready by the end of the Summer.
The helpful and constructive criticism of the referee, Prof. S. A. Wouthuysen, led to improvements and to my writing a number of additional sections during the year 196162 while I was at the Institute for Advanced Study at Princeton. Finally, I used the whole contents of this book as the basis for a lecture course at the State University of Iowa during the Winter of 1962. Each member of the class generously helped me and Strocchi, while we were correcting the galley proofs, with innumerable suggestions leading to many amendments.
One of them, Louis A. Frank, deserves special thanks for his enthusiastic interest. This book is thus the result of close collaboration and discussions with students. Its purpose is to take the reader, who already has some knowledge of non-relativistic wave mechanics, as far as the borderline between relativistic wave mechanics and field theory, so that he may more confidently attack the study of the latter. No problems are set for the reader to work out on his own. Such problems as were assigned to the students who followed the courses have been incorporated in the text as Notes.
Those on pp. 106110 are due to Dr. A. Di Giacomo of Pisa. The inclusion of a list of notations has seemed unnecessary. E. C. C.
Department of Physics, University of Toronto September, 1963
CONTENTS
INTRODUCTION
PARTICLES AND WAVE FUNCTIONS
1. De Broglies relation
At the end of the last century it seemed possible to interpret all known physical phenomena in terms of electromagnetic waves and charged particles, but the discoveries of the twentieth century have brought about a radical revision of classical concepts. The theory of
blackbody radiation (Planck 1900) and the explanation of the
photoelectric effect (Einstein 1905) were based on the hypothesis that exchanges of energy between matter and radiation took place in quanta
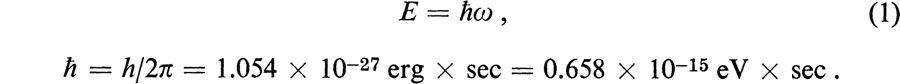
The subsequent discovery of the
Compton effect (Compton 1923) showed that a quantum associated with a plane wave has a
momentum p given by

where
k is the
wave propagation vector, (briefly,
wavevector)
, |k| = 2/ =
/c. The relation between the wave properties of radiation and the particle properties of the associated quanta is relativistically invariant. In fact
k1
, k2,
k3 and i
/c are the components of a four-vector, as may be shown from the invariance of the phase of a monochromatic plane wave. On the other hand it is known that the components of the momentum and the energy of a particle can be regarded as the space and time components of the four-momentum
p = (
p, i
E/c).
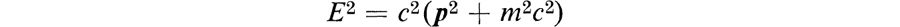
can be written in the form
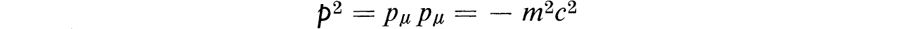
and, since
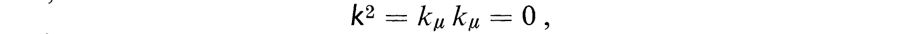
we have
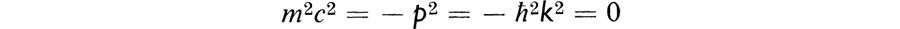
for the quanta of the electromagnetic radiation.
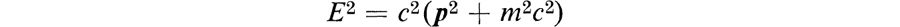
can be written in the form
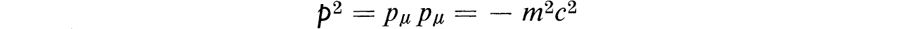
and, since
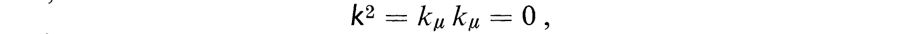
we have
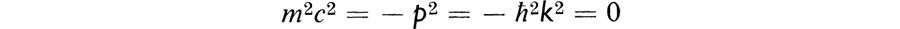
for the quanta of the electromagnetic radiation.
This shows that the rest mass of a photon is zero. De Broglies postulate of matter waves, which was based on purely theoretical arguments, can be condensed in the statement that the relation p = k, where 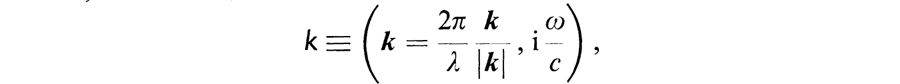 between the wave four-vector and the four-momentum holds not only for photons, but also for particles of mass not equal to zero. In the case of electromagnetic radiation the wave aspect was discovered much earlier than the corpuscular, for material particles the opposite has been the case. N OTE 1. Using the conservation of four-momentum, the expression
between the wave four-vector and the four-momentum holds not only for photons, but also for particles of mass not equal to zero. In the case of electromagnetic radiation the wave aspect was discovered much earlier than the corpuscular, for material particles the opposite has been the case. N OTE 1. Using the conservation of four-momentum, the expression 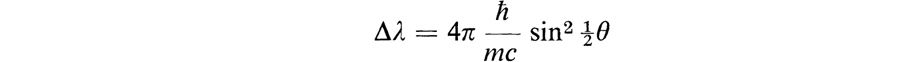 for the change in wavelength in the Compton effect, may be cast into a Lorentz invariant form. This amounts to finding an invariant equation for the four-momenta p and p of the electron, and k and k of the photon, before and after collision, which reduces to the given formula in the reference frame in which the electron is initially at rest (
for the change in wavelength in the Compton effect, may be cast into a Lorentz invariant form. This amounts to finding an invariant equation for the four-momenta p and p of the electron, and k and k of the photon, before and after collision, which reduces to the given formula in the reference frame in which the electron is initially at rest (










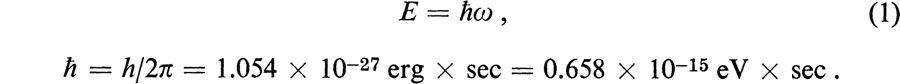 The subsequent discovery of the Compton effect (Compton 1923) showed that a quantum associated with a plane wave has a momentum p given by
The subsequent discovery of the Compton effect (Compton 1923) showed that a quantum associated with a plane wave has a momentum p given by  where k is the wave propagation vector, (briefly, wavevector), |k| = 2/ = /c. The relation between the wave properties of radiation and the particle properties of the associated quanta is relativistically invariant. In fact k1, k2, k3 and i/c are the components of a four-vector, as may be shown from the invariance of the phase of a monochromatic plane wave. On the other hand it is known that the components of the momentum and the energy of a particle can be regarded as the space and time components of the four-momentum p = (p, iE/c).
where k is the wave propagation vector, (briefly, wavevector), |k| = 2/ = /c. The relation between the wave properties of radiation and the particle properties of the associated quanta is relativistically invariant. In fact k1, k2, k3 and i/c are the components of a four-vector, as may be shown from the invariance of the phase of a monochromatic plane wave. On the other hand it is known that the components of the momentum and the energy of a particle can be regarded as the space and time components of the four-momentum p = (p, iE/c). 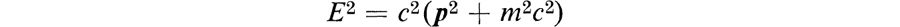 can be written in the form
can be written in the form 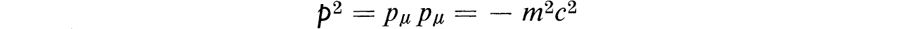 and, since
and, since 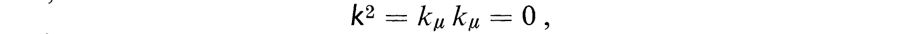 we have
we have 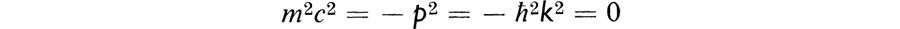 for the quanta of the electromagnetic radiation.
for the quanta of the electromagnetic radiation. 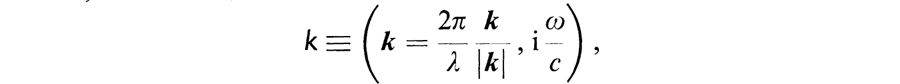 between the wave four-vector and the four-momentum holds not only for photons, but also for particles of mass not equal to zero. In the case of electromagnetic radiation the wave aspect was discovered much earlier than the corpuscular, for material particles the opposite has been the case. N OTE 1. Using the conservation of four-momentum, the expression
between the wave four-vector and the four-momentum holds not only for photons, but also for particles of mass not equal to zero. In the case of electromagnetic radiation the wave aspect was discovered much earlier than the corpuscular, for material particles the opposite has been the case. N OTE 1. Using the conservation of four-momentum, the expression 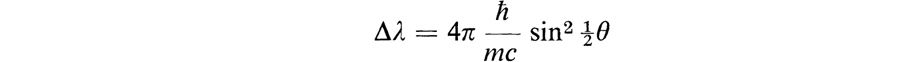 for the change in wavelength in the Compton effect, may be cast into a Lorentz invariant form. This amounts to finding an invariant equation for the four-momenta p and p of the electron, and k and k of the photon, before and after collision, which reduces to the given formula in the reference frame in which the electron is initially at rest (
for the change in wavelength in the Compton effect, may be cast into a Lorentz invariant form. This amounts to finding an invariant equation for the four-momenta p and p of the electron, and k and k of the photon, before and after collision, which reduces to the given formula in the reference frame in which the electron is initially at rest (