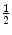1. Introduction
1.1 From the Hall Effect to Quantum Spin Hall Effect
In 1879, the American physicist Edwin H. Hall observed an effect that now bears his name: he measured a voltage that arises from the deflected motion of charged particles in solids under external electric field and magnetic field []. Consider a two-dimensional sample subjected to a perpendicular magnetic field B . When charged particles go through the sample, the particles deflect their motion due to the Lorentz force and accumulate near the boundary. As a result the charge accumulation at the boundary produces an electric field E . In equilibrium, the Lorentz force on a moving charged particle becomes zero:
where v is the velocity of the particle and q the charge of the particle. The voltage difference between the two boundaries is V H = EW ( W the width of the sample), and the electric current through the sample is I = q e W ( e is the density of the charge carriers). The ratio of the voltage to the electric current is known as the Hall resistance:
which is linear in the magnetic field B . In practice, the Hall effect is used to measure the sign of charge carriers q , that is, the particle-like or hole-like charge carrier, and the density of charge carriers e in solids. It can be also used to measure the magnetic field.
In the following year after his discovery, Hall measured the Hall resistance in ferromagnetic or even in paramagnetic metal in a magnetic field and observed that the Hall resistance could have additional contribution other than the linear term in magnetic field []. It could be proportional to the magnetization M in a ferromagnetic metal, that is, the Hall effect persists even in the absence of a magnetic field. An empirical relation is given by
which has been applied to many materials over a broad range of external field. The second term represents the contribution from the magnetization M . This part of resistance cannot be simply understood as a result of the Lorentz force on a charged particle. It has taken almost one century to explore its physical origin. The main reason seems to be that this effect involves the topology of the band structure in solids, which had been just formulated in the 1980s. In 1954, Karplus and Luttinger [].
Generally speaking, the anomalous Hall effect can have either an extrinsic origin due to disorder-related spin-dependent scattering of the charge carriers, or an intrinsic origin due to spin-dependent band structure of conduction electrons, which can be expressed in terms of the Berry phase in the momentum space []. As a result, electrons with spin-up will deflect to one direction, while electrons with spin-down to the opposite direction. In a ferromagnetic metal, the magnetization will cause an imbalance in the population between the electrons with spin-up and spin-down and consequently lead to the anomalous Hall effect.
While the anomalous Hall resistance vanishes in the absence of an external magnetic field and magnetization in a paramagnetic metal, the spin-dependent deflected motion of electrons in solids can still lead to an observable effect, that is, the spin Hall effect. The spin version of the Hall effect was first proposed by the Russian physicists Dyakonov and Perel in 1971 [].
The discovery of the integer quantum Hall effect opens a new phase in the study of the Hall effects. In 1980, von Klitzing, Dorda, and Pepper discovered experimentally that in two-dimensional electron gas at semiconductor hetero-junction subjected to a strong magnetic field, the longitudinal conductance becomes zero while the quantum plateau of the Hall conductance appears at e 2 h [].
To understand further, physicists like to use a semiclassical picture to explain the quantization of the Hall conductance. For a charged particle in a uniform magnetic field, the particle cycles around the magnetic flux rapidly because of the Lorentz force. The radius of the cycle is given by the magnetic field
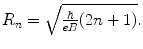
When the particle is close to the boundary, the particle bounces back from the rigid boundary and skips along the boundary forward. As a result, it forms a conducting channel along the boundary, which is called the edge state. The group velocity of the particle in the bulk is much slower than the cyclotron velocity, and then the particles in the bulk are intended to be pinned or localized by impurities or disorders. However, the rapid-moving particles along the edge channel are not affected by the impurities or disorders and form a perfect one-dimensional conducting channel with a quantum conductance e 2 h . Consider the Landau levels are discrete. Each Landau level will generate one edge channel. Consequently, the number of the filled Landau levels or the filling factor determines the quantized Hall conductance. Thus, the key feature of the quantum Hall effect is that all electrons in the bulk are localized and the electrons near the edges form a series of edge-conducting channels [], which is a characteristic of a topological phase.
In 1988 Haldane proposed that the integer quantum Hall effect could be realized in a lattice system of spinless electrons in a periodic magnetic flux [], this effect has not yet been observed.
The quantum spin Hall effect is a quantum version of the spin Hall effect or a spin version of the quantum Hall effect and can be regarded as a combination of two quantum anomalous Hall effects of spin-up and spin-down electrons with opposite chirality. Overall it has no charge Hall conductance, but a nonzero spin Hall conductance. In 2005, Kane and Mele generalized the Haldane model to a graphene lattice of spin-















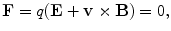

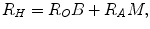
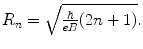 When the particle is close to the boundary, the particle bounces back from the rigid boundary and skips along the boundary forward. As a result, it forms a conducting channel along the boundary, which is called the edge state. The group velocity of the particle in the bulk is much slower than the cyclotron velocity, and then the particles in the bulk are intended to be pinned or localized by impurities or disorders. However, the rapid-moving particles along the edge channel are not affected by the impurities or disorders and form a perfect one-dimensional conducting channel with a quantum conductance e 2 h . Consider the Landau levels are discrete. Each Landau level will generate one edge channel. Consequently, the number of the filled Landau levels or the filling factor determines the quantized Hall conductance. Thus, the key feature of the quantum Hall effect is that all electrons in the bulk are localized and the electrons near the edges form a series of edge-conducting channels [], which is a characteristic of a topological phase.
When the particle is close to the boundary, the particle bounces back from the rigid boundary and skips along the boundary forward. As a result, it forms a conducting channel along the boundary, which is called the edge state. The group velocity of the particle in the bulk is much slower than the cyclotron velocity, and then the particles in the bulk are intended to be pinned or localized by impurities or disorders. However, the rapid-moving particles along the edge channel are not affected by the impurities or disorders and form a perfect one-dimensional conducting channel with a quantum conductance e 2 h . Consider the Landau levels are discrete. Each Landau level will generate one edge channel. Consequently, the number of the filled Landau levels or the filling factor determines the quantized Hall conductance. Thus, the key feature of the quantum Hall effect is that all electrons in the bulk are localized and the electrons near the edges form a series of edge-conducting channels [], which is a characteristic of a topological phase.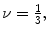

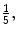
 ,
,  known as the fractional quantum Hall effect []. This picture is applicable to all the quantum plateaus observed in the fractional quantum Hall effect. Now it is well accepted that the fractional quantum Hall effect is a topological quantum phase of composite fermions, which breaks time reversal symmetry.
known as the fractional quantum Hall effect []. This picture is applicable to all the quantum plateaus observed in the fractional quantum Hall effect. Now it is well accepted that the fractional quantum Hall effect is a topological quantum phase of composite fermions, which breaks time reversal symmetry.