Carlo Alabiso - A Primer on Hilbert Space Theory: Linear Spaces, Topological Spaces, Metric Spaces, Normed Spaces, and Topological Groups (UNITEXT for Physics)
Here you can read online Carlo Alabiso - A Primer on Hilbert Space Theory: Linear Spaces, Topological Spaces, Metric Spaces, Normed Spaces, and Topological Groups (UNITEXT for Physics) full text of the book (entire story) in english for free. Download pdf and epub, get meaning, cover and reviews about this ebook. year: 2021, publisher: Springer, genre: Children. Description of the work, (preface) as well as reviews are available. Best literature library LitArk.com created for fans of good reading and offers a wide selection of genres:
Romance novel
Science fiction
Adventure
Detective
Science
History
Home and family
Prose
Art
Politics
Computer
Non-fiction
Religion
Business
Children
Humor
Choose a favorite category and find really read worthwhile books. Enjoy immersion in the world of imagination, feel the emotions of the characters or learn something new for yourself, make an fascinating discovery.
- Book:A Primer on Hilbert Space Theory: Linear Spaces, Topological Spaces, Metric Spaces, Normed Spaces, and Topological Groups (UNITEXT for Physics)
- Author:
- Publisher:Springer
- Genre:
- Year:2021
- Rating:3 / 5
- Favourites:Add to favourites
- Your mark:
A Primer on Hilbert Space Theory: Linear Spaces, Topological Spaces, Metric Spaces, Normed Spaces, and Topological Groups (UNITEXT for Physics): summary, description and annotation
We offer to read an annotation, description, summary or preface (depends on what the author of the book "A Primer on Hilbert Space Theory: Linear Spaces, Topological Spaces, Metric Spaces, Normed Spaces, and Topological Groups (UNITEXT for Physics)" wrote himself). If you haven't found the necessary information about the book — write in the comments, we will try to find it.
This book offers an essential introduction to the theory of Hilbert space, a fundamental tool for non-relativistic quantum mechanics. Linear, topological, metric, and normed spaces are all addressed in detail, in a rigorous but reader-friendly fashion. The rationale for providing an introduction to the theory of Hilbert space, rather than a detailed study of Hilbert space theory itself, lies in the strenuous mathematics demands that even the simplest physical cases entail. Graduate courses in physics rarely offer enough time to cover the theory of Hilbert space and operators, as well as distribution theory, with sufficient mathematical rigor. Accordingly, compromises must be found between full rigor and the practical use of the instruments. Based on one of the authorss lectures on functional analysis for graduate students in physics, the book will equip readers to approach Hilbert space and, subsequently, rigged Hilbert space, with a more practical attitude. It also includes a brief introduction to topological groups, and to other mathematical structures akin to Hilbert space. Exercises and solved problems accompany the main text, offering readers opportunities to deepen their understanding. The topics and their presentation have been chosen with the goal of quickly, yet rigorously and effectively, preparing readers for the intricacies of Hilbert space. Consequently, some topics, e.g., the Lebesgue integral, are treated in a somewhat unorthodox manner. The book is ideally suited for use in upper undergraduate and lower graduate courses, both in Physics and in Mathematics.
Carlo Alabiso: author's other books
Who wrote A Primer on Hilbert Space Theory: Linear Spaces, Topological Spaces, Metric Spaces, Normed Spaces, and Topological Groups (UNITEXT for Physics)? Find out the surname, the name of the author of the book and a list of all author's works by series.
A Primer on Hilbert Space Theory: Linear Spaces, Topological Spaces, Metric Spaces, Normed Spaces, and Topological Groups (UNITEXT for Physics) — read online for free the complete book (whole text) full work
Below is the text of the book, divided by pages. System saving the place of the last page read, allows you to conveniently read the book "A Primer on Hilbert Space Theory: Linear Spaces, Topological Spaces, Metric Spaces, Normed Spaces, and Topological Groups (UNITEXT for Physics)" online for free, without having to search again every time where you left off. Put a bookmark, and you can go to the page where you finished reading at any time.
Font size:
Interval:
Bookmark:
UNITEXT for Physics series, formerly UNITEXT Collana di Fisica e Astronomia, publishes textbooks and monographs in Physics and Astronomy, mainly in English language, characterized of a didactic style and comprehensiveness. The books published in UNITEXT for Physics series are addressed to upper undergraduate and graduate students, but also to scientists and researchers as important resources for their education, knowledge and teaching.
More information about this series at http://www.springer.com/series/13351
This Springer imprint is published by the registered company Springer Nature Switzerland AG
The registered company address is: Gewerbestrasse 11, 6330 Cham, Switzerland
Ittay Weiss dedicates the book to his daughters, Eugenia and Esther, for allowing him to work on it.
Other than correction of typos, stylistic improvements, and the addition of many new exercises, two new chapters have been added to the book offering a non-classical view of the classical spaces and a guided tour of some of the main attractions of Hilbert space land. The new material creates a more evenly spread experience across the text and interjects the abstract ambient theory with more concrete scenarios.
The classical sequence spaces 
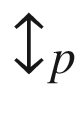and function spaces 
are fundamental and go hand-in-hand with the general theory. However, the function spaces are technically demanding and so they were only superficially treated in the first edition. The new chapter devoted to the classical spaces builds on the metric machinery already in place and attempts to create a clear conceptual framework that emphasizes the similarities between the two families of spaces. This approach complements the standard measure-theoretic presentations found in the literature.
The chapter devoted to Hilbert spaces is the new closing chapter of the book and is written in the form of a discussion. It highlights the practical simplification borne by the presence of an inner product as it draws upon the material in the previous chapters to compare against the situation in Banach spaces. In this way, the chapter serves both as a guided tour for the readers future journey to Hilbert space theory and a retrospective reflection on its preceding chapters. The topics covered are classical in Hilbert space theory but as they are handled here after a preliminary exposition of Banach spaces, the chapter takes the opportunity to create a richer historical context provided by a brief account of famous results in the development of the general theory.
The book consists of eight chapters with an additional chapter of solved problems arranged by topic. Each chapter is composed of five sections, with each section accompanied by a set of exercises (with the exception of the shorter Chapter 7 with just a single batch of exercises). The total of 422 exercises and 50 solved problems comprise an integral part of the book designed to assist the reader and hone her intuition.
Chapter 1 is a general introduction to analysis and, in particular, to each of the subjects presented in the chapters that follow. Chapter 1 also contains a Preliminaries section, intended to quickly orient the reader as to the notation and concepts used throughout the book, starting with sets and ending with an axiomatic presentation of the real and complex numbers.
Chapter 2 is devoted to linear spaces. At the advanced undergraduate level, the reader is already familiar with at least some aspects of linear spaces, primarily finite-dimensional ones. The chapter does not rely on any previous knowledge though, and is in that sense self-contained. However, the material is somewhat advanced since the focus is the technically and conceptually demanding infinite-dimensional linear spaces.
Chapter 3 is an introduction to topology, a subject considered to be at a rather high level of abstraction. The main aim of the chapter is to familiarize the reader with the fundamentals of the theory, particularly the portions that are most directly relevant for analysis and Hilbert spaces. Care is taken to find a reasonable balance between the study of extreme topology, i.e., spaces or phenomena that one may consider pathological but that hone the topological intuition, and mundane topology, i.e., those spaces or phenomena one is most likely to find in nature but that may obscure the true nature of topology.
Font size:
Interval:
Bookmark:
Similar books «A Primer on Hilbert Space Theory: Linear Spaces, Topological Spaces, Metric Spaces, Normed Spaces, and Topological Groups (UNITEXT for Physics)»
Look at similar books to A Primer on Hilbert Space Theory: Linear Spaces, Topological Spaces, Metric Spaces, Normed Spaces, and Topological Groups (UNITEXT for Physics). We have selected literature similar in name and meaning in the hope of providing readers with more options to find new, interesting, not yet read works.
Discussion, reviews of the book A Primer on Hilbert Space Theory: Linear Spaces, Topological Spaces, Metric Spaces, Normed Spaces, and Topological Groups (UNITEXT for Physics) and just readers' own opinions. Leave your comments, write what you think about the work, its meaning or the main characters. Specify what exactly you liked and what you didn't like, and why you think so.