Manhattan GMAT - Manhattan GMAT Strategy Guide 3 : Word Problems

Data Sufficiency (DS) problems are a cross between math and logic. Imagine that your boss just walked into your office and dumped a bunch of papers on your desk, saying, Hey, our client wants to know whether they should raise the price on this product. Can you answer that question from this data? If so, which pieces do we need to prove the case? What would you do?

The client has asked a specific question: should the company raise the price? You have to decide which pieces of information will allow you to answer that questionor, possibly, that you don't have enough information to answer the question at all.

This kind of logical reasoning is exactly what you use when you answer DS questions.

How Data Sufficiency Works

If you already feel comfortable with the basics of Data Sufficiency, you may want to move quickly through this particular section of the chapterbut you are encouraged to read it. There are a few insights that you may find useful.

Every DS problem has the same basic form:

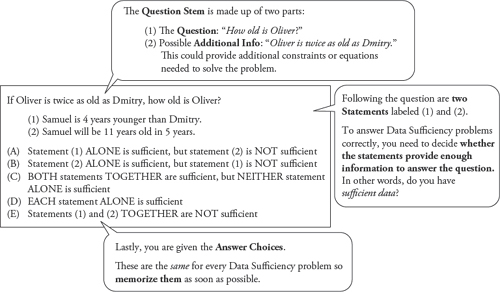
The question stem contains the question you need to answer. The two statements provide given information, information that is true. DS questions look strange but you can think of them as deconstructed Problem Solving (PS) questions. Compare the DS-format problem above to the PS-format problem below:

Samuel is 4 years younger than Dmitry, and Samuel will be 11 years old in 5 years. If Oliver is twice as old as Dmitry, how old is Oliver?

The two questions contain exactly the same information; that information is just presented in a different order. The PS question stem contains all of the givens as well as the question. The DS problem moves some of the givens down to statement (1) and statement (2).

As with regular PS problems, the given information in the DS statements is always true. In addition, the two statements won't contradict each other. In the same way that a PS question wouldn't tell you that x > 0 and x < 0, the two DS statements won't do that either.

In the PS format, you would go ahead and calculate Oliver's age. The DS format works a bit differently. Here is the full problem, including the answer choices:

If Oliver is twice as old as Dmitry, how old is Oliver?

(1) Samuel is 4 years younger than Dmitry.

(2) Samuel will be 11 years old in 5 years.

(A) Statement (1) ALONE is sufficient, but statement (2) is NOT sufficient.

(B) Statement (2) ALONE is sufficient, but statement (1) is NOT sufficient.

(C) BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.

(D) EACH statement ALONE is sufficient.

(E) Statements (1) and (2) TOGETHER are NOT sufficient.

Despite all appearances, the question is not actually asking you to calculate Oliver's age. Rather, it's asking which pieces of information would allow you to calculate Oliver's age.

You may already be able solve this one on your own, but you'll see much harder problems on the test, so your first task is to learn how to work through DS questions in a systematic, consistent way.

As you think the problem through, jot down information from the question stem:

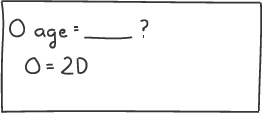
Hmm. If they tell you Dmitry's age, then you can find Oliver's age. Remember that!

Take a look at the first statement. Also, write down the 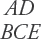answer grid (you'll learn why as you work through the problem):

(1) Samuel is 4 years younger than Dmitry.

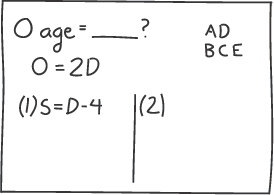
The first statement doesn't allow you to figure out anyone's real age. Statement (1), then, is not sufficient. Now you can cross off the top row of answers, (A) and (D).

Why? Here's the text for answers (A) and (D):

(A) Statement (1) ALONE is sufficient, but statement (2) is NOT sufficient.

(D) EACH statement ALONE is sufficient.

Both answers indicate that statement (1) is sufficient to answer the question. Because statement (1) is not sufficient to find Oliver's age, both (A) and (D) are wrong.

The answer choices will always appear in the order shown for the above problem, so any time you decide that statement (1) is not sufficient, you will always cross off answers (A) and (D). That's why your answer grid groups these two answers together.

Next, consider statement (2), but remember one tricky thing: forget what statement (1) told you. Because of the way DS is constructed, you must evaluate the two statements separately before you look at them together:

(2) Samuel will be 11 years old in 5 years.

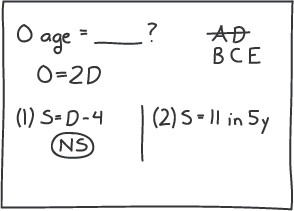
It's useful to write the two statements side-by-side, as shown above, to help remember that statement (2) is separate from statement (1) and has to be considered by itself first.

Statement (2) does indicate how old Sam is now, but says nothing about Oliver or Dmitry. (Remember, you're looking only at statement (2) now.) By itself, statement (2) is not sufficient, so cross off answer (B).

Now that you've evaluated each statement by itself, take a look at the two statements together. Statement (2) provides Sam's age, and statement (1) allows you to calculate Dmitry's age if you know Sam's age. Finally, the question stem allows you to calculate Oliver's age if you know Dmitry's age:

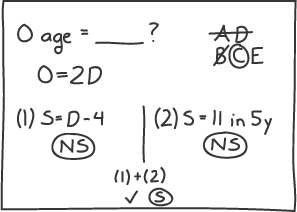
As soon as you can tell that you can solve, put down a check mark or write an S with a circle around it (or both!). Don't actually calculate Oliver's age; the GMAT doesn't give you any extra time to calculate a number that you don't need.

The correct answer is (C).

The Answer Choices

The five Data Sufficiency answer choices will always be exactly the same (and presented in the same order), so memorize them before you go into the test.

Here are the five answers written in an easier way to understand:

(A) Statement (1) does allow you to answer the question, but statement (2) does not.

(B) Statement (2) does allow you to answer the question, but statement (1) does not.

(C) Neither statement works on its own, but you can use them together to answer the question.

(D) Statement (1) works by itself and statement (2) works by itself.

(E) Nothing works. Even if you use both statements together, you still can't answer the question.

Answer (C) specifically says that neither statement works on its own. For this reason, you are required to look at each statement by itself and decide that neither one works before you are allowed to evaluate the two statements together.

Here's an easier way to remember the five answer choices; we call this the twelve-ten mnemonic (memory aid):

Within the next week, memorize the DS answers. If you do a certain number of practice DS problems in that time frame, you'll likely memorize the answers without conscious effortand you'll solidify the DS lessons you're learning right now.