Pascal Gadaud - Crystal Elasticity

to Philippe M.
for having conveyed to me his
taste for experimental work and
hopefully some of his skill

to Jacques W.
for having transmitted to me his
scientific curiosity even if it
may involve calling everything
into question every morning

Series Editor

Gilles Pijaudier-Cabot

Pascal Gadaud

First published 2022 in Great Britain and the United States by ISTE Ltd and John Wiley & Sons, Inc.

Apart from any fair dealing for the purposes of research or private study, or criticism or review, as permitted under the Copyright, Designs and Patents Act 1988, this publication may only be reproduced, stored or transmitted, in any form or by any means, with the prior permission in writing of the publishers, or in the case of reprographic reproduction in accordance with the terms and licenses issued by the CLA. Enquiries concerning reproduction outside these terms should be sent to the publishers at the undermentioned address:

ISTE Ltd
27-37 St Georges Road
London SW19 4EU
UK

www.iste.co.uk

John Wiley & Sons, Inc.
111 River Street
Hoboken, NJ 07030
USA

www.wiley.com

ISTE Ltd 2022

The rights of Pascal Gadaud to be identified as the author of this work have been asserted by him in accordance with the Copyright, Designs and Patents Act 1988.

Any opinions, findings, and conclusions or recommendations expressed in this material are those of the author(s), contributor(s) or editor(s) and do not necessarily reflect the views of ISTE Group.

Library of Congress Control Number: 2022933477

British Library Cataloguing-in-Publication Data

A CIP record for this book is available from the British Library

ISBN 978-1-78630-822-1

The first part of this book focuses on the description of crystal elasticity. This field became fully established at the beginning of the 20th century. Its ancestral branches can be referred to as crystallosapiens and elastosapiens.

The ancestor of the first branch can be considered the mineralogist Ren Just Hay, the father of geometric crystallography in the 18th century, who observed the features of the cleavage of crystals such as calcite and deduced that they were made up of small orderly stacks, which he named integrant molecules. The concept of atomic lattice dates back to the 19th century, when it was introduced by Gabriel Delafosse, one of Hays disciples. Shortly afterwards, Auguste Bravais formulated the hypothesis of a lattice structure of crystals based on the principles of geometry, according to which he listed 14 different types of crystal lattices that accounted for crystal anisotropy and symmetry properties. Finally, at the beginning of the 20th century, Max von Laue used X-ray diffraction to experimentally confirm Bravais works.

As for the second branch, its forerunner is beyond any doubt Robert Hooke, who in the 17th century established the linear relation between force and displacement in a loaded spring. Then, the notions of stress and deformation were formulated by Thomas Young at the beginning of the 19th century. Soon afterwards, Augustin-Louis Cauchy established the 3D formalism for the generalized Hookes law using the notion of elastic constants and tensor calculus applied to the mechanics of continuous media.

As this brief history indicates, by the beginning of the 20th century, the conditions were met for a wide and varied descent.

First of all, Clarence Zener rigorously quantified the elastic anisotropy of cubic symmetry, a concept that will be constantly revisited in this part of the book. For other symmetries, different approaches were also proposed.

Experimental characterization also improved. While simple quasi-static tests, such as the tensile test, were previously used to characterize elasticity, dedicated physical methods involving volume or surface elastic waves were used for more precise measurements of macroscopic quantities: ultrasounds, the dynamic resonant method or Brillouin scattering.

Due to very strong development, the mechanics of heterogeneous continuous media could transition from the elasticity of the monocrystal and its constants to the elasticity of aggregates (poly crystals). The historical mean-field models elaborated by Woldemar Voigt and Adolph Reuss were the basis for the exploration of numerous approaches. Finally, the recent numerical progresses allowed the optimization of the whole-field methods.

Finally, the recent emergence of nanostructures required a zooming in on interatomic interactions in order to describe fine-scale elasticity or to predict the properties of massive materials using dynamic molecular methods.

These approaches at various scales, as well as the amount of experimental and theoretical data or data resulting from simulations involving many categories of materials, make it impossible to draw an overall view that describes crystal elasticity in a simple manner.

This book proposes a phenomenological approach that addresses this problem: at the macroscopic scale, anisotropy and a dimensionless representation can be used to simplify the mathematical formulation of the classical crystal elasticity, which is reviewed in presents this transition that involves the writing of a new Hookes law (which we could call nano-Hookes law), which deals with spatial rigidity at the atomic level.

A second-scale transition is then approached, which passes from monocrystal elasticity to polycrystal elasticity. is dedicated to this subject. First, the historical cases of mean-field homogenization are approached, and then a much simplified homogenization is proposed, which allows for the phenomenological classification of these more or less various complex approaches found in the literature. It will be noted that anisotropy remains an essential parameter for comparing the elasticity of polycrystals and mechanical approaches.

Finally, is dedicated to illustrating specific cases of crystal elasticity using the data obtained using a specific high-performance vibration method. The description of this method will be followed by multiple examples of the evolution of elasticity in relation to the structural or physical aspects of functional materials.

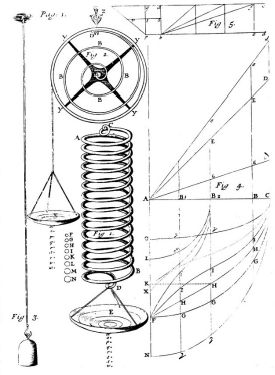
Robert Hookes illustration of the elasticity of metals (De potentia restitutiva, 1678)

The second part of this book describes a Lagrangian approach to vibrations. The aim is to propose a unique way to describe simple geometry vibrations.

While a numerical calculation is essential for predicting the vibrations of complex structures under widely variable loadings based on the elasticity data of the materials composing these structures, a reverse approach is proposed here: based on the vibrations of structures and the simplest possible loads (plate torsion, beam and plate bending, tractioncompression on a cylindrical rod), detected resonance frequencies can be used to retrieve elasticity data on the materials, whether crystalline or not. Four chapters are dedicated to this subject.

Furthermore, a high-performance experiment must be set up, as described in the first part, and a proper formalism should be proposed to connect elasticity and vibrations. Various approaches developed in the 20th century can be found in the literature, but this part presents a unique approach that best addresses the problem. It involves writing the Lagrangian of a dynamic system and applying the Hamilton principle to minimize the energy.