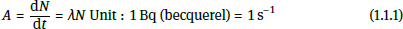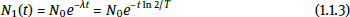Bo N. Nilsson - Exercises with Solutions in Radiation Physics

Bo Nilsson

Exercises with Solutions in Radiation Physics

Bo Nilsson

Managing Editor: Paulina Lena-Szreter

Language Editor:xs Andrew Laister

Published by De Gruyter Open Ltd, Warsaw/Berlin
Part of Walter de Gruyter GmbH, Berlin/Munich/Boston

This work is licensed under the Creative Commons Attribution-NonCommercial-NoDerivs 3.0 license, which means that the text may be used for non-commercial purposes, provided credit is given to the author. For details go to http://creativecommons.org/licenses/by-nc-nd/3.0/.

Copyright 2015 Bo Nilsson
published by De Gruyter Open

ISBN 978-3-11-044205-2
e-ISBN 978-3-11-044206-9

Bibliographic information published by the Deutsche Nationalbibliothek
The Deutsche Nationalbibliothek lists this publication in the Deutsche Nationalbibliografie; detailed bibliographic data are available in the Internet at http://dnb.dnb.de.

www.degruyteropen.com

Cover illustration: iStock

This material is intended for use in courses in radiation physics. Many textbooks include exercises, but not often full solutions, and they often refer to specific material in the textbook. This material can be used in many courses, often included in a medical physics graduate program, independent of a specific textbook. The material consists of six chapters covering the basic fundamental radiation physics, but not the more specific clinical applications where there is a rapid change and in which the exercises may be obsolete after some time. The first chapter includes exercises related to radioactive sources and decay schemes. This is followed by a chapter covering the interaction of ionizing radiation, including photons and charged particles. The text then continues with a chapter on detectors and measurements including both some simple counting statistics and properties of detectors. The next chapter is dedicated to dosimetry, which is a major subject in medical physics. A short chapter is covering radiobiology, where there is a focus on different cell survival models. The last chapter is dealing with radiation protection and health physics. Both radiation shielding calculations and radioecology are covered. The exercises in the material have been used in the education for medical physicists in Stockholm, Sweden, and the order of chapters follows the order of courses in this education, but hopefully they are useful in all applications of radiation physics including also health physics. Some problems are probably similar to what can be found in other material as there are some items that are always important to include in the courses and when producing exercises it is easy to forget where any idea is coming from.

The student is supposed to have a background in mathematics and physics corresponding to a BSc in physics. The mathematics involved is mainly straightforward and includes only basic integrals and differential equations. Each chapter starts with a small refreshment of important definitions and relations that are useful for the chapter. The material is not aimed to be a textbook and for a deeper knowledge and understanding the reader is referred to ordinary textbooks, some of which are listed in the bibliography. The chapter then continues with a section of exercises followed by a section with solutions. The reader is recommended to make an effort to understand the exercise and try to solve it before checking the proposed solution. Most exercises are numerical and a numerical answer is expected. Some exercises are more intended for a discussion where probably different answers can be acceptable. In order to solve the exercise and obtain a numerical value, data like decay constants, interaction coefficients etc are often needed. They are normally not included in the exercise, but the reader is expected to understand which data are needed and then find them in relevant tabulated material. Much of the information is obtained at different sites on the Internet, and some sites are listed in the bibliography. However, some data may be difficult to find, and for those are tables included in this material. The reference list at the end of the book is divided in two sections, one including tables and sites that could be useful when solving the exercises and one including some typical text-books for the different courses related to the different chapters. This list is far from complete, but gives just some examples of books used by education establishments in Stockholm. There is also after some chapters, specific references to papers mentioned in the chapter.

Many of these exercises have been used during several years in the education for hospital physics in Stockholm. However, in spite of this, there are probably both typing mistakes or badly explained solutions. I am of course grateful if these mistakes are reported to me.

Finally, I would like to acknowledge help from my colleagues at the department, Prof. Irena Gudowska, ass Prof, Albert Siegbahn, and ass Prof. Iuliana Toma-Dasu; who have encouraged me to make this collection of exercises and also have pointed out several mistakes in the material. Without their contribution the mistakes would be even more abundant. I also want to thank all students that have been working with the problems during several years.

Stockholm, June 2014

Bo Nilsson

Activity is defined as

where A is activity, dN/dt is the number of spontaneous nuclear transformations, dN, from a particular energy state in a time interval dt. is the decay constant (s1) and N is the number of radioactive nuclei.

The specific activity is defined as the activity of a certain radionuclide per mass unit (Bq kg1).

A radionuclide decays according to the equation

where N1(t) is the number of radioactive nuclides after a time t, N0 = N1(0) is the number of radioactive nuclides at time 0 and T is the half-life (T = In 2/).

The equation may also be expressed as

Sometimes the daughter nuclides are also radioactive and a chain of radioactive nuclides is obtained. A general solution for the activity of a radionuclide in the chain is given by the Bateman equations. In this compilation only the first three radionuclides in the chain will be treated.