Fundamental Chess Openings
Paul van der Sterren

Contents
Symbols and Notation
| x | capture |
| + | check |
| ++ | double check |
| # | checkmate |
| !! | brilliant move |
| ! | good move |
| !? | interesting move |
| ?! | dubious move |
| ? | bad move |
| ?? | blunder |
| 0-0 | castles kingside |
| 0-0-0 | castles queenside |
| (D) | see next diagram |
| K | king |
| Q | queen |
| R | rook |
| B | bishop |
| N | knight |
Algebraic Notation
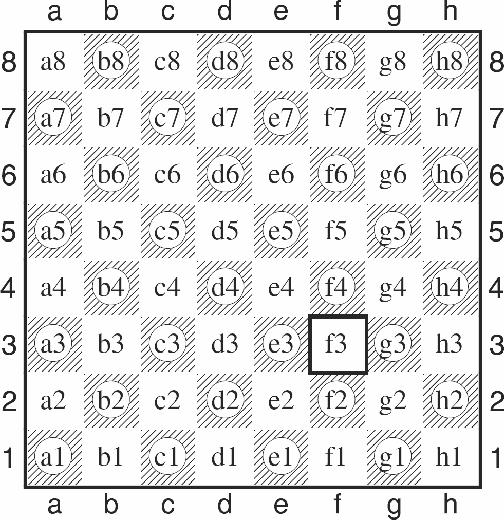
Moves are shown by giving the piece symbol followed by the destination square. For instance, if a knight moves to the square marked in the diagram, this is written as Nf3 in algebraic notation. If two pieces of the same type can move to the same square, more information is given. For instance, if there are knights on g1 and e5, then the one on e5 moving to f3 is written as Nef3.
For pawn moves, only the arrival square is given (for example, e4 means that a pawn moves to the e4-square). For pawn captures, the file the pawn is leaving is also given. Thus exf4 means that a pawn on the e-file makes a capture that brings it to the f4-square.
Publishers Note on the Kindle Edition
The printed edition of Fundamental Chess Openings is a large-format 480-page tome. With plenty of diagrams on each double-page spread, it is always easy to know what position is being discussed, and since the book lies nicely flat, it is a simple matter to turn to a different page to check a cross-reference.
For this Kindle edition, it was a priority to retain the ease of use while offering the additional conveniences of the electronic format. We have almost doubled the number of diagrams to make sure that there is always one close at hand, even when reading on a device with a small screen. Plentiful links in the text make it easy to refer to relevant cross-references, especially if using a touch-screen device.
I would particularly like to draw your attention to the two indexes, as these are a great way to navigate to specific lines. If you know either the , you will quickly be reading Van der Sterrens commentary on that system. The Index of Variations provides a skeleton view of the whole book.
For links within the text, the following convention is used: a cross-reference to a different section (for a transposition or a relevant comparison) is shown using the word link. A signpost indicating that the current section splits to cover a number of subvariations takes the form of underlined moves or variation names.
Fundamental Chess Openings was published in 2009. The nature of the book ensures that it will hardly date as the years pass, but the opportunity has been taken to correct a few minor errors and, e.g., to indicate if certain lines have gone out of fashion or exploded in popularity over the last four years, and notable new ideas that lie behind these changes.
It is my sincere hope that this edition proves as useful and popular as the print edition.
Graham Burgess
Woodbury, Minnesota
November 2012
Introduction
In the colossal body of chess literature, no aspect of the game has been treated as extensively as the openings. In varying degrees of expertise, clarity and depth, thousands of books discuss every imaginable and unimaginable opening the game of chess has to offer. This is a process that will never stop. As long as a particular opening is being played, its variations will be worked out deeper and deeper and assessments will be modified on the basis of these new experiences. As long as chess is alive, its opening theory will also be alive and new books will be needed to document all of this new life.
This book intends to introduce the reader to this strange but fascinating world, the world of opening theory. There will be no long sequences of moves, no complicated analysis and no real attempt to keep up with the very latest developments. Instead I shall attempt to clarify the background, the genesis and the development of all major openings and try to show how they are much more interconnected and based on the same ideas and insights than many people think. This approach makes this book a very different one from the usual opening manuals. It could perhaps be said to precede them. If it has the effect on the reader that it whets his appetite for these usual opening books, or at least makes him understand them a little bit better, this book will have fulfilled its purpose.
What is Opening Theory?
Everyone who devotes even the tiniest amount of thought to his first move not only makes a start with that particular game but also with the development of opening theory. From that moment on, every new game will confront him with the starting position again and therefore with his earlier thoughts on it. Also he will sooner or later find out that millions of other players have pondered exactly the same problems and, whether he wants to or not, he will to some extent start comparing his own ideas about how to start a game with theirs.
This means that opening theory arises quite naturally with the start of a game. No one can avoid it. It ends, equally naturally, with the end of a game. If we pursue our thinking about the opening position logically and systematically, while accepting only the highest possible degree of certainty as a satisfactory result, we cannot end our investigation unless we are sure we have reached either a winning or a drawn position. Seen in this light, thinking about the starting position involves a thorough examination of the middlegame and endgame as well.
It could be said then, that opening theory does not really exist, at least not as something separate from other aspects of the game. Ultimately, opening theory comprises all theory.
However, since the human brain and even the computer is still not capable of completely seeing through (and thereby destroying) chess as a whole, in practice opening theory does not end with an empty board but in positions where there is a certain consensus about how they should be assessed, for instance chances are equal or White (or Black) has the advantage.
Sometimes a question can be answered with total confidence. In the position after 1 e4 there is some room for discussion on how good or bad 1...g5 is (though not much), but if White continues 2 d4 here, there can be no question on the value of 2...f6 because 3 Qh5# is then mate. End of game, end of theory.
But in most cases an assessment is merely a temporary stop. The moment somebody starts questioning it, the argument continues. Until the next temporary stop is reached.
And so, ever since the beginnings of chess, every single chess-player has contributed something to that gigantic construction called opening theory. This brings us to the next question.
How Much Theory Should a Player Know?
The most severe answer to this has to be everything, the softest as much as you like and the profoundest nothing. All three are correct.
Knowledge of opening theory is a double-edged sword. The player who knows a lot will undoubtedly profit by his knowledge, but he may also live in constant fear of meeting an opponent who knows even more. Everyone who has studied opening theory in depth will have learned that, no matter how well you do your work, there is always the possibility of having overlooked something or of not having looked deep enough. Trying to keep abreast of the latest developments, reading everything, keeping a close watch on the Internet, makes you very knowledgeable but also acutely aware of the possibility of missing something. In short, he who lives by the sword shall die by the sword.















