Adams - Zombies & calculus

Zombies & Calculus

Zombies & Calculus

Colin Adams

PRINCETON UNIVERSITY PRESS
PRINCETON AND OXFORD

Copyright 2014 by Princeton University Press

Published by Princeton University Press, 41 William Street, Princeton, New Jersey 08540

In the United Kingdom: Princeton University Press, 6 Oxford Street, Woodstock, Oxfordshire OX20 1TW

press.princeton.edu

Jacket image Jeff Thrower/Shutterstock.

Jacket design by Lorraine Donneker.

All Rights Reserved

Library of Congress Cataloging-in-Publication Data

Adams, Colin Conrad.

Zombies and calculus / Colin Adams.

pages cm

Summary: How can calculus help you survive the zombie apocalypse? Colin Adams, humor columnist for the Mathematical Intelligencer and one of todays most outlandish and entertaining popular math writers, demonstrates how in this zombie adventure novel. Zombies and Calculus is the account of

Craig Williams, a math professor at a small liberal arts college in New England, who, in the middle of a calculus class, finds himself suddenly confronted by a late-arriving student whose hunger is not for knowledge. As the zombie virus spreads and civilization crumbles, Williams uses calculus to help his small band of survivors defeat the hordes of the undead. Along the way, readers learn how to avoid being eaten by taking advantage of the fact that zombies always point their tangent vector toward their target, and how to use exponential growth to determine the rate at which the virus is spreading. Williams also covers topics such as logistic growth, gravitational acceleration, predator-prey models, pursuit problems, the physics of combat, and more. With the aid of his story, you too can survive the zombie onslaught. Featuring easy-to-use appendixes that explain the mathematics necessary to enjoy the book, Zombies and Calculus is suitable for recent converts to calculus as well as more advanced readers familiar with multivariable calculusProvided by publisher.

Includes bibliographical references and index.

ISBN 978-0-691-16190-7 (hardback)

1. ZombiesFiction. 2. CalculusFiction. 3. MathematicsFiction. I. Title.

PS3601.D3698Z34 2014

813.6dc23

2014012448

British Library Cataloging-in-Publication Data is available

This book has been composed in Minion Pro

Printed on acid-free paper.

Typeset by S R Nova Pvt Ltd, Bangalore, India

Printed in the United States of America

1 3 5 7 9 10 8 6 4 2

Contents

Zombies & Calculus

Introduction

L et me just start this off by saying if you are squeamish, you should not read this book. Of course, theres little likelihood that you are squeamish, considering that you are even reading this at all. The squeamish amongst us, especially those too squeamish to behead a zombie, have long ago been infected and joined the zombie hordes. So if you are reading this, squeamishness is most likely the least of your problems.

This book is my attempt to write down how calculus has helped me to stay alive this long. I am writing this approximately three months after the first incidents of infection occurred. You may be reading this much later than that, and if so, congratulations on still being around. But for me, surviving three months has been a huge victory over immense odds. And honestly, I would not be here today if it were not for calculus. Maybe it will help you to stay alive a little longer, too.

This book is aimed at survivors who have seen some calculus already. I cant teach you all of calculus. That takes a couple of years of work. If you have never seen calculus, you can still read the book, and look at the last appendix, which gives background necessary to understand much of the math in this book, and you will be a better person for it. Perhaps, it will motivate you to study calculus. Of course, these days, trying to stay alive is a full-time job. Its unlikely you will find the time to study calculus as well.

The primary audience for this book is those survivors who learned calculus before the apocalypse. If you have had a year of calculus, thats great. Multivariable calculus is even better, but if you havent seen that, Ill explain that material as we go along.

Sometimes the conversations I recorded here become more technical. When that is the case, I have moved the more sophisticated parts of the conversation to the first appendix. The places where this occurs are marked by a bloody handprint and a boldface roman numeral, like (I). If you relish the technical stuff, just jump to the appendix and read on as if the text there continues what was being discussed when you encountered the roman numeral, returning to the main text after finishing that section of the appendix. If you dont like technical stuff, just ignore the roman numerals and keep reading straight through the text. Both ways work just fine.

The second appendix is a conversational review of all of calculus, focussing on the aspects that are relevant to this story. If you need some help with the mathematics when it appears in the book, take a look there. It should help.

Life is not what it once was. I have seen things and done things that I would not have believed possible three months ago. But Im still here, and somehow it makes me feel incrementally better to write this down, to feel like Im helping others by doing so. This is my story, and in it, I will tell you how you can use calculus to help you stay alive. Good luck to you, and good luck to all of us that remain.

chapter one

HOUR 6

Up until April of this year, I was a math professor at Roberts College, in the tiny town of Westbridge, Massachusetts, on the far western border of the state. Roberts was a small liberal arts college, endowed with excellent students, a beautiful New England campus, and deep pockets. I couldnt imagine a better job than teaching those highly motivated students the mathematics that I love, and also having the time to work on my own research in mathematics. It was a dream job. On top of that, Roberts was set in the Berkshire Mountains, which by anyone elses standards are not mountains at all, but they are still some of the prettiest hills you have ever seen.

Spring break ended at the beginning of April, and we had just returned for the last six weeks of the semester. It was a Friday, and I was reviewing for my calculus class the fact the derivative f(x) of a function f(x) is the slope of the tangent line to the graph of the function at x. I had drawn this picture () on the board.

Megan, one of those students who always sits in the first row, and who really has a true appreciation for the beauty of mathematics, had asked the perfect set-up question.

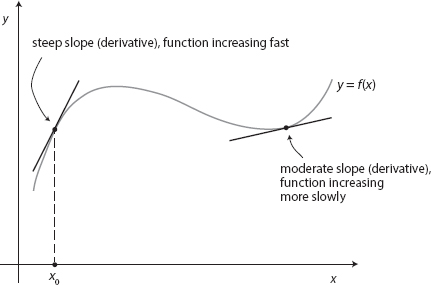
Figure 1.1: The derivative is the slope of the tangent line.

But Professor Williams, what if the graph isnt smooth? What if it has a kink? How do you know which tangent line to use?

I smiled. Great question, Megan. Im glad you asked. She returned the smile, pleased with the compliment. Megan tended to dress on the conservative side, and that day, she was wearing a tan cashmere sweater over a white blouse, with pearls and a matching tan skirt. Thora, who was sitting just behind Megan, and who had chopped purple hair and more piercings than I could easily count, gave a barely audible groan.