Miller - Bob Millers math for the new GRE

I have many people to thank.

I thank my wife, Marlene, who makes life worth living, who is truly the wind under my wings.

I thank the rest of my family: children Sheryl and Eric and their spouses Glenn and Wanda (who are also like my children); grandchildren Kira, Evan, Sean, Sarah, Ethan, and Noah; my brother Jerry, and my parents, Cele and Lee, and my in-law parents, Edith and Siebeth.

I thank Larry Kling, Michael Reynolds, and Mel Friedman for making this book possible.

I thank Martin Levine for making my whole writing career possible.

I have been negligent in thanking my great math teachers of the past. I thank Mr. Douglas Heagle, Mr. Alexander Lasaka, Mr. Joseph Joerg, and Ms. Arloeen Griswold, the best math teacher I ever had, of George W. Hewlett High School; Ms. Helen Bowker of Woodmere Junior High; and Professor Pinchus Mendelssohn and Professor George Bachman of Polytechnic University. The death of Professor Bachman was an extraordinary loss to our country. In a country that produces too few advanced degrees in math, every year two or three of his students would receive a Ph.D. in math, with more receiving their M.S. His teaching and writings were clear and memorable. He wrote four books and numerous papers on subjects that had never been written about or had been written so poorly that nobody could understand the material.

As usual, the last three thanks go to three terrific people: a great friend, Gary Pitkofsky; another terrific friend and fellow lecturer, David Schwinger; and my cousin, Keith Robin Ellis, the sharer of our dreams.

Bob Millers Math for the GMAT, Third Edition
Bob Millers Math for the Accuplacer
Bob Millers Math for the ACT
Bob Millers Math for the TABE
Bob Millers Basic Math and Pre-Algebra for the Clueless, Second Edition
Bob Millers Algebra for the Clueless, Second Edition
Bob Millers Geometry for the Clueless, Second Edition
Bob Millers Math SAT for the Clueless, Second Edition
Bob Millers Pre-Calc with Trig for the Clueless, Third Edition
Bob Millers High School Calc for the Clueless
Bob Millers Calc 1 for the Clueless, Second Edition
Bob Millers Calc 2 for the Clueless, Second Edition
Bob Millers Calc 3 for the Clueless

EPILOGUE

If you feel more is needed, you may need a little more for your confidence. But if our journey together has been complete, you have all you need for success.

Again, congratulations. Now that youve finished the book, you should review any section that has caused you difficulty.

Remember, again, that you do not need a perfect score. You need only a score that will get you into the graduate school of your choice.

Good luck with the rest of your college education and your chosen career.

Also Available from REA...

Research & Education Association

For more information, visit us online at: www.rea.com/GRE

Also Available from REA...

Research & Education Association

For more information, visit us online at: www.rea.com/GRE

CHAPTER 1:

The Basics

All math begins with whole numbers. Master them and you will begin to speak the language of math.

Lets begin at the beginning. The GRE works only with real numbers , numbers that can be written as decimals. However, it does not always say numbers. Lets get specific.

NUMBERS

Whole numbers: 0, 1, 2, 3, 4,...

Integers: 0, 1, 2, 3, 4,..., where 3 stands for both +3 and 3.

Positive integers are integers that are greater than 0. In symbols, x > 0, x is an integer.

Negative integers are integers that are less than 0. In symbols, x < 0, x is an integer.

Even integers: 0, 2, 4, 6, ...

Odd integers: 1, 3, 5, 7, ...

Inequalities

For any numbers represented by a , b , c , or d on the number line:

We say c > d ( c is greater than d ) if c is to the right of d on the number line.

We say d < c ( d is less than c ) if d is to the left of c on the number line.

c > d is equivalent to d < c .

a b means a < b or a = b ; likewise, a > b means a > b or a = b .

Example 1:

Tell whether the following inequalities are true:

1. 4 7
2. 9 9
3. 7 2.

Solution:

1. 4 7 is true, since 4 < 7;
2. 9 9 is true, since 9 = 9;
3. 7 2 is false, since 7 > 2.

Example 2:

Graph all integers between 4 and 5.

Solution:

Notice that the word between does not include the endpoints.

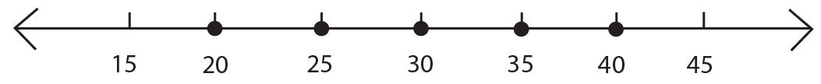
Example 3:

Graph all the multiples of 5 between 20 and 40 inclusive.

Solution:

Notice that inclusive means to include the endpoints.

Odd and Even Numbers

Here are some facts about odd and even integers that you should know.

• The sum of two even integers is even.
• The sum of two odd integers is even.
• The sum of an even integer and an odd integer is odd.
• The product of two even integers is even.
• The product of two odd integers is odd.
• The product of an even integer and an odd integer is even.
• If n is even, n 2 is even. If n 2 is even and n is an integer, then n is even.
• If n is odd, n 2 is odd. If n 2 is odd and n is an integer, then n is odd.

OPERATIONS ON NUMBERS

Product is the answer in multiplication; quotient is the answer in division; sum is the answer in addition; and difference is the answer in subtraction.

Since 3 4 = 12, 3 and 4 are said to be factors or divisors of 12, and 12 is both a multiple of 3 and a multiple of 4.