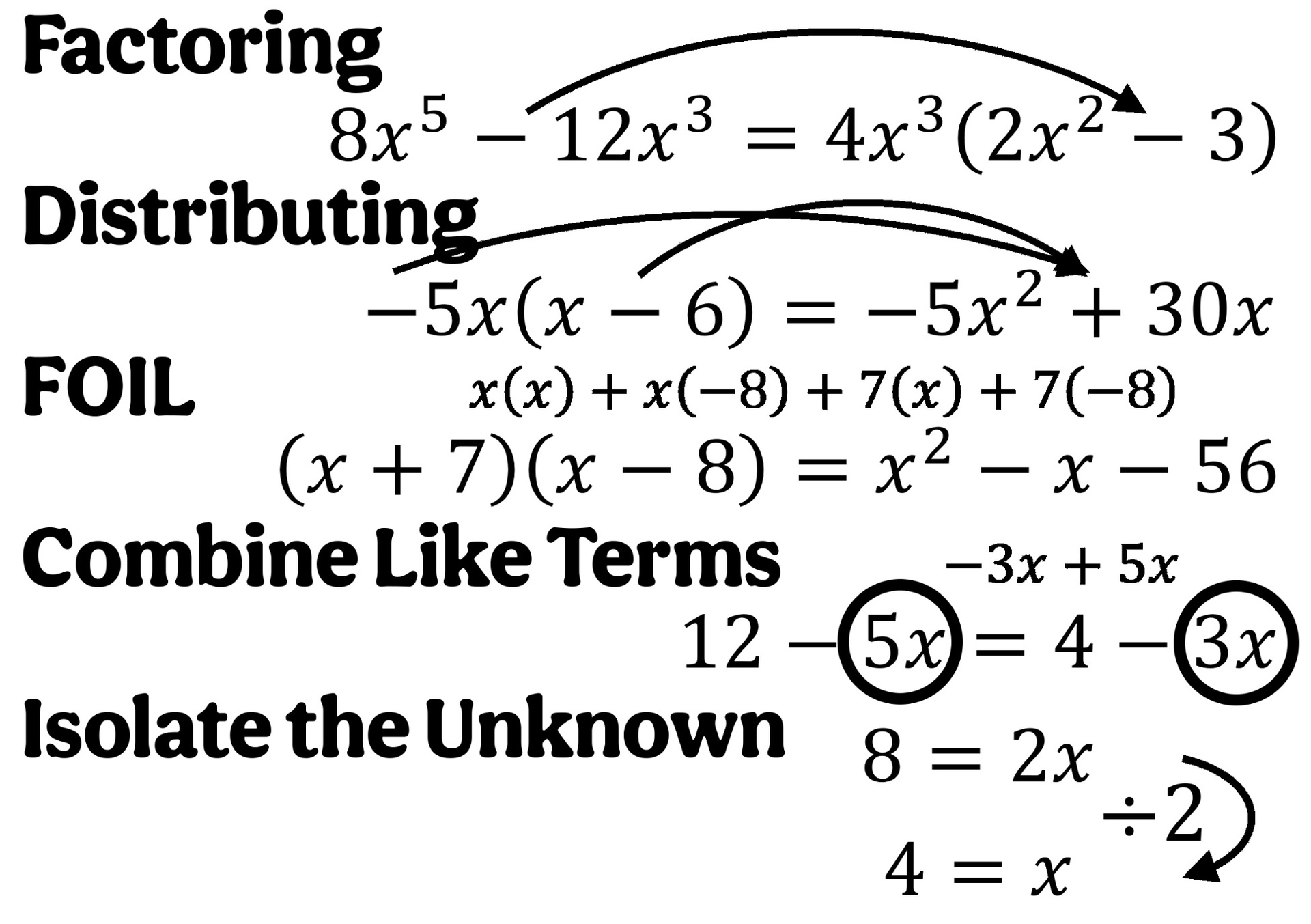McMullen - Beginning Algebra Skills Practice Workbook: Factoring, Distributing, FOIL, Combine Like Terms, Isolate the Unknown

Beginning Algebra Skills Practice Workbook: Factoring, Distributing, FOIL, Combine Like Terms, Isolate the Unknown — read online for free the complete book (whole text) full work

Below is the text of the book, divided by pages. System saving the place of the last page read, allows you to conveniently read the book "Beginning Algebra Skills Practice Workbook: Factoring, Distributing, FOIL, Combine Like Terms, Isolate the Unknown" online for free, without having to search again every time where you left off. Put a bookmark, and you can go to the page where you finished reading at any time.

Light

Font size:

↓

↑

Georgia Tahoma Arial Verdana

Reset

Interval:

↓

↑

Bookmark:

Make

1234567...20

BEGINNING ALGEBRA

Skills Practice Workbook

with Answers

Chris McMullen, Ph.D.

Copyright 2021 Chris McMullen, Ph.D.

monkeyphysicsblog.wordpress.com

improveyourmathfluency.com

chrismcmullen.com

All rights reserved.

Zishka Publishing

ISBN: 978-1-941691-91-5

Mathematics > Prealgebra

Mathematics > Algebra

CONTENTS

Introduction

The goal of this workbook is to help students develop fluency in specific beginning algebra skills. Most algebra students would benefit from additional practice with these skills.

Combine like terms: This vital skill is used for almost every topic in algebra. It is used to simplify expressions and to solve equations for unknowns.

Multiply variables with exponents: When x m multiplies x n , the exponents are added together to make x m+n . This fundamental concept is applied when two expressions are multiplied. For example,

(3x + 4)(2x 5)

involves x x = x .

Distribute: According to the distributive property of arithmetic,

a(b + c) = ab + ac.

This property also applies to algebra. For example,

x(x + 3) = x(x) + x(3) = x + 3x.

Distribute minus signs: The distributive property applies to negative numbers, too. For example,

(x 8) = 1(x) 1(8) = x + 8.

Here, the two minus signs make a plus sign in the second term.

Factor: When the distributive property is applied in reverse, it is called factoring. For example,

15x + 20x = 5x (3x + 4).

The greatest common factor (GCF) of 15 and 20 equals 5, and the highest power of x that is common to x and x is x .

The FOIL method: This valuable skill allows students to multiply two expression of the form

(6x 5)(2x + 7).

FOIL is an abbreviation for first, outside, inside, last.

Square of the sum: An expression of the form

(x + y)

equals

x + 2xy + y .

Knowing this formula often comes in handy.

Difference of squares: An expression of the form

(x + y)(x y)

equals

x y .

It is often useful to factor expressions of the form

x y

as

(x + y)(x y).

Isolate the unknown: A fundamental way to solve a large class of algebraic equations is to first combine like terms and then isolate the unknown by applying one operation at a time to both sides of the equation.

Factor quadratic expressions: Some expressions of the form

x + bx + c

can be put in the form

(x + d)(x + e)

without using irrational numbers or fractions. Knowing how to do this can save valuable time.

Answer key. Practice makes permanent, but not necessarily perfect. Check the answers at the back of the book and strive to learn from any mistakes. This will help to ensure that practice makes perfect.

1 Combine Like Terms

A variable is a letter like x or y that represents an unknown number. The reason for calling it a variable is that it equals different values in different problems. For example, x might turn out to equal 3 in one problem and x might turn out to equal 8 in another problem. The value of x varies from problem to problem.

An equation has an equal sign, whereas an expression does NOT have an equal sign. For example,

6x 9 = 15

is an equation, whereas

2x 3x + 5

is an expression. This book begins by working with expressions. Later chapters will work with equations.

A coefficient is a number that multiplies a variable. For example, 6 is the coefficient in the expression 6x while 4 is the coefficient in 4x.

The terms of an expression are separated by plus and minus signs. For example, the expression

4x + 6x 3x + 5

has four terms, starting with 4x and ending with 5.

When two terms have the same variable and the same exponent, like 5x and 3x , the terms are considered to be like terms . Two constant terms like 7 and 4 (which do NOT have any variable) are also considered to be like terms . If the exponents are different, the terms are unlike . For example, 2x and 6x are unlike terms because in one case the variable is squared, but in the other case the variable is not squared.

If two terms are like terms, they can be combined together by adding or subtracting the coefficients. For example,

5x + 3x = 8x .

This only works for like terms.

Chapter 1 Examples

Example 1 . Simplify 6x + 9 + 3x 5.

Identify the like terms:

6x + 9 + 3x 5 = (6x + 3x) + (9 5) = 9x + 4

Note: It is not really necessary to write the middle step above that has parentheses. We just wrote this step to help show which terms are like terms. The solution could be written more concisely as

6x + 9 + 3x 5 = 9x + 4.

Example 2 . Simplify 8x 6x 3x + 4x.

Identify the like terms:

8x 6x 3x + 4x

= (8x 3x ) + (6x + 4x)

= 5x 2x

Note: 6 + 4 = 2 because 6 is farther from zero than 4.

Example 3 . Simplify x + 5 3x .

Identify the like terms:

x + 5 3x

= (x 3x ) + 5

= 2x + 5

Notes: The constant term, 5, does not have any like terms to combine with. When no coefficient is present before a variable, as in x , the coefficient equals 1. For example, x = 1x . Therefore,

x 3x = 1x 3x = 2x .

Note that 1 3 = 2 because 3 is farther from zero than 1.

Example 4 . Simplify 8x 3 + 4x 5 5x 7.

Identify the like terms:

8x 3 + 4x 5 5x 7

= (8x + 4x 5x) + (3 5 7)

= 7x 15

Example 5 . Simplify 4x + 3x 6 + x 3x 2.

Identify the like terms:

4x + 3x 6 + x 3x 2

= (3x + x ) + (4x 3x) + (6 2)

= 4x + x 8

Notes: 4x 3x = 1x = x. When the coefficient of a variable is 1, the coefficient is not written. It is customary to order the terms from highest power to lowest power.

Chapter 1 Problems

Directions : Combine like terms to simplify each expression.

Problem 1. Simplify 4x + 5 + 11x + 3.

Problem 2. Simplify 2x + 16x + x 6x.

Problem 3. Simplify 17x 10x 7 + 6.

Problem 4. Simplify 3x + 9 4x.

Problem 5. Simplify x + 7 + x + 8.

Problem 6. Simplify 2x + 36x 26x.

Problem 7. Simplify 12x 3 + 6x 14.

Problem 8. Simplify 7x + 4x 6x .

Problem 9. Simplify 27x + 5x + 11x + 8x .

Problem 10. Simplify 6x + 3 + x 7 + 2x.

Problem 11. Simplify 13 9x + 6.

Problem 12. Simplify 5x 8x 4x .

Problem 13. Simplify 17x 14x 12x 14x.

Problem 14. Simplify 16 + 13x 17 22x 6.

Problem 15. Simplify 25x + 28x 21x 15x .

Problem 16. Simplify 8x 17 + 4x 10.

Problem 17. Simplify 14 + 22x 19 + 21x .

Problem 18. Simplify 6x 4x + 9x x.

Problem 19. Simplify 25x + 5x + 50x 6x .

Problem 20. Simplify 3x 5 8x + 4 7x .

Problem 21. Simplify 9x 2x + 4x + 10x .

Problem 22. Simplify 6x + 12 4 x 9.

Problem 23. Simplify 19x + 13x 2x 3x .

Problem 24. Simplify 15x + 21 12x + 4.

Problem 25. Simplify 4x 9x + 13 + 7x + 18.

Problem 26. Simplify 6x 5x + 2x 2x .

Problem 27. Simplify 41x + 10x + 33x + 9x + 8x.

Problem 28. Simplify 26 9x 26 4x .

Next page

1234567...20

Light

Font size:

↓

↑

Georgia Tahoma Arial Verdana

Reset

Interval:

↓

↑

Bookmark:

Make

Similar books «Beginning Algebra Skills Practice Workbook: Factoring, Distributing, FOIL, Combine Like Terms, Isolate the Unknown»

Look at similar books to Beginning Algebra Skills Practice Workbook: Factoring, Distributing, FOIL, Combine Like Terms, Isolate the Unknown. We have selected literature similar in name and meaning in the hope of providing readers with more options to find new, interesting, not yet read works.

Carolyn Wheater

Practice Makes Perfect: Algebra I Review and Workbook, Third Edition

Christopher Monahan

Practice Makes Perfect: Algebra II Review and Workbook, Third Edition

Najwa Hirn

Algebra Workbook Adding Subtracting Real Numbers

Chris McMullen

Essential Prealgebra Skills Practice Workbook

Chris McMullen

Trig Identities Practice Workbook with Answers: 10 (Improve Your Math Fluency)

Mary Jane Sterling

Algebra I Workbook for Dummies

Chris McMullen

Geometry Proofs Essential Practice Problems Workbook with Full Solutions (Improve Your Math Fluency)

Chris McMullen

Fractions Essentials Workbook with Answers (Improve Your Math Fluency)

Chris McMullen

Algebra Word Problems Practice Workbook with Full Solutions

Sterling

Algebra I Workbook For Dummies

Wheater

Practice Makes Perfect Algebra I Review and Workbook

McMullen

Calculus: Essential calculus skills practice workbook with full solutions

Discussion, reviews of the book Beginning Algebra Skills Practice Workbook: Factoring, Distributing, FOIL, Combine Like Terms, Isolate the Unknown and just readers' own opinions. Leave your comments, write what you think about the work, its meaning or the main characters. Specify what exactly you liked and what you didn't like, and why you think so.