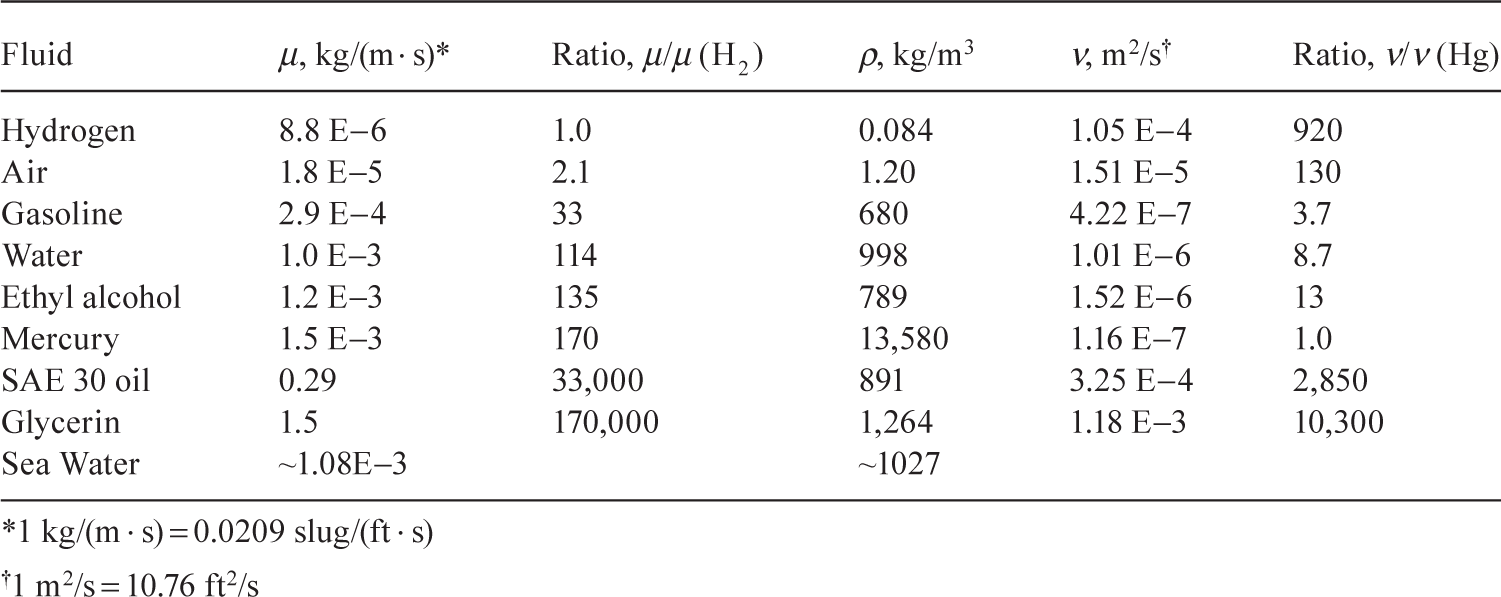
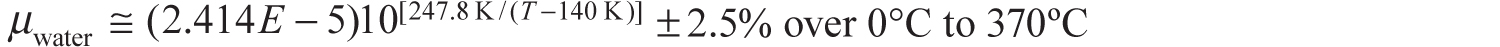A Brief History of Dimensional Analysis
This book is an introduction to a powerful tool known as dimensional analysis. The method is also termed similarity analysis, and the concept referred to as similitude. Dimensional analysis can be used to find patterns within and succinct explanations for physical phenomena. It is used to find functional relations that are independent of whether we have a large amount of each quantity or a small amount. It is a search for laws that are independent of measurement systems.
The history of dimensional analysis is linked with the history of science starting as early as the great English mathematician and physicist Sir Isaac Newton. Histories and developments of the subject are provided by Macagno (1971), Rott (1992), White (2016), Taylor et al. (2007), and Gibbings (2011), and only some material is summarized here.
In 1687, Isaac Newton described some rules of dimensional relations and analyses in the Principia. This included similarity in the trajectory shapes of bodies in motion, and indeed his theories of motion present a precise set of mathematical relations between the quantities of position, velocity, acceleration, momentum, and force. In around 1736, Swiss mathematician and physicist Leonhard Euler wrote extensive descriptions of units and dimensional reasoning, including the dimensions associated with Newtons second law, and so-called unit conversion factorsconcepts essential to consistent descriptions of the physical world.
In 1822, Jean-Baptiste Joseph Fourier clarified greatly the concept of physical dimensions, introduced the concept of dimensional homogeneity in mathematical relations, pointed out that equations describing physical phenomena are independent of the choice of system of units, and applied similarity to describe and unify heat flow. Lord Rayleigh (b. 1842) provided perhaps the most influential breakthroughs in dimensional analysis in studies of hydrodynamic drag, flow stability, sound waves, and light scatter from particles. He also strongly advocated dimensional analysis. He demonstrated that the principle of similitude yields elegant scaling laws and demonstrated the method using examples from Newtons law of gravitation, drag on spheres, heat flow in thin fins, vibrating drops, and the vibration of Aeolian harp strings. Rayleigh argued in favor of the importance and appropriate use of the method (e.g., as an initial analysis of a problem) (Rayleigh, 1915):
I have often been impressed by the scanty attention paid even by original workers in physics to the great principle of similitude. It happens not infrequently that results in the form of laws are put forward as novelties on the basis of elaborate experiments, which might have been predicted a priori after a few minutes consideration.
Rayleigh stopped short of teaching a formalized approach to dimensional analysis, but the French mathematician Aim Vaschy in 1892, and independently the Russian mathematician Dimitri Riabouchinsky in 1911, each formulated and then reported methods that we now call the Pi theorem (named after and introduced to most of the community by Edgar Buckingham, 1914). Since this time, dozens of texts and papers on similarity and similitude have been written. Modern similitude has been strongly leveraged by researchers in and for the field of fluid mechanics, but it has been applied successfully in many other fields. White (2016) provides references to examples in biomechanics, astrophysics, economics, chemistry, clinical medicine, social sciences, and plant genetics, among others.
We shall not attempt to review here all of the history of similarity methods or applications, but instead offer a few select milestone contributions to dimensional analysis as summarized in . Note the years listed in the table are approximate dates of contribution as suggested by Rott (1992), Gibbings (2011), and White (2016).
Brief review of important contributions to dimensional analysis |
Individual and approximate date of contribution | Summary of Contribution |
Newton, 1687 | Defines basic relationships between mass, momentum, and force. Discusses similarity in motion trajectories in the Principia. |
Euler, 1765 | Extensive discussions of physical units, unit conversion factors; in, e.g., Theoria motus corporum solidorum seu rigidorum. |
Fourier, 1822 | Dimensional homogeneity, physical quantities vs. units, similarity in heat flow in his Analytical Theory of Heat. |
Stokes, 1856 | Identifies Reynolds number as key parameter in drag effects on a pendulum. |
Maxwell, 1871 | Applies dimensional analysis to electromagnetic theory and theory of gases, introduces M, L, and T nomenclature. |
Rayleigh, 1877 | Application of similitude to wide range of problems, demonstrating wide generality of approach. Extensive applications to light scatter and fluid mechanics, and to correlating measurements of drag. |
Reynolds, 1883 | Turbulent transition experiments identify Reynolds number (named/highlighted by Sommerfeld, 1908 and later reemphasized and taught by Prandtl, 1910). |
Vaschy, 1892, and Riabouchinsky, 1911 | Independently develop formalized methods now known as Buckingham Pi theorem. |
Buckingham, 1914 | Publishes and teaches the Pi theorem. |
Bridgman, 1922 | Publishes classic book on dimensional analysis theory. |
Ipsen, 1960 | Ipsens method, publishes a book describing a step-by-step approach to dimensional analysis. |
The last entry of is a method for performing dimensional analysis due to David Carl Ipsen (1960), a professor at the University of California at Berkeley. Ipsens method is a formal, step-by-step approach that we will primarily adopt in this book for the process of dimensional analysis.
In addition to Ipsens method, we will discuss a set of rules of thumb that in this text I propose as a guide to the integration of physical intuition and experimental observations with dimensional analysis. Enabling the combination of dimensional analysis and physical intuition is a major goal of this book.
Small Changes in Geometry Can Have Significant Effects: The Effect of Roughness on Drag
Eiffel found that the resistance to a sphere moving through air changes its character somewhat suddenly at a certain velocity. The consideration of viscosity shows that the critical velocity is inversely proportional to the diameter of the sphere.
Lord Rayleigh (1915)
There are experimental data available for many types of physical phenomena. However, the problem of interest to you may not have been studied exactly. It may be very tempting to roughly approximate predictions for one geometry given data for a similar geometry (indeed we saw in chapter 7 a scaling that approximately equated the shape of a mosquito and airliner). However, it is not always the case that the behavior of one geometry can be used (i.e., stretched) to predict behavior of another. In nonlinear phenomena, a tiny change can have a big effect. Seemingly small differences in geometry can at times have significant effects. We have already seen one example of this in chapter 8, where we discussed the significant importance of pipe inner surface roughness on pressure drop. In this chapter, we discuss in some detail a similar problem: the significant effect that relatively small roughness elements can have on the drag of immersed bodies. We also here discuss how drag scaling laws obtained for one shape might be used to roughly predict the drag experienced by different shapes.