John Weiss - The Calculus Direct: An Intuitively Obvious Approach to a Basic Knowledge of the Calculus for the Casual Observer

The Calculus Direct

The Calculus Direct

An Intuitively Obvious Approach to a Basic Knowledge of the Calculus for the Casual Observer

By John Weiss

2009

Copyright John Weiss

San Antonio, Texas

2009

All pages herein may be reproduced by educators for their pupils without worry of copyright infringement

All pages herein may be reproduced by the curious for conversation with other curious people without worry of copyright infringement

Copyright laws still stand for those who wish to reproduce for monetary gain without express permission by the author John Weiss

Preface:

T he idea for this book w as formulated from my experience as an adjunct mathematics instructor at Northwest Vista comm unity college. I became involved with the developmental mathema tics in 2006 and was appalled to learn that a student without strong mathematical skills who hoped to attain a technological degree had to complete 7 semesters of mathematics to advance through calculus. This is worrisome because t he Calculus is the most important subject in modern education. Physics, engineering, biology, rocket science, and the electrical and computer systems that govern our lives derive all of their abilities from the calculus and not the algebras. With mastery of the calculus entire subjects are laid bare for the student to easily grasp. Because of this, d eveloping a competent student in this area is critical in our modern world . Unfortunately the calculus is p laced at the top of a mountain of mediocre material that systematically bores and frustrates the average prospective student to the point of hating mathematics. Although this mediocre material is useful in some respects it is not critical to the modern mind. Just as Latin has fallen from a required part of education in our modern times so must most of the curious but altogether useless algebraic puzzles that we constantly harass the modern student with. I make the statement that if we are ever to raise the average student to an appreciable technical understanding we must shun our antiquated embrace of menial algebraic puzzles and instead open the doors to modern mathematical ponderings. Although a thorough understanding of algebra is required, one does not need to master algebra before calculus can be studied. It is on this principal that I write this book. My goal is to take a willing student who is at the lowest level of mathematics and achieve a workable understanding of the calculus. In essence we will start with addition, and taking no previous mathematical knowledge for granted, follow the most efficient path to integration . If I can show, as I believe I have , that a basic understanding of Calculus can be found through one concise book then my point will be proven.

This book is not the end all of mathematical education, it is a starting point. I have left certain recommendations open and in fact explicitly state topics for further reading. I hope the student finds this book illuminating and proceeds to further exploration of the natural world and the seemingly magical connection with mathematics. It fills me with wonder that m ere thought (mathematics) could actually mirror and even predict this ether surrounding us that we refer to as reality. Maybe, hopefully, this will pique your interest as well.

The intended audience for this book is the non-traditional student who is entering college without a firm understanding in mathematics and wishes for supplemental material. It is also intended for the uninformed yet curious souls tinkering in their garage and trying to make sense of a world that has left them behind technically. It is my sincerest belief that these types of people have been and always will be the backbone of American technological innovations

As an aside for those wayward mathematical purists, this is not a completely rigorous and solid approach. There may be sections that you disagree with and dislike as far as the mathematical approach is concerned, but keep in mind that the purpose is to open doors for nontraditional as well as weak mathematical readers. Some leniency in rigor is required for these type of students.

As an aside to those readers who hope this to be a magical book unfortunately you still need to put in the effort to understand the calculus. I have written what I believe to be the route with the least amount of work, but I do no t know of a magic bullet that automatically produces understanding by merely holding the book and eating cake. Some work on the readers part is expected, even demanded, for the reader to understand everything. This does not mean it is hard or discouraging, but some effort is needed.

C h apter 1.1 Addition

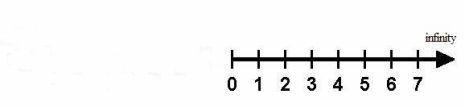
The numberline is the basis of all mathematics and we will begin our journey here. Our first lesson is how to create the numberline. We do this by starting with a line and then choosing a position on the line as a datum point. A datum point is an origin on which all other values are based upon. At this home point we list our home number - zero. Now, choose some arbitrary measurement and call this a unit measurement. We then measure one of these unit measurements to the right of zero (we chose the right of zero because we read from left to right) and we find a position which we naturally label 1 to signify one unit from zero. Measure another unit, this time starting at our labeled point 1, and we find point 2 signifying (no prizes) two units away from zero. Repeat this process repeatedly until we reach infinity. Infinity is a number that is larger than any number you can think of. We like to say that it is somewhere in the distance far - far to the right of zero and leave it at that because people begin to go crazy every so often when they study infinity too much. Im not joking, institutionalized. As such we will just define infinity as the furthest point to the right and continue to more pressing matters. Infinity, in our case, looks to be larger than seven and this should feel correct.

Lets take that same unit measurement we used to create the right side of the numberline and measure that same distance to the left of zero. But what will we label this point on the numberline? Since our values continue to become smaller as we move from right to left we will need a number that continues this pattern. But what number is less than zero?

In tangible life we cannot have anything less than zero. However, and this is a very important mathematical lesson, we are interested in the idea and not the reality. It doesnt matter to us that we cant hold a number less than zero in our hand, only that we can describe it, and we describe it as -1 (pronounced negative one). Measuring another unit value from -1 and traveling further to the left we find -2, repeating we get -3, -4, and so on until we find negative infinity. Negative infinity is considered the number furthest left of every other number and the least number if that makes sense. But we wont study infinity until youre older.

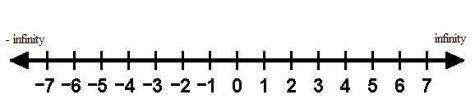
This is known as the integer numberline. An integer means any whole number positive or negative including zero. Numbers, as we have seen, are a symbolic form for writing value. Each corresponds to a particular group of units. However, to truly appreciate math, we need to create a new understanding of numbers and the numberline allows us to define a way of visualizing this. For instance, when we say the number 5, the number of fingers on a hand, we are indicating a length of five units measured to the right of zero. When we say -10 we indicate a group of ten units to the left of zero. To further examine this new way of thinking the student will be introduced to a vector.