Table of Contents
For Elvira
Preface
How can it be that mathematics, a product of human thought independent of experience, is so admirably adapted to the objects of reality?
Albert Einstein
Mathematical ideas spring from the human imagination. It is hard to conceive of a world without systems of numerationa world without numbers. Mathematics is a wealth of ideas which can be discovered, understood and enjoyed by anyone. Over the years mathematics has had some bad PR. Many believe that mathematical ideas are truly enjoyed by only a small elite group. But this mystique has no foundation. You dont have to be a mathematician or an enthusiast to be intrigued or amused by mathematical concepts.
Fractals, Googols, and other Mathematical Tales explores a variety of mathematical ideas, but does so from a lighter side. I hope you will empathize with Decimal Point, watch out for the googols, help a fractal crash the shapes party, guess what happens to Penrose the cat in Pancake World, and thereby help these and the other mathematical ideas come alive. These stories are written for those who love mathematics, for those who hate it, for those who dread and fear it, and for those who are indifferent to it. I hope that Fractals, Googols and other Mathematical Tales will amuse, entertain and inform regardless of age or background.
Penrose discovers pancake world
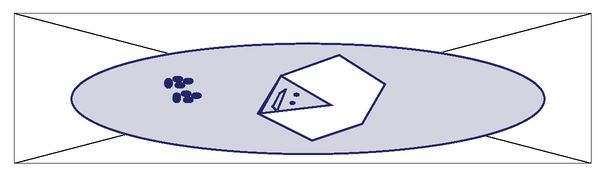
Penrose always felt especially curious when the moon was full. Tonight was one of those nights. Jumping up on the back fence, he peered down and noticed a hole in the ground he had never seen before. It was a large deep hole with no end in sight. A perfect adventure for a full moon night, Penrose thought as he slowly entered the hole. After his tail had cleared the opening, he rubbed his paws over his eyes. He was startled by the brightness of the new world he had entered. My goodness, he thought what have I stumbled into? There were creatures whose shapes he had not known existed. Everything was so very flat. He saw something that was shaped like a large hexagon, and picked it up. He suddenly heard something, shaped like a , screaming, My house! My house! Whats happened to my house? Penrose immediately put it back. And the calmed down. Whats the matter?
Penrose asked the . The addressed Penroses paws, saying My house disappeared for a moment.
Thats ridiculous. Didnt you see me pick it up? Penrose replied. Up? said the , What is up? Up is up here, said Penrose. But the , still looking at Penroses paws, had a confused expression and responded, There is no such thing or word in the world as up. What about the word down? said Penrose. Down? said the , with a blank look about it.
Penroses tail was pointing straight up as his curiosity increased. He asked the , Where am I? The replied, You are in Pancake World. Pancake World? said Penrose.
Yes, repeated the , Pancake World.
Then Penrose asked, When you speak to me, why do you talk to my paws?
Where should I address you? asked the .
Up here, replied Penrose. Up? said the .
Do you know the meaning of right and left ? asked Penrose. Yes, of course, replied the . What about the words forward , backward, and sideways? Penrose asked. Again the replied, Yes. Penrose thought a moment, and then asked, What about the words in and out ? What do the words in or out mean? asked the .
Now Penrose realized where he was and why the only talked to his paws. He understood how he had made the hexagon house disappear and reappear to the .
How Penrose appeared to in Pancake world.
Suddenly Penrose felt himself being lifted up. He opened his eyes and realized his mistress was lifting him from her geometry book on which he had fallen asleep while reading the pages on 2-dimensional objects.
The solutions and answers section at the end of the book talks about what Penrose discovered.
The drawings of cave artists, Byzantine artists rendering of two-dimensional icons, Renaissance artists capturing depth of field on their canvases, todays artists seeking to portray 4th dimensional objects all explore and use the fascinating realms of dimensions. It is possible for humans to easily visualize the first three dimensions. Although dimensions exist mathematically beyond the third, it is sometimes difficult to accept something we cannot see or imagine. Computers are used today to aid with visualization of the 4th dimension. For example, Thomas Bancroft (a mathematician) and Charles Strauss (a computer scientist) at Brown University have generated, via the computer, motion pictures of a hypercube moving in and out of 3-D space. The various images, captured at different angles of a hypercube in a 3-D world, are somewhat analogous to how Penroses paw prints were one facet of his essence revealed to the 2-D triangle.
There are various applications of multiple dimensions in the scientific world. Interest in the fourth dimension surfaced in the 19th century. For example, in 1827 Augustus Mbius, mathematician (for whom the Mbius strip is named), observed that a left hands silhouette (a 2-dimensional object) can be turned into a right hands silhouette by passing it through the third dimension. More recently, proponents of the superstring theory described the universe as 10-dimensional (9 spatial dimensions and 1 dimension of time) with its building blocks of matter and energy being infinitesimal strings. The theory speculates that at the moment of the Big Bang the 9-dimensions were equal. Then, as the universe expanded, only 3 of the spatial dimensions expanded with it. The other 6 dimensions remained entwined and encased in compact geometries that measure only 10-33 centimeters. Scientists are using 6-dimensional topological models to describe these strings.
The story of the decimal point
For many years a point named decimal lay hidden among numbers. While other points were beginning to make their dots, no one had yet found decimal. The point dotting the is was quite a braggart, always boasting it was on top of things. The point named period felt so smug because it always ended a complete thought or sentence. The