GENERAL CONCLUSIONS .
WHAT I have attempted to explain in the foregoing pages is how the scientist is to set about making a selection of the innumerable facts that are offered to his curiosity, since he is compelled to make a selection, if only by the natural infirmity of his mind, though a selection is always a sacrifice. To begin with, I explained it by general considerations, recalling, on the one hand, the nature of the problem to be solved, and on the other, seeking a better understanding of the nature of the human mind, the principal instrument in the solution. Then I explained it by examples, but not an infinity of examples, for I too had to make a selection, and I naturally selected the questions I had studied most carefully. Others would no doubt have made a different selection, but this matters little, for I think they would have reached the same conclusions.
There is a hierarchy of facts. Some are without any positive bearing, and teach us nothing but themselves. The scientist who ascertains them learns nothing but facts, and becomes no better able to foresee new facts. Such facts, it seems, occur but once, and are not destined to be repeated.
There are, on the other hand, facts that give a large return, each of which teaches us a new law. And since he is obliged to make a selection, it is to these latter facts that the scientist must devote himself.
No doubt this classification is relative, and arises from the frailty of our mind. The facts that give but a small return are the complex facts, upon which a multiplicity of circumstances exercise an appreciable influencecircumstances so numerous and so diverse that we cannot distinguish them all. But I should say, rather, that they are the facts that we consider complex, because the entanglement of these circumstances exceeds the compass of our mind. No doubt a vaster and a keener mind than ours would judge otherwise. But that matters little; it is not this superior mind that we have to use, but our own.
The facts that give a large return are those that we consider simple, whether they are so in reality, because they are only influenced by a small number of well-defined circumstances, or whether they take on an appearance of simplicity, because the multiplicity of circumstances upon which they depend obey the laws of chance, and so arrive at a mutual compensation. This is most frequently the case, and is what compelled us to enquire somewhat closely into the nature of chance. The facts to which the laws of chance apply become accessible to the scientist, who would lose heart in face of the extraordinary complication of the problems to which these laws are not applicable.
We have seen how these considerations apply not only to the physical but also to the mathematical sciences. The method of demonstration is not the same for the physicist as for the mathematician. But their methods of discovery are very similar. In the case of both they consist in rising from the fact to the law, and in seeking the facts that are capable of leading up to a law.
In order to elucidate this point, I have exhibited the mathematicians mind at work, and that under three forms: the mind of the inventive and creative mathematician; the mind of the unconscious geometrician who, in the days of our far-off ancestors or in the hazy years of our infancy, constructed for us our instinctive notion of space; and the mind of the youth in a secondary school for whom the master unfolds the first principles of the science, and seeks to make him understand its fundamental definitions. Throughout we have seen the part played by intuition and the spirit of generalization, without which these three grades of mathematicians, if I may venture so to express myself, would be reduced to equal impotence.
And in demonstration itself logic is not all. The true mathematical reasoning is a real induction, differing in many respects from physical induction, but, like it, proceeding from the particular to the universal. All the efforts that have been made to upset this order, and to reduce mathematical induction to the rules of logic, have ended in failure, but poorly disguised by the use of a language inaccessible to the uninitiated.
The examples I have drawn from the physical sciences have shown us a good variety of instances of facts that give a large return. A single experiment of Kaufmanns upon radium rays revolutionizes at once Mechanics, Optics, and Astronomy. Why is this? It is because, as these sciences developed, we have recognized more clearly the links which unite them, and at last we have perceived a kind of general design of the map of universal science. There are facts common to several sciences, like the common fountain head of streams diverging in all directions, which may be compared to that nodal point of the St. Gothard from which there flow waters that feed four different basins.
Then we can make our selection of facts with more discernment than our predecessors, who regarded these basins as distinct and separated by impassable barriers.
It is always simple facts that we must select, but among these simple facts we should prefer those that are situated in these kinds of nodal points of which I have just spoken.
And when sciences have no direct link, they can still be elucidated mutually by analogy. When the laws that regulate gases were being studied, it was realized that the fact in hand was one that would give a great return, and yet this return was still estimated below its true value, since gases are, from a certain point of view, the image of the Milky Way; and these facts, which seemed to be of interest only to the physicist, will soon open up new horizons to the astronomer, who little expected it.
Lastly, when the geodesist finds that he has to turn his glass a few seconds of arc in order to point it upon a signal that he has erected with much difficulty, it is a very small fact, but it is a fact giving a great return, not only because it reveals the existence of a little hump upon the terrestrial geoid, for the little hump would of itself be of small interest, but because this hump gives him indications as to the distribution of matter in the interior of the globe, and, through that, as to the past of our planet, its future, and the laws of its development.
THE END .
A CATALOG OF SELECTED DOVER BOOKS IN ALL FIELDS OF INTEREST
100 BEST-LOVED POEMS, Edited by Philip Smith. The Passionate Shepherd to His Love, Shall I compare thee to a summers day? Death, be not proud, The Raven, The Road Not Taken, plus works by Blake, Wordsworth, Byron, Shelley, Keats, many others. 96pp. 5 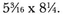 .
.
0-486-28553-7
100 SMALL HOUSES OF THE THIRTIES, Brown-Blodgett Company. Exterior photographs and floor plans for 100 charming structures. Illustrations of models accompanied by descriptions of interiors, color schemes, closet space, and other amenities. 200 illustrations. 112pp. 8 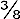 x 11.
x 11.
0-486-44131-8
1000 TURN-OF-THE-CENTURY HOUSES: With Illustrations and Floor Plans, Herbert C. Chivers. Reproduced from a rare edition, this showcase of homes ranges from cottages and bungalows to sprawling mansions. Each house is meticulously illustrated and accompanied by complete floor plans. 256pp. 9 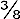













 .
. x 11.
x 11.