Herzberg - Stochastic Calculus with Infinitesimals

Stochastic Calculus with Infinitesimals — read online for free the complete book (whole text) full work

Below is the text of the book, divided by pages. System saving the place of the last page read, allows you to conveniently read the book "Stochastic Calculus with Infinitesimals" online for free, without having to search again every time where you left off. Put a bookmark, and you can go to the page where you finished reading at any time.

Light

Font size:

↓

↑

Georgia Tahoma Arial Verdana

Reset

Interval:

↓

↑

Bookmark:

Make

1234567...51

Frederik Herzberg Lecture Notes in Mathematics Stochastic Calculus with Infinitesimals 2013 10.1007/978-3-642-33149-7_1 Springer-Verlag Berlin Heidelberg 2013

1. Infinitesimal Calculus, Consistently and Accessibly

Frederik S. Herzberg 1, 2

(1)

Institute of Mathematical Economics, Bielefeld University, Bielefeld, Germany

(2)

Munich Center for Mathematical Philosophy, Ludwig Maximilian University of Munich, Munich, Germany

Abstract

The most important feature of Nelsons [60] radically elementary analysis is the discretization of the continuum. The crucial step herein is the consistent use of infinitely large (nonstandard) numbers and infinitesimals, in a manner which was first proposed by Nelson through the axiom system of Internal Set Theory [59], motivated by the groundbreaking work of Abraham Robinson [66, 67]. One decade on, Nelson [60] introduced an even more elementary, yet still very powerful, formal system, which we shall review presently.

The most important feature of Nelsons [] introduced an even more elementary, yet still very powerful, formal system, which we shall review presently.

1.1 An Accessible Axiom System for Infinitesimal Calculus: Minimal Internal Set Theory

Mathematical analysis broadly conceived (including probability theory) can be made much more intuitive if one allows for the use of infinitesimals as engineers, and partially also applied mathematicians, have done for centuries. A positive infinitesimal is a number which is greater than zero, yet in some sense arbitrarily smallviz. less than 12, less than 13, less than 14, less than 15 etc. In other words, it is a number which is positive, yet smaller than the reciprocal of any standard natural numberwherein, of course, the term standard still is in need of being defined.

So, on the one hand, the mathematical community has known infinitesimals since at least the days of Leibniz, and practitioners successfully use them every day. On the other hand, it is not immediately obvious how to give a rigorous definition of the predicate standard or equivalently of the notion of an infinitesimal.

While there are several approaches to accomplish this, the first modern rigorous attempt to define infinitesimalswhich will serve as our first motivationis due to Robinson []. Robinson extended the real line with a huge number of additional elements so that it became a real-ordered field which also contained infinitesimals and infinitely large numbers.

The technique that Robinson employed has some similarity to the construction of the reals out of Cauchy sequences of rational numbers: (a) The new numbers that he constructed are equivalence classes of real numbers (where the equivalence relation is such that two sequences are equivalent if and only if they agree on a set to which a given non-trivial {0,1}-valued finitely-additive measure on the set of natural numbers assigns mass 1). (c) The original real numbers are embedded into the new number system as equivalence classes of constant sequences.

On this Robinsonian account, the standard natural numbers, are images of ordinary natural numbers under the canonical embedding. For an example of an infinitesimal, just consider the -equivalence class of any null sequence of real numbers. If one considers -equivalence classes of strictly increasing sequences of natural numbers, one obtains infinitely large numbers, which nevertheless have some relation to natural numbers and will therefore be called nonstandard natural numbers .

We will not go further into the details of Robinsons delicate construction of which we only sketched the very basic steps. Readers who are interested in learning more about Robinsons nonstandard analysis and its exciting applications are encouraged to have a look at Appendix B and the references therein. Instead we will present a simple axiom system which captures a minimal fragment of nonstandard analysis, but is just powerful enough for our purposes of developing a stochastic calculus with infinitesimals.

In order to simplify both the presentation of the axiom system and the later material, we take an important, at first sight radical step: Henceforth, when we refer to real numbers or to the setR , we mean (elements of) the extended number system which, of course, not only contains ordinary real numbers, but also other, nonstandard real numbers such as infinitesimals and infinitely large numbers. If we want to refer to the ordinary natural numbers, we will refer to them as standard natural numbers. Otherwise, the term natural number can refer to a standard or nonstandard natural number, andNwill be used to denote the set of all (standard and nonstandard) natural numbers in this sense.

With these conventions, we now introduce the following collection of axioms and axiom schemes, which we shall henceforth refer tofor historical reasons (see Sect.A.1 of Appendix A)as Minimal Internal Set Theory , abbreviated minIST :

• All theorems
• 0 is standard.
• For every , if n is standard, then n +1 is standard, too.
• There exists a nonstandard natural numbern , i.e. some which is not standard.
• ( External Induction ) If A ( v ) is any formula of the new, extended language such that A (0) holds and such that A ( n ) entails 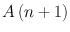for all standard n , then A ( n ) readily holds for all standard n .

Unless explicitly stated otherwise, we will in this book always assume the axioms of Minimal Internal Set Theory (minIST).

Formulae which do not involve the predicate standard will be called internal , because they can already be expressed in conventional mathematics. All other formulae (i.e. precisely those that involve the predicate standard) are called external .

Note that we have not allowed that external formulae may be used to define new sets; violation of this rule is called illegal set formation . (Robinsonian nonstandard analysis has methods to treat external sets, too; in that framework, these sets are no longer illegal.) For example, the usual principle of mathematical induction only pertains to internal formulae; if one wishes to prove an external formula by means of induction, one has to apply the above axiom scheme of External Induction.

1.2 Finer Classification of the Reals: Finite vs. Limited

By the first axiom of minIST , we just inherit all results and concepts from conventional mathematics. For instance, a set v is finite if and only if v is bijective to {0,, n 1} for some n N , which is then called the cardinality of v .

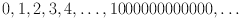Now the number of elements of a finite set may be nonstandard. But any nonstandard natural number is greater than every standard natural number, whichcombined with the fact that are all standardshows that nonstandard natural numbers are very large indeed, yea, in some sense unlimited . In particular, finite probability spaces can have an unlimited number of elements and thus be very rich.

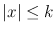Any real number x which satisfies for some standard k is called limited (denoted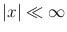

Next page

1234567...51

Light

Font size:

↓

↑

Georgia Tahoma Arial Verdana

Reset

Interval:

↓

↑

Bookmark:

Make

Similar books «Stochastic Calculus with Infinitesimals»

Look at similar books to Stochastic Calculus with Infinitesimals. We have selected literature similar in name and meaning in the hope of providing readers with more options to find new, interesting, not yet read works.

Colin Conrad Adams

How to Ace the Rest of Calculus: The Streetwise Guide, Including MultiVariable Calculus

Tânia Tomé

Stochastic Dynamics and Irreversibility

Gushchin

Stochastic Calculus for Quantitative Finance

Ju

Stochastic Dynamics of Power Systems

Larry Herzberg

Speak and Read Chinese: Fun Mnemonic Devices for Remembering Chinese Words and Their Tones

Jean-François Le Gall

Brownian Motion, Martingales, and Stochastic Calculus

Uwe Hassler

Stochastic Processes and Calculus

CK-12 Foundation [Foundation

CK-12 Calculus

Chris Monahan [Chris Monahan]

Calculus II

Herzberg Larry

Chinese proverbs and popular sayings : with observations on culture and language

Venkatarama Krishnan

Nonlinear Filtering and Smoothing: An Introduction to Martingales, Stochastic Integrals and Estimation

H. Jerome Keisler

Elementary Calculus: An Infinitesimal Approach

Discussion, reviews of the book Stochastic Calculus with Infinitesimals and just readers' own opinions. Leave your comments, write what you think about the work, its meaning or the main characters. Specify what exactly you liked and what you didn't like, and why you think so.