Research & Education Association 61 Ethel Road West
Piscataway, New Jersey 08854
E-mail: ALGEBRA & TRIGONOMETRY
SUPER REVIEW Copyright 2013 by Research & Education Association, Inc. Prior edition copyright 2000 by Research & Education Association, Inc. All rights reserved. No part of this book may be reproduced in any form without permission of the publisher. Printed in the United States of America Library of Congress Control Number 2012949163 ISBN-13: 978-0-7386-1118-1
ISBN-10: 0-7386-1118-2 LIMIT OF LIABILITY/DISCLAIMER OF WARRANTY: Publication of this work is for the purpose of test preparation and related use and subjects as set forth herein. While every effort has been made to achieve a work of high quality, neither Research & Education Association, Inc., nor the authors and other contributors of this work guarantee the accuracy or completeness of or assume any liability in connection with the information and opinions contained herein and in REAs software and/or online materials.
REA and the authors and other contributors shall in no event be liable for any personal injury, property or other damages of any nature whatsoever, whether special, indirect, consequential or compensatory, directly or indirectly resulting from the publication, use or reliance upon this work. All trademarks cited in this publication are the property of their respective owners. Cover image: iStockphoto.com/CTRd
 | SUPER REVIEW and REA are registered trademarks
of Research & Education Association, Inc. |
I-12
REAs
Algebra &
Trigonometry
Super Review
Need help with algebra and trigonometry? Want a quick review or refresher for class? This is the book for you! REAs Algebra & Trigonometry Super Review gives you everything you need to know! This Super Review can be used as a supplement to your high school or college textbook, or as a handy guide for anyone who needs a fast review of the subject. Comprehensive, yet concise coverage review covers the material that is typically taught in a beginning-level algebra and trigonometry course. Each topic is presented in a clear and easy-to-understand format that makes learning easier.
Questions and answers for each topic let you practice what youve learned and build your algebra and trigonometry skills. End-of-chapter quizzes gauge your understanding of the important information you need to know, so youll be ready for any algebra and trigonometry problem you encounter on your next quiz or test. Whether you need a quick refresher on the subject, or are prepping for your next test, we think youll agree that REAs Super Review provides all you need to know!
Available Super Review Titles
ARTS/HUMANITIES
Basic Music Classical Mythology History of Architecture History of Greek Art
BUSINESS
Accounting Macroeconomics Microeconomics
COMPUTER SCIENCE
C++ Java
HISTORY
Canadian History European History United States History
LANGUAGES
English French French Verbs Italian Japanese for Beginners Japanese Verbs Latin Spanish
MATHEMATICS
Algebra & Trigonometry Basic Math & Pre-Algebra Calculus Geometry Linear Algebra Pre-Calculus Statistics
SCIENCES
Anatomy & Physiology Biology Chemistry Entomology Geology Microbiology Organic Chemistry I & II Physics
SOCIAL SCIENCES
Psychology I & II Sociology
WRITING
College & University Writing
About Research & Education Association
Founded in 1959, Research & Education Association is dedicated to publishing the finest and most effective educational materialsincluding study guides and test prepsfor students in middle school, high school, college, graduate school, and beyond. Today, REAs wide-ranging catalog is a leading resource for teachers, students, and professionals. Visit www.rea.com to see a complete listing of all our titles.
Acknowledgments
We would like to thank Pam Weston, Publisher, for setting the quality standards for production integrity and managing the publication to completion; Larry B.
Kling, Vice President, Editorial, for his supervision of revisions and overall direction; Kelli Wilkins, Copywriter, for coordinating development of this edition; Mel Friedman, REAs Lead Mathematics Editor, for his editorial review and revisions; Christine Saul, Senior Graphic Designer, for typesetting this edition and designing our cover.
Contents
A set is defined as a collection of items. Each individual item belonging to a set is called an element or member of that set. Sets are usually represented by capital letters, elements by lowercase letters. If an item k belongs to a set A , we write k A ( k is an element of A ). If k is not in A , we write k A ( k is not an element of A ).
The order of the elements in a set does not matter: 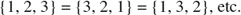 A set can be described in two ways: 1) it can be listed element by element, or 2) a rule characterizing the elements in a set can be formulated. For example, given the set A of the whole numbers starting with 1 and ending with 9, we can describe it either as A = {1, 2, 3, 4, 5, 6, 7, 8, 9} or as {the set of whole numbers greater than 0 and less than 10}. In both methods, the description is enclosed in brackets. A kind of shorthand is often used for the second method of set description; instead of writing out a complete sentence in between the brackets, we write instead
A set can be described in two ways: 1) it can be listed element by element, or 2) a rule characterizing the elements in a set can be formulated. For example, given the set A of the whole numbers starting with 1 and ending with 9, we can describe it either as A = {1, 2, 3, 4, 5, 6, 7, 8, 9} or as {the set of whole numbers greater than 0 and less than 10}. In both methods, the description is enclosed in brackets. A kind of shorthand is often used for the second method of set description; instead of writing out a complete sentence in between the brackets, we write instead 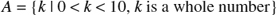 This is read as the set of all elements k such that k is greater than 0 and less than 10, where k is a whole number. A set not containing any members is called the empty or null set. It is written either as or { }.
This is read as the set of all elements k such that k is greater than 0 and less than 10, where k is a whole number. A set not containing any members is called the empty or null set. It is written either as or { }.
Problem Solving Examples:  List the elements of the set:
List the elements of the set: 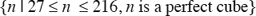
 This expression may be read as: the set of all elements (numbers) n , such that n is a perfect cube between 27 and 216 inclusive. One way to list the elements is:
This expression may be read as: the set of all elements (numbers) n , such that n is a perfect cube between 27 and 216 inclusive. One way to list the elements is: 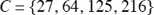 Note that we could have chosen any other letter for the set, but C suggests the word cube.
Note that we could have chosen any other letter for the set, but C suggests the word cube.  Given that N = {9, 15, 21, , 99}, describe N in words.
Given that N = {9, 15, 21, , 99}, describe N in words.  First, we observe that 9, 15, 21, and 99 are all odd numbers. However, two odd numbers are missing between 9 and 15 and also between 15 and 21. If we continue skipping two odd numbers, we should finally arrive at 99.
First, we observe that 9, 15, 21, and 99 are all odd numbers. However, two odd numbers are missing between 9 and 15 and also between 15 and 21. If we continue skipping two odd numbers, we should finally arrive at 99.
So we can describe the set as: all third odd numbers between 9 and 99, inclusive. (Though technically correct, this is an awkward construction.) We notice also that 9, 15, 21, and 99 are all odd multiples of 3. So an alternative description would be: the set of odd multiples of 3 between 9 and 99 inclusive. (This is a more elegant description.) 


















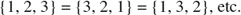 A set can be described in two ways: 1) it can be listed element by element, or 2) a rule characterizing the elements in a set can be formulated. For example, given the set A of the whole numbers starting with 1 and ending with 9, we can describe it either as A = {1, 2, 3, 4, 5, 6, 7, 8, 9} or as {the set of whole numbers greater than 0 and less than 10}. In both methods, the description is enclosed in brackets. A kind of shorthand is often used for the second method of set description; instead of writing out a complete sentence in between the brackets, we write instead
A set can be described in two ways: 1) it can be listed element by element, or 2) a rule characterizing the elements in a set can be formulated. For example, given the set A of the whole numbers starting with 1 and ending with 9, we can describe it either as A = {1, 2, 3, 4, 5, 6, 7, 8, 9} or as {the set of whole numbers greater than 0 and less than 10}. In both methods, the description is enclosed in brackets. A kind of shorthand is often used for the second method of set description; instead of writing out a complete sentence in between the brackets, we write instead 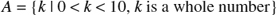 This is read as the set of all elements k such that k is greater than 0 and less than 10, where k is a whole number. A set not containing any members is called the empty or null set. It is written either as or { }.
This is read as the set of all elements k such that k is greater than 0 and less than 10, where k is a whole number. A set not containing any members is called the empty or null set. It is written either as or { }. List the elements of the set:
List the elements of the set: 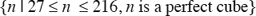
 This expression may be read as: the set of all elements (numbers) n , such that n is a perfect cube between 27 and 216 inclusive. One way to list the elements is:
This expression may be read as: the set of all elements (numbers) n , such that n is a perfect cube between 27 and 216 inclusive. One way to list the elements is: 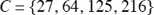 Note that we could have chosen any other letter for the set, but C suggests the word cube.
Note that we could have chosen any other letter for the set, but C suggests the word cube.