ESSENTIAL MODERN PHYSICS Study Guide Workbook (with Full Solutions) Chris McMullen, Ph.D.  Essential Modern Physics Study Guide Workbook Chris McMullen, Ph.D. monkeyphysicsblog.wordpress.com improveyourmathfluency.com chrismcmullen.com Copyright 2019 Chris McMullen, Ph.D. All rights reserved.
Essential Modern Physics Study Guide Workbook Chris McMullen, Ph.D. monkeyphysicsblog.wordpress.com improveyourmathfluency.com chrismcmullen.com Copyright 2019 Chris McMullen, Ph.D. All rights reserved.  Zishka Publishing ISBN: 978-1-941691-28-1 Textbooks > Science > Physics Study Guides > Workbooks> Science CONTENTS
Zishka Publishing ISBN: 978-1-941691-28-1 Textbooks > Science > Physics Study Guides > Workbooks> Science CONTENTS
INTRODUCTION
The goal of this study guide workbook is to provide practiceand help carrying out essential problem-solving strategies that are standard inmodern physics. The aim here is not to overwhelm the student with comprehensivecoverage of every type of problem, but to focus on the main strategies andtechniques with which most physics students struggle.
This workbook is not intended to serve as a substitute forlectures or for a textbook, but is rather intended to serve as a valuablesupplement. Each chapter includes a concise review of the essentialinformation, a handy outline of the problem-solving strategies, and exampleswhich show step-by-step how to carry out the procedure. This is not intended toteach the material, but is designed to serve as a time-saving review forstudents who have already been exposed to the material in class or in atextbook. Students who would like more examples or a more thorough introductionto the material should review their lecture notes or read their textbooks. Every problem in this study guide workbook applies the samestrategy which is solved step-by-step in at least one example within thechapter. Study the examples and then follow them closely in order to completethe exercises.
Many of the exercises are broken down into parts to help guidethe student through the exercises. Each exercise tabulates the correspondinganswers on the same page. Students can find full solutions at the end of eachchapter. The prerequisites for using this workbook includefirst-year physics (including energy, waves, and electricity and magnetism) andcalculus (including derivatives and integrals). Although Schrdingers equationin quantum mechanics is a differential equation, students do not need previous exposureto differential equations: This workbook provides a concise introduction tobasic differential equations in Chapter 12, and shows how to apply thesemethods in the remaining chapters. Special relativity the physics of therelative motion of objects where at least one object is traveling at a veryhigh speed (compared to the speed of light). Ether a hypothetical substance oncebelieved to fill space; it was believed to serve as a medium for thetransmission of light waves. Photon a single particle in a beam oflight. Interferometer a device involving theinterference of two beams of light, which was used by Michelson and Morley tomeasure the speed of light relative to the earth. Time dilation the phenomenon whereby timeappears to travel more slowly for objects moving fast (close to light speed)relative to other observers. Length contraction the phenomenon wherebyobjects moving fast (close to light speed) appear shorter relative to otherobservers. Simultaneity when two events occur at theexact same moment relative to an observer, the events are said to besimultaneous for that observer. Momentum mass times velocity. Inertia the natural tendency of any objectto maintain constant momentum. Mass a measure of inertia. Vacuum a region of space completely devoidof matter (it doesnt even contain air). Inertial reference frame a frame thattravels with constant velocity. Inertial reference frame a frame thattravels with constant velocity.
Galilean Relativity In our everyday experience with objects that travelmuch slower than the speed of light, relative velocities appear to obey the formulafor vector addition. Suppose that one observer (designated R) is at restwhile a second observer (designated M) is moving with speed v relativeto the first observer. Suppose also that each observer sets up a coordinatesystem with the x-axis along the relative velocity  If each observer measures the velocity of anobject, the x-components of the velocities that they measure (uRand uM) are related by the following vector addition formula,provided that neither observer nor the object are traveling close to lightspeed. uM = uRv This equation is actually pretty simple: Its justsubtraction. The challenge is to remember what the notation means (M stands formoving, while R stands for rest) so that you can apply the equation correctly.We will explore this equation further in the examples that follow. The Mysterious Ether Physicists once believed in a hypotheticalsubstance called the ether, which was believed to permeate all of space.At the time, all other waves besides light were known to travel in a medium.You can see ripples travel along the surface of water.
If each observer measures the velocity of anobject, the x-components of the velocities that they measure (uRand uM) are related by the following vector addition formula,provided that neither observer nor the object are traveling close to lightspeed. uM = uRv This equation is actually pretty simple: Its justsubtraction. The challenge is to remember what the notation means (M stands formoving, while R stands for rest) so that you can apply the equation correctly.We will explore this equation further in the examples that follow. The Mysterious Ether Physicists once believed in a hypotheticalsubstance called the ether, which was believed to permeate all of space.At the time, all other waves besides light were known to travel in a medium.You can see ripples travel along the surface of water.
Sound waves createalternating regions of compression (high pressure) and rarefaction (lowpressure) in a medium such as air, water, wood, or metal. Light was also knownto be a wave, yet sunlight can travel through space (a near-perfect vacuum).Since all other waves required a medium in which to propagate, the concept ofthe ether could explain the transmission of light through space. It turns out that the ether hypothesis is incorrect,as demonstrated by the Michelson-Morley experiment. Light can travel through aperfect vacuum (without an ether). The Earth, Light, and the Hypothetical Ether The result of the Michelson-Morley experimentthat theether doesnt existcame as a big shock to the physics community at thetime. To understand why, we must explore the ether as it had been believed toexist.
The ether was believed to permeate all of space. The reference frame ofthe ether was believed to serve as an absolute reference frame. That is, thespeed of light was only believed to travel c = 2.9979 108m/s in a reference frame that was at rest relative to the ether. Furthermore,it was believed that the vector addition equation of Galilean relativityapplied to objects moving any speed, including light itself. (Like the ether,this also proved to be incorrect.) The earth orbits the sun and therefore must bemoving relative to the hypothetical ether (as the direction of earths velocityis constantly changing). From the point of view of the ether, the earth ismoving relative to the ether with instantaneous speed v.
From the pointof view of earthlings, the earth seems to be stationary and we would insteadinterpret the ether to be moving with speed v. (You should haveexperience with this. If you are sitting in a bus that is moving, objectsoutside of the bus appear to be moving relative to you.) From the perspectiveof stationary earthlings, the ether is seen as an ether wind (whenyou run through air that is originally still, the air seems to pass by you likea sort of wind). Imagine that you get in a motorboat and travelalong the surface of a river. The boat would travel with a speed of 30 m/s on astill pond, but there is a river current of 10 m/s. When the boat is headeddownstream, it would be traveling 30 + 10 = 40 m/s relative to the land.
Whenthe boat is headed upstream, it would be traveling 30 10 = 20 m/s relative tothe land. When the boat is headed cross-stream, apply the Pythagorean theoremto determine that the boat travels 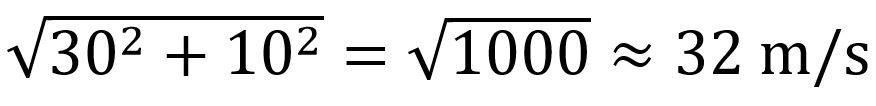









 Essential Modern Physics Study Guide Workbook Chris McMullen, Ph.D. monkeyphysicsblog.wordpress.com improveyourmathfluency.com chrismcmullen.com Copyright 2019 Chris McMullen, Ph.D. All rights reserved.
Essential Modern Physics Study Guide Workbook Chris McMullen, Ph.D. monkeyphysicsblog.wordpress.com improveyourmathfluency.com chrismcmullen.com Copyright 2019 Chris McMullen, Ph.D. All rights reserved.  Zishka Publishing ISBN: 978-1-941691-28-1 Textbooks > Science > Physics Study Guides > Workbooks> Science CONTENTS
Zishka Publishing ISBN: 978-1-941691-28-1 Textbooks > Science > Physics Study Guides > Workbooks> Science CONTENTS If each observer measures the velocity of anobject, the x-components of the velocities that they measure (uRand uM) are related by the following vector addition formula,provided that neither observer nor the object are traveling close to lightspeed. uM = uRv This equation is actually pretty simple: Its justsubtraction. The challenge is to remember what the notation means (M stands formoving, while R stands for rest) so that you can apply the equation correctly.We will explore this equation further in the examples that follow. The Mysterious Ether Physicists once believed in a hypotheticalsubstance called the ether, which was believed to permeate all of space.At the time, all other waves besides light were known to travel in a medium.You can see ripples travel along the surface of water.
If each observer measures the velocity of anobject, the x-components of the velocities that they measure (uRand uM) are related by the following vector addition formula,provided that neither observer nor the object are traveling close to lightspeed. uM = uRv This equation is actually pretty simple: Its justsubtraction. The challenge is to remember what the notation means (M stands formoving, while R stands for rest) so that you can apply the equation correctly.We will explore this equation further in the examples that follow. The Mysterious Ether Physicists once believed in a hypotheticalsubstance called the ether, which was believed to permeate all of space.At the time, all other waves besides light were known to travel in a medium.You can see ripples travel along the surface of water.