Pinaki Mondal - How Many Zeroes?: Counting Solutions of Systems of Polynomials via Toric Geometry at Infinity
Here you can read online Pinaki Mondal - How Many Zeroes?: Counting Solutions of Systems of Polynomials via Toric Geometry at Infinity full text of the book (entire story) in english for free. Download pdf and epub, get meaning, cover and reviews about this ebook. year: 2021, publisher: Springer, genre: Children. Description of the work, (preface) as well as reviews are available. Best literature library LitArk.com created for fans of good reading and offers a wide selection of genres:
Romance novel
Science fiction
Adventure
Detective
Science
History
Home and family
Prose
Art
Politics
Computer
Non-fiction
Religion
Business
Children
Humor
Choose a favorite category and find really read worthwhile books. Enjoy immersion in the world of imagination, feel the emotions of the characters or learn something new for yourself, make an fascinating discovery.
- Book:How Many Zeroes?: Counting Solutions of Systems of Polynomials via Toric Geometry at Infinity
- Author:
- Publisher:Springer
- Genre:
- Year:2021
- Rating:5 / 5
- Favourites:Add to favourites
- Your mark:
How Many Zeroes?: Counting Solutions of Systems of Polynomials via Toric Geometry at Infinity: summary, description and annotation
We offer to read an annotation, description, summary or preface (depends on what the author of the book "How Many Zeroes?: Counting Solutions of Systems of Polynomials via Toric Geometry at Infinity" wrote himself). If you haven't found the necessary information about the book — write in the comments, we will try to find it.
This graduate textbook presents an approach through toric geometry to the problem of estimating the isolated solutions (counted with appropriate multiplicity) of n polynomial equations in n variables over an algebraically closed field. The text collects and synthesizes a number of works on Bernsteins theorem of counting solutions of generic systems, ultimately presenting the theorem, commentary, and extensions in a comprehensive and coherent manner. It begins with Bernsteins original theorem expressing solutions of generic systems in terms of the mixed volume of their Newton polytopes, including complete proofs of its recent extension to affine space and some applications to open problems. The text also applies the developed techniques to derive and generalize Kushnirenkos results on Milnor numbers of hypersurface singularities, which has served as a precursor to the development of toric geometry. Ultimately, the book aims to present material in an elementary format, developing all necessary algebraic geometry to provide a truly accessible overview suitable to second-year graduate students.
Pinaki Mondal: author's other books
Who wrote How Many Zeroes?: Counting Solutions of Systems of Polynomials via Toric Geometry at Infinity? Find out the surname, the name of the author of the book and a list of all author's works by series.











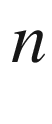 polynomial equations in
polynomial equations in 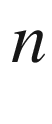 variables over an algebraically closed field
variables over an algebraically closed field  . The outcome of this approach is the number of solutions for generic systems in terms of their Newton polytopes, and an explicit characterization of what makes a system generic. The pioneering work in this field was done in the 1970s by Kushnirenko, Bernstein and Khovanskii, who completely solved the problem of counting solutions of generic systems on the torus
. The outcome of this approach is the number of solutions for generic systems in terms of their Newton polytopes, and an explicit characterization of what makes a system generic. The pioneering work in this field was done in the 1970s by Kushnirenko, Bernstein and Khovanskii, who completely solved the problem of counting solutions of generic systems on the torus 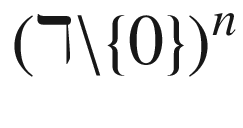 . In the context of our problem, however, the natural domain of solutions is not the torus, but the affine space
. In the context of our problem, however, the natural domain of solutions is not the torus, but the affine space  . There were a number of works on extending Bernsteins theorem to the case of affine space, and recently it has been completely resolved, the final steps having been carried out by the author.
. There were a number of works on extending Bernsteins theorem to the case of affine space, and recently it has been completely resolved, the final steps having been carried out by the author.