Jacobson - Basic Algebra II

BASIC
ALGEBRA
II

Second Edition

Nathan Jacobson

Yale University

Dover Publications, Inc.
Mineola, New York

To Mike and Polly

Copyright

Copyright 1980, 1989 by Nathan Jacobson
All rights reserved.

Bibliographical Note

This Dover edition, first published in 2009, is an unabridged republication of the 1989 second edition of the work originally published by W. H. Freeman and Company, San Francisco, in 1980.

Library of Congress Cataloging-in-Publication Data

Jacobson, Nathan, 19101999.

Basic algebra / Nathan Jacobson. Dover ed.
p. cm.

Originally published: 2nd ed. San Francisco : W.H. Freeman, 19851989.

ISBN-13: 978-0486-47189-1 (v. 1)

ISBN-10: 0-486-47189-6 (v. 1)

ISBN 13: 978-0-486-47187-7 (v. 2)

ISBN-10 0-486-47187-X (v. 2)

1. Algebra I. Title.

QA154.2.J32 2009
512.9dc22

2009006506

Manufactured in the United States by Courier Corporation
47187X01
www.doverpublications.com

Contents of Basic Algebra I

INTRODUCTION: CONCEPTS FROM SET THEORY.
THE INTEGERS

0.1 The power set of a set

0.2 The Cartesian product set. Maps

0.3 Equivalence relations. Factoring a map through an equivalence relation

0.4 The natural numbers

0.5 The number system 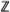of integers

0.6 Some basic arithmetic facts about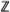

0.7 A word on cardinal numbers

1 MONOIDS AND GROUPS

1.1 Monoids of transformations and abstract monoids

1.2 Groups of transformations and abstract groups

1.3 Isomorphism. Cayleys theorem

1.4 Generalized associativity. Commutativity

1.5 Submonoids and subgroups generated by a subset. Cyclic groups

1.6 Cycle decomposition of permutations

1.7 Orbits. Cosets of a subgroup

1.8 Congruences. Quotient monoids and groups

1.9 Homomorphisms

1.10 Subgroups of a homomorphic image. Two basic isomorphism theorems

1.11 Free objects. Generators and relations

1.12 Groups acting on sets

1.13 Sylows theorems

2 RINGS

2.1 Definition and elementary properties

2.2 Types of rings

2.3 Matrix rings

2.4 Quaternions

2.5 Idealsi quotient rings

2.6 Ideals and quotient rings for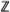

2.7 Homomorphisms of rings. Basic theorems

2.8 Anti-isomorphisms

2.9 Field of fractions of a commutative domain

2.10 Polynomial rings

2.11 Some properties of polynomial rings and applications

2.12 Polynomial functions

2.13 Symmetric polynomials

2.14 Factorial monoids and rings

2.15 Principal ideal domains and Euclidean domains

2.16 Polynomial extensions of factorial domains

2.17 Rings (rings without unit)

3 MODULES OVER A PRINCIPAL IDEAL DOMAIN

3.1 Ring of endomorphisms of an abelian group

3.2 Left and right modules

3.3 Fundamental concepts and results

3.4 Free modules and matrices

3.5 Direct sums of modules

3.6 Finitely generated modules over a p.i.d. Preliminary results

3.7 Equivalence of matrices with entries in a p.i.d.

3.8 Structure theorem for finitely generated modules over a p.i.d.

3.9 Torsion modulesi primary componentsi invariance theorem

3.10 Applications to abelian groups and to linear transformations

3.11 The ring of endomorphisms of a finitely generated module over a p.i.d.

4 GALOIS THEORY OF EQUATIONS

4.1 Preliminary resultsi some oldi some new

4.2 Construction with straight-edge and compass

4.3 Splitting field of a polynomial

4.4 Multiple roots

4.5 The Galois group. The fundamental Galois pairing

4.6 Some results on finite groups

4.7 Galois criterion for solvability by radicals

4.8 The Galois group as permutation group of the roots

4.9 The general equation of the nth degree

4.10 Equations with rational coefficients and symmetric group as Galois group

4.11 Constructible regular n-gons

4.12 Transcendence of e and . The Lindemann-Weierstrass theorem

4.13 Finite fields

4.14 Special bases for finite dimensional extension fields

4.15 Traces and norms

4.16 Mod p reduction

5 REAL POLYNOMIAL EQUATIONS AND INEQUALITIES

5.1 Ordered fields. Real closed fields

5.2 Sturms theorem

5.3 Formalized Euclidean algorithm and Sturms theorem

5.4 Elimination procedures. Resultants

5.5 Decision method for an algebraic curve

5.6 Generalized Sturms theorem. Tarskis principle

6 METRIC VECTOR SPACES AND THE CLASSICAL GROUPS

6.1 Linear functions and bilinear forms

6.2 Alternate forms

6.3 Quadratic forms and symmetric bilinear forms

6.4 Basic concepts of orthogonal geometry

6.5 Witts cancellation theorem

6.6 The theorem of Cartan-Dieudonne

6.7 Structure of the linear group GLn(F)

6.8 Structure of orthogonal groups

6.9 Symplectic geometry. The symplectic group

6.10 Orders of orthogonal and symplectic groups over a finite field

6.11 Postscript on hermitian forms and unitary geometry

7 ALGEBRAS OVER A FIELD

7.1 Definition and examples of associative algebras

7.2 Exterior algebras. Application to determinants

7.3 Regular matrix representations of associative algebras. Norms and traces

7.4 Change of base field. Transitivity of trace and norm

7.5 Non-associative algebras. Lie and Jordan algebras

7.6 Hurwitz problem. Composition algebras

7.7 Frobenius and Wedderburns theorems on associative division algebras

8 LATTICES AND BOOLEAN ALGEBRAS

8.1 Partially ordered sets and lattices

8.2 Distributivity and modularity

8.3 The theorem of Jordan-Hlder-Dedekind

8.4 The lattice of subspaces of a vector space. Fundamental theorem of projective geometry

8.5 Boolean algebras

8.6 The Mbius function of a partialy ordered set

Preface

The most extensive changes in this edition occur in the segment of the book devoted to commutative algebra, especially in .

In ), we give an improved proof of the existence of a projective resolution of a short exact sequence of modules.

A number of new exercises have been added and some defective ones have been deleted.

Some of the changes we have made were inspired by suggestions made by our colleagues, Walter Feit, George Seligman, and Tsuneo Tamagawa. They, as well as Ronnie Lee, Sidney Porter (a former graduate student), and the Chinese translators of this book, Professors Cao Xi-hua and Wang Jian-pan, pointed out some errors in the first edition which are now corrected. We are indeed grateful for their interest and their important inputs to the new edition. Our heartfelt thanks are due also to F. D. Jacobson, for reading the proofs of this text and especially for updating the index.

January 1989

Nathan Jacobson

Preface to the First Edition

This volume is a text for a second course in algebra that presupposes an introductory course covering the type of material contained in the Introduction and the first three or four chapters of Basic Algebra I. These chapters dealt with the rudiments of set theory, group theory, rings, modules, especially modules over a principal ideal domain, and Galois theory focused on the classical problems of solvability of equations by radicals and constructions with straight-edge and compass.

Basic Algebra II contains a good deal more material than can be covered in a years course. Selection of chapters as well as setting limits within chapters will be essential in designing a realistic program for a year. We briefly indicate several alternatives for such a program: should be included in the course. This, of course, will necessitate thinning down other parts, e.g., homological algebra. There are many other possibilities for a one-year course based on this text.