Paul Parsons - Instant Mathematics

Portable Press

An imprint of Printers Row Publishing Group

10350 Barnes Canyon Road, Suite 100, San Diego, CA 92121

Copyright 2019 Welbeck Non-fiction Limited

All rights reserved. No part of this publication may be reproduced, distributed, or transmitted in any form or by any means, including photocopying, recording, or other electronic or mechanical methods, without the prior written permission of the publisher, except in the case of brief quotations embodied in critical reviews and certain other noncommercial uses permitted by copyright law.

Printers Row Publishing Group is a division of Readerlink Distribution Services, LLC.

Portable Press is a registered trademark of Readerlink Distribution Services, LLC.

Correspondence regarding the content of this book should be addressed to Portable Press, Editorial Department, at the above address. Author and illustration inquiries should be addressed to Welbeck Publishing Group, 2022 Mortimer St, London W1T 3JW.

Portable Press

Publisher: Peter Norton

Associate Publisher: Ana Parker

Editor: Stephanie Romero Gamboa

Senior Product Manager: Kathryn C. Dalby

Produced by Welbeck Non-fiction Limited

eBook ISBN: 978-1-64517-273-4

eBook Edition: August 2020

All illustrations provided by Adam Cunningham and John Ringland, Noun Project & public domain sources.

Its difficult to imagine a world without mathematics. No numbers, no rules of geometry, algebra, or logicno way of quantifying the world.

The first evidence we have for the human understanding of mathematics dates back tens of thousands of years. The Lebombo bone, named after the Lebombo Mountains in Africa where it was discovered by archeologists in the 1970s, is a lower leg bone from a baboon. Its special because, deliberately cut into its length, are twenty-nine notches, believed to be tally marks. The bones owner was keeping count of somethingperhaps hunting kills, the members of an enemy war party, or simply the passing of days.

The bone has been radiocarbon dated to around the year 40,000 BC , making it the worlds oldest mathematical artifact.

Counting is the most primitive example of mathematics. And it was clearly something humans discovered about the world, rather than invented. The number of apples in a basket obeys the rules of arithmetic. So, if I add one apple, the number in the basket increases by one. This has always been the case, whether people were aware of the fact or not.

The same is true of geometry. In around 300 BC , Greek philosopher Euclid wrote his book Elements, in which he set out the fundamental postulates describing the nature of circles, straight lines, and angles. These were rules of logic and inference, formulated to describe the world we live in. They constituted a discovery about that world, not an invention.

And yet, in the past, some very clever people have taken the opposite view: that mathematics is nothing more than a construct of the human mind. The mathematics is not there till we put it there, declared the great twentieth-century English astronomer Sir Arthur Eddington.

Thats certainly true in some cases. For example, imaginary numbers are a construct designed to make tangible the seeming impossibility of taking the square root of a negative number. Choose any ordinary number you like and square it, and the answer will always be positive. So how can you ever take a meaningful square root of something negative? Italian mathematician Rafael Bombelli came up with the answer in the sixteenth century. By denoting 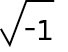by the symbol i, he was able to express arbitrary square roots of negative numbers as a multiple of i, which could be manipulated using the same rules of algebra that governed ordinary numbers. It might sound like a ridiculous thing to do, but, today, imaginary numbers are a mainstay of wave theory, quantum mechanics, and data analysis.

Mathematical proofs are another example, where establishing the validity of a mathematical statement can entail a good deal of creativity on the part of the mathematician. For example, take Fermats Last Theorem: the notion that the equation xn + yn = zn, where x, y, z, and n are all whole numbers, has no solutions for n > 2. It took almost four hundred years to arrive at a watertight proof of this, which was ultimately found only thanks to a substantial feat of ingenuity on the part of the British mathematician Andrew Wiles.

From this perspective, the best mathematicians are architects with a creative vision that is at least the equal of their technical expertise. As Albert Einstein himself put it: Logic will get you from A to B. Imagination will take you everywhere. And the insights gained as pioneering minds charted unknown mathematical territories were inevitably fed back to expand humanitys broader grasp of the subject.

In this book, we present a cheaters guide to the essentials of modern mathematics. Starting from the Lebombo bone and working forward in a (very roughly) chronological order, we lay out the major principles and discoveries, and detail the lives of those who made them.

Following on from the understanding of basic numbers and arithmetic, humans discovered the concepts of geometry, encompassing trigonometry, the calculation of areas and volumes, and Pythagoran theorem relating the three side lengths of a right-angled triangle. In recent centuries, geometry has grown to encompass curved spaces and topologywhich governs how points in a space are connected to one another. A ring doughnut (a surface with a hole through it) has a very different topology to a sphere, for example.

Theres evidence that algebrathe replacement of numerical quantities with symbols, and their subsequent manipulation to solve equationswas known to Babylonian mathematicians as far back as 1800 BC . It was refined further during the European Dark Ages by mathematicians in Asia.

In the seventeenth century, polymaths Isaac Newton and Gottfried Leibniz developed calculus, used for inferring the rate of change of a numerical quantity, and that has since found applications in almost every branch of mathematics, as well as in theoretical physics.

At around the same time, a coterie of European mathematicians (who, it must be said, were also fond of supplementing their income by gambling) laid down the foundations of probability theory: the mathematical laws governing the likelihood of random events. From this grew statistics: the art of extracting information from data in the presence of random noiseconverting messy, real-world observations into hard-and-fast knowledge, against which scientific theories can be tested and refined.

Mathematical reasoning also gave rise to computing. The number system were most used to dealing with is base 10, built from the numerals 09. But in the second century BC , the Indian mathematician Pingala was the first to contemplate binary, or base 2 numbers, constructed from just 0 and 1. Binary later became the language of information. And in the nineteenth century, the desire to process this information in an automated way led to the invention of mechanical computers. These were followed by the room-sized behemoths used for codebreaking during the Second World War, and most recently by those tiny yet powerful information processors that go everywhere with us inside our mobile phones.