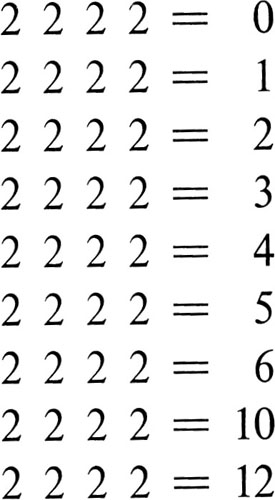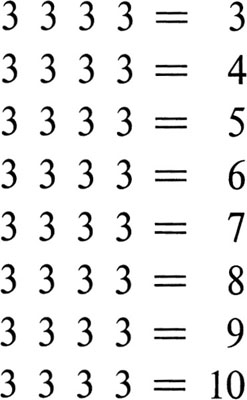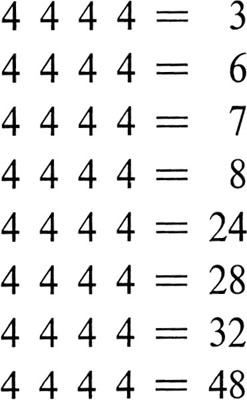Pierre Berloquin - 100 Numerical Games

Copyright

Copyright 1976 by Pierre Berloquin

All rights reserved.

Bibliographical Note

This Dover edition, first published in 2015, is an unabridged republication of the work originally published in 1976 by Charles Scribners Sons, New York.

International Standard Book Number

eISBN-13: 978-0-486-80569-6

Manufactured in the United States by Courier Corporation
78958601 2015

www.doverpublications.com

Pierre Berloquin, who put together this stimulating and delightful collection of mind benders, is a clever young Frenchman who was born in 1939 in Tours and graduated in 1962 from the cole Nationale Suprieure des Mines in Paris. His training as an operations research engineer gave him an excellent background in mathematics and logical thinking.

But Berloquin was more interested in writing than in working on operations research problems. After two years with a Paris advertising agency, he decided to try his luck at freelance writing and this is how he has earned his living since. In 1964 he began his popular column on Games and Paradoxes in the magazine Science et Vie (Science and Life). Another column, From a Logical Point of View, appears twice monthly in The World of Science, a supplement of the Paris newspaper Le Monde. Occasionally he contributes to other French magazines. One of his favorite avocations is leading groups of crativit a French cocktail of brainstorming, synectics, and encounter therapy, for the discovery of new ideas and the solution of problemsa logical extension of his interest in puzzles.

Berloquins published books are Le Livre des Jeux (card and board games), Le Jeu de Tarot (tarot card game), Testez Votre Intelligence (intelligence tests), 100 Grandes Russites (solitaire games), Un Souvenir dEnfance dEvariste Galois (Memoir of the Childhood of Evariste Galois) and 100 Jeux de Cartes Classiques (card games); he is coauthor of Voulez-Vous Jouer avec Nous (Come Play with Us) and Le Livre des Divertissements (party games).

This volume is Berloquins own translation into English of one of his four paperback collections of brainteasers which have been enormously popular in France and Italy since they were published in Paris in 1973. This one is concerned only with numerical puzzles. The other three contain geometric, logical, and alphabetical problems. Denis Dugas, the graphic artist who illustrated all four books, is one of the authors old friends.

The puzzles in this collection have been carefully selected or designed (many are original with the author or artist) so that none will be too difficult for the average reader who is not a mathematician to solve, and at the same time not be too easy. They are all crisply, clearly given, accurately answered at the back of the book, and great fun to work on whether you crack them or not.

At present, Berloquin is living in Neuilly, a Paris suburb, with his wife, Annie, and their two children.

Martin Gardner

Timothy spent all his money in five stores. In each store, he spent $ 1 more than half of what he had when he came in.

How much did Timothy have when he entered the first store?

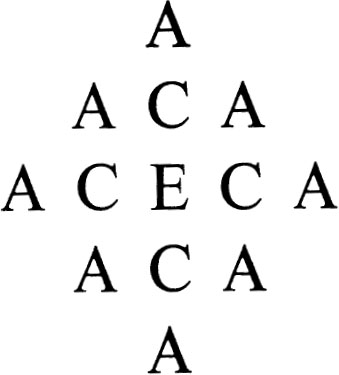
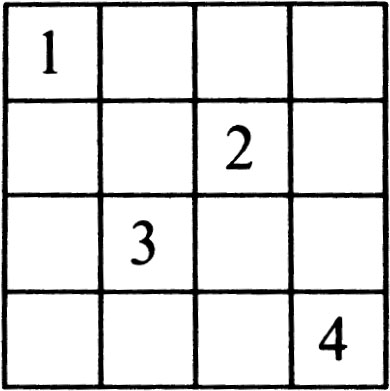
How many ways can you read ACE off the diagram? You can move horizontally, vertically, or diagonally.

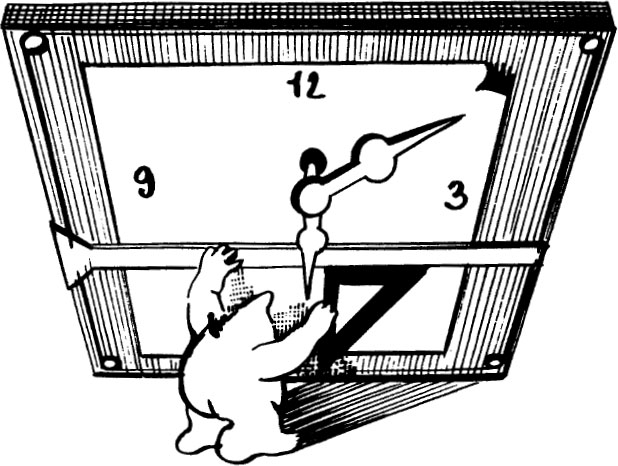
Let us follow the hour hand and minute hand of a clock for 24 hours.

How many times do they form a right angle?

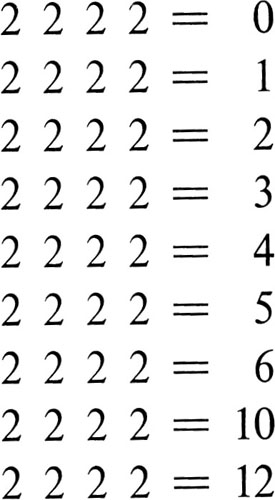
Add arithmetical symbols between the 2s to make every equation true. You may use plus, minus, times, and divide symbols, as well as parentheses and brackets for grouping.

Two towns are linked by a railroad. Every hour on the hour a train leaves each town for the other town. The trains all go at the same speed and every trip from one town to the other takes 5 hours.

How many trains are met by one train during one trip?

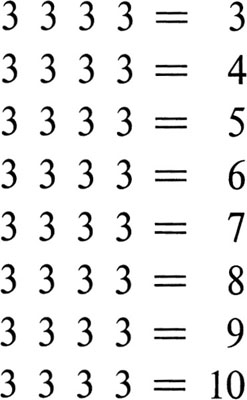
Add arithmetical symbols between the 3s to make every equation true.

A clock strikes every houronce at 1:00, twice at 2:00, and so on.

The clock takes 6 seconds to strike 5:00 and 12 seconds to strike 9:00. The time needed to strike 1:00 is negligible.

How long does the clock need for all its striking in 24 hours?

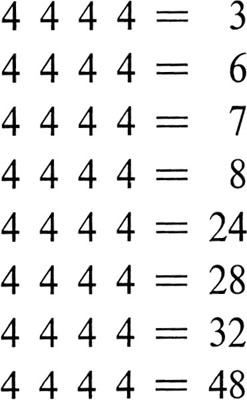
Add arithmetical symbols between the 4s to make every equation true.

Timothy goes to a fountain which delivers an unlimited amount of water. He brings two empty containers, one of 7 liters, the other of 11 liters.

How many operations does he need to fill one of the containers with exactly 6 liters of water? (In these container games, in each operation one container must be completely filled or completely emptied.)

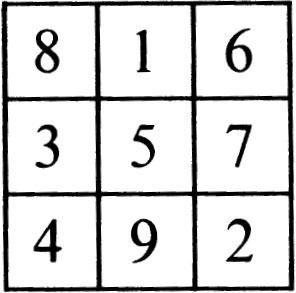
This is a magic square. On each horizontal, each vertical, and each of the two diagonals the numbers always add up to 15.

Can you complete the next square with the integers from 5 through 16 to make it magic? On each horizontal, each vertical, and each of the two diagonals the sum of the numbers is to be 34.

(Hint: The two unfilled central squares must add up to 29; the two unfilled corner squares must also add up to 29.)